Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-28T18:20:11.270Z Has data issue: false hasContentIssue false
  • English
  • Français

Measurements of buoyancy flux in a stratified turbulent flow

Published online by Cambridge University Press:  27 December 2018

Diana Petrolo Open the ORCID record for Diana Petrolo [Opens in a new window]  and
Andrew W. Woods
Diana Petrolo
Affiliation:
BP Institute for Multiphase Flow, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK Dipartimento di Ingegneria e Architettura (DIA), Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124 Parma, I, Italy
Andrew W. Woods*
Affiliation:
BP Institute for Multiphase Flow, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present new experimental data on the controls on the buoyancy flux in a stratified turbulent flow. The inner cylinder of an annulus of fluid with vertical axis is rotated to produce a turbulent flow field with Reynolds numbers of up to 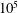$10^{5}$, while a flux of saline fluid is supplied to the base of the tank, and an equal flux of fresh fluid is supplied to the top of the tank. In addition, fluid is vented from the base and the top of the tank with the same volume fluxes as the supply. The steady-state vertical flux of salt is explored. When the salt flux supplied to the base of the tank is very small, the tank becomes nearly well-mixed, and the vertical salt flux is approximately equal to one-half the source flux. As the source salt flux increases, a weak stable salinity gradient develops across the tank, and the vertical salt flux increases. As the source flux continues to increase, eventually the vertical salt flux reaches a maximum, and further increases in the source salt flux can lead to an increase in the vertical salinity gradient but not the vertical flux. We interpret the transition in the vertical buoyancy flux as representing a change from a source-limited regime, where the buoyancy flux and buoyancy frequency, $N$, are related, to a mixing-limited regime, in which the buoyancy flux is independent of 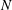$N$. In the mixing-limited regime, the effective eddy diffusivity is proportional to 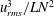$u_{rms}^{3}/LN^{2}$ while in the source-limited regime, the eddy diffusivity is approximately proportional to 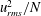$u_{rms}^{2}/N$, where 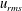$u_{rms}$ and 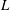$L$ are the characteristic turbulence speed and length scale. This transition may have implications for the balance between upwelling and diapycnal mixing in the ocean, if the intensity of the turbulence varies in space or the flux of deep water varies in time.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Ocean processes
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 861 , 25 February 2019 , R2
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Balmforth, N. J., Smith, S. G. L. & Young, W. R. 1998 Dynamics of interfaces and layers in a stratified turbulent fluid. J. Fluid Mech. 355, 329–358.Google Scholar
Barenblatt, G. I., Bertsch, M., Dal Passo, R., Prostokishin, V. M. & Ughi, M. 1993 A mathematical model of turbulent heat and mass transfer in stably stratified shear flow. J. Fluid Mech. 253, 341–358.Google Scholar
Billant, P. & Chomaz, J. M. 2001 Self-similarity of strongly stratified inviscid flows. Phys. Fluids 13 (6), 1645–1651.Google Scholar
Bryan, F. 1987 Parameter sensitivity of primitive equation ocean general circulation models. J. Phys. Oceanogr. 17 (7), 970–985.Google Scholar
Falder, M., White, N. J. & Caulfield, C. P. 2016 Seismic imaging of rapid onset of stratified turbulence in the south Atlantic ocean. J. Phys. Oceanogr. 46 (4), 1023–1044.Google Scholar
Garrett, C. & Munk, W. 1975 Space-time scales of internal waves: a progress report. J. Geophys. Res. 80 (3), 291–297.Google Scholar
Holford, J. M. & Linden, P. F. 1999 Turbulent mixing in a stratified fluid. Dyn. Atmos. Oceans 30 (2–4), 173–198.Google Scholar
Kato, H. & Phillips, O. M. 1969 On the penetration of a turbulent layer into stratified fluid. J. Fluid Mech. 37 (4), 643–655.Google Scholar
Maffioli, A., Brethouwer, G. & Lindborg, E. 2016 Mixing efficiency in stratified turbulence. J. Fluid Mech. 794, R3.Google Scholar
Munk, W. 1966 Abyssal recipes. In Deep Sea Research and Oceanographic Abstracts, vol. 13, pp. 707–730. Elsevier.Google Scholar
Munk, W. & Wunsch, C. 1998 Abyssal recipes ii: energetics of tidal and wind mixing. Deep Sea Res. I 45 (12), 1977–2010.Google Scholar
Oglethorpe, R. L. F., Caulfield, C. P. & Woods, A. W. 2013 Spontaneous layering in stratified turbulent Taylor–Couette flow. J. Fluid Mech. 721, R3.Google Scholar
Osborn, T. R. 1980 Estimates of the local rate of vertical diffusion from dissipation measurements. J. Phys. Oceanogr. 10 (1), 83–89.Google Scholar
Rahmstorf, S. 1994 Rapid climate transitions in a coupled ocean–atmosphere model. Nature 372 (6501), 82–85.Google Scholar
Rahmstorf, S. 2006 Thermohaline ocean circulation. In Encyclopedia of Quaternary Sciences, vol. 5. Postdam Institute for Climate Impact Research.Google Scholar
Riley, J. J. & Lindborg, E. 2008 Stratified turbulence: a possible interpretation of some geophysical turbulence measurements. J. Atmos. Sci. 65 (7), 2416–2424.Google Scholar
Salehipour, H., Peltier, W. R. & Mashayek, A. 2015 Turbulent diapycnal mixing in stratified shear flows: the influence of Prandtl number on mixing efficiency and transition at high Reynolds number. J. Fluid Mech. 773, 178–223.Google Scholar
Sheen, K. L., White, N. J. & Hobbs, R. W. 2009 Estimating mixing rates from seismic images of oceanic structure. Geophys. Res. Lett. 36 (24), L00D04.Google Scholar
Thorpe, S. A. 2005 The Turbulent Ocean. Cambridge University Press.Google Scholar
Waterhouse, A. F., MacKinnon, J. A., Nash, J. D., Alford, M. H., Kunze, E., Simmons, H. L., Polzin, K. L., St. Laurent, L. C., Sun, O. M., Pinkel, R., Talley, L. D., Whalen, C. B., Huussen, T. N., Carter, G. S., Fer, I., Waterman, S., Naveira Garabato, A. C., Sanford, T. B. & Lee, C. M. 2014 Global patterns of diapycnal mixing from measurements of the turbulent dissipation rate. J. Phys. Oceanogr. 44 (7), 1854–1872.Google Scholar
Woods, A. W., Caulfield, C. P., Landel, J. R. & Kuesters, A. 2010 Non-invasive turbulent mixing across a density interface in a turbulent Taylor–Couette flow. J. Fluid Mech. 663, 347–357.Google Scholar
Wunsch, C. 2000 Oceanography: moon, tides and climate. Nature 405 (6788), 743–744.Google Scholar