Article contents
Magnetohydrodynamic drift equations: from Langmuir circulations to magnetohydrodynamic dynamo?
Published online by Cambridge University Press: 23 March 2012
Abstract
We derive the closed system of averaged magnetohydrodynamic (MHD) equations for general oscillating flows. The used small parameter of our asymptotic theory is the dimensionless inverse frequency, and the leading term for a velocity field is chosen to be purely oscillating. The employed mathematical approach combines the two-timing method and the notion of a distinguished limit. The properties of commutators are used to simplify calculations. The derived averaged equations are similar to the original MHD equations, but surprisingly (instead of the commonly expected Reynolds stresses) a drift velocity plays a part of an additional advection velocity. In the special case of a vanishing magnetic field 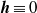 , the averaged equations produce the Craik–Leibovich equations for Langmuir circulations (which can be called ‘vortex dynamo’). We suggest that, since the mathematical structure of the full averaged equations for
, the averaged equations produce the Craik–Leibovich equations for Langmuir circulations (which can be called ‘vortex dynamo’). We suggest that, since the mathematical structure of the full averaged equations for 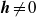 is similar to those for
is similar to those for 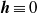 , these full equations could lead to a possible mechanism of MHD dynamo, such as the generation of the magnetic field of the Earth.
, these full equations could lead to a possible mechanism of MHD dynamo, such as the generation of the magnetic field of the Earth.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012
References
- 10
- Cited by


