Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T00:16:50.808Z Has data issue: false hasContentIssue false
  • English
  • Français

Mach reflection promoted by an upstream shock wave

Published online by Cambridge University Press:  02 October 2020

Xiao-Ke Guan ,
Chen-Yuan Bai ,
Jing Lin  and
Zi-Niu Wu Open the ORCID record for Zi-Niu Wu [Opens in a new window]
Xiao-Ke Guan
Affiliation:
Department of Engineering Mechanics, Tsinghua University, Beijing100084, PR China
Chen-Yuan Bai
Affiliation:
Ministry of Education Key Laboratory of Fluid Mechanics, Beijing University of Aeronautics and Astronautics, Beijing100191, PR China
Jing Lin
Affiliation:
Department of Engineering Mechanics, Tsinghua University, Beijing100084, PR China
Zi-Niu Wu*
Affiliation:
Department of Engineering Mechanics, Tsinghua University, Beijing100084, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

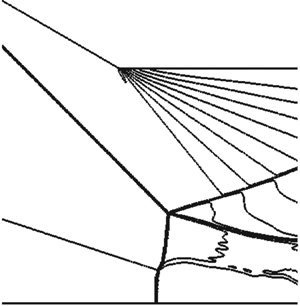
Mach reflection subjected to the influence of an upstream shock wave from the same side is studied here. This situation occurs when two incident shock waves induced by a double wedge reflect at the same point of the reflecting surface and when the downstream incident shock wave is stronger than the upstream one. A shock polar analysis is used to show that this configuration produces an inverted Mach stem and a type IV shock interference between the Mach stem and the upstream shock wave. This shock interference produces a jet that divides the flow stream downstream of the Mach stem into two ducts with different sonic throats, thus complicating the mechanism by which the Mach stem size is determined. A transition analysis shows that the Mach reflection of the downstream shock wave is promoted by the upstream one. Computational fluid dynamics is used to assess the flow pattern anticipated by shock polar analysis and demonstrates how the heights of Mach stem and jet depend on the inflow Mach number and wedge turning angle.

JFM classification

Compressible Flows: Shock waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 903 , 25 November 2020 , A44
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bai, C. Y. & Wu, Z. N. 2017 Size and shape of shock waves and slipline for Mach reflection in steady flow. J. Fluid Mech. 818, 116–140.CrossRefGoogle Scholar
Ben-Dor, G. 2007 Shock Wave Reflection Phenomena. Springer.Google Scholar
Ben-Dor, G., Elperin, T., Li, H. & Vasiliev, E. 1999 The influence of the downstream pressure on the shock wave reflection phenomenon in steady flows. J. Fluid Mech. 386, 213–232.CrossRefGoogle Scholar
Ben-Dor, G., Igra, O. & Elperin, T. 2001 Handbook of Shock Waves, vols. I–III. Academic.Google Scholar
Ben-Dor, G., Ivanov, M., Vasilev, E. I. & Elperin, T. 2002 Hysteresis processes in the regular reflection 2: mach reflection transition in steady flows. Prog. Aerosp. Sci. 38, 347–387.CrossRefGoogle Scholar
Chen, Z. J., Bai, C. Y. & Wu, Z. N. 2020 Mach reflection in steady supersonic flow considering wedge boundary-layer correction. Chin. J. Aeronaut. 33, 465–475.CrossRefGoogle Scholar
Courant, R. & Friedrichs, K. O. 1948 Supersonic Flow and Shock Waves. Wiley-Interscience.Google Scholar
Edney, B. 1968 Anomalous heat transfer and pressure distributions on blunt bodies at hypersonic speeds in the presence of an impinging shock. FFA Rep. 115 Flygtekniska Forsoksanstalten, Stockholm.CrossRefGoogle Scholar
Gaitonde, D. & Shang, J. S. 1995 On the structure of an unsteady type IV interaction at Mach 8. Comput. Fluids 24, 469–485.CrossRefGoogle Scholar
Gao, B. & Wu, Z. N. 2010 A study of the flow structure for Mach reflection in steady supersonic flow. J. Fluid Mech. 656, 29–50.CrossRefGoogle Scholar
Grasso, F., Purpura, C., Chanetz, B. & Delery, J. 2003 Type III and type IV shock/shock interferences: theoretical and experimental aspects. Aerosp. Sci.Technol. 7 (2), 93–106.CrossRefGoogle Scholar
Guan, X. K., Bai, C. Y. & Wu, Z. N. 2018 Steady Mach reflection with two incident shock waves. J. Fluid Mech. 855, 882–909.CrossRefGoogle Scholar
Guan, X. K., Bai, C. Y. & Wu, Z. N. 2020 Double solution and influence of secondary waves on transition criteria for shock interference in pre-Mach reflection with two incident shock waves. J. Fluid Mech. 887, A22.CrossRefGoogle Scholar
Hekiri, H. & Emanuel, G. 2015 Structure and morphology of a triple point. Phys. Fluids 27, 056102.CrossRefGoogle Scholar
Hillier, R. 2007 Shock-wave/expansion-wave interactions and the transition between regular and Mach reflection. J. Fluid Mech. 575 (575), 399–424.CrossRefGoogle Scholar
Hornung, H. G. 1986 Regular and Mach reflections of shock waves. Annu. Rev. Fluid Mech. 18, 33–58.CrossRefGoogle Scholar
Hornung, H. G. 2014 Mach reflection in steady flow. I. Mikhail Ivanov's contributions, II. Caltech stability experiments. In 29th International Symposium on Rarefied Gas Dynamics, AIP Conference Proceedings, vol. 1628, pp. 1384–1393. AIP Publishing.CrossRefGoogle Scholar
Hornung, H. G. & Robinson, M. L. 1982 Transition from regular to Mach reflection of shock waves. Part 2. The steady-flow criterion. J. Fluid Mech. 123, 155–164.CrossRefGoogle Scholar
Hu, Z. M., Gao, Y. L., Myong, R. S., Dou, H. S. & Khoo, B. C. 2010 Geometric criterion for transition in hypersonic double-wedge flows. Phys. Fluids 22, 016101.CrossRefGoogle Scholar
Kawamura, R. & Saito, H. 1956 Reflection of shock waves—1. Pseudo-stationary cases. J. Phys. Soc. Japan 11, 584–592.CrossRefGoogle Scholar
Keyes, J. W. & Hains, F. D. 1973 Analytical and experimental studies of shock interference heating in hypersonic flows. NASA TND-7139, https://babel.hathitrust.org/cgi/pt?id=uiug.30112106885186&view=1up&seq=1.Google Scholar
Khatt, A. & Jagadeesh, G. 2018 Hypersonic shock tunnel studies of Edney type III and IV shock interactions. Aerosp. Sci. Technol. 72, 335–352.CrossRefGoogle Scholar
Li, H. & Ben-Dor, G. 1997 A parametric study of Mach reflection in steady flows. J. Fluid Mech. 341, 101–125.CrossRefGoogle Scholar
Li, H., Chpoun, A. & Ben-Dor, G. 1999 Analytical and experimental investigations of the reflection of asymmetric shock waves in steady flows. J. Fluid Mech. 390, 25–43.CrossRefGoogle Scholar
Li, J., Zhu, Y. J. & Luo, X. S. 2014 On type VI–V transition in hypersonic double-wedge flows with thermo-chemical nonequilibrium effects. Phys. Fluids 26, 086104.CrossRefGoogle Scholar
Lin, J., Bai, C. Y. & Wu, Z. N. 2019 Study of asymmetrical shock wave reflection in steady supersonic flow. J. Fluid Mech. 864, 848–875.CrossRefGoogle Scholar
Mouton, C. A. 2007 Transition between regular reflection and Mach reflection in the dual-solution domain. PhD dissertation, California Institute of Technology.Google Scholar
Mouton, C. A. & Hornung, H. G. 2007 Mach stem height and growth rate predictions. AIAA J. 45, 1977–1987.CrossRefGoogle Scholar
Roe, P. L. 1986 Characteristic based schemes for the Euler equations. Annu. Rev. Fluid Mech. 18, 337–365.CrossRefGoogle Scholar
Roye, L., Henderson, F. & Menikoff, R. 1998 Triple-shock entropy theorem and its consequences. J. Fluid Mech. 366, 179–210.Google Scholar
Schmisseur, J. D. & Gaitonde, D. V. 2011 Numerical simulation of Mach reflection in steady flows. Shock Waves 21, 499–509.CrossRefGoogle Scholar
Shah, S., Martinez, R., Fernandez, N. & Mourtos, N. 2008 Double wedge shockwave interaction flow characterization. In Thermal and Fluid Analysis Workshop, TFAWS-08-1033, https://tfaws.nasa.gov/TFAWS08/Proceedings/Papers/TFAWS-08-1033.pdf.Google Scholar
Sudani, N., Sato, M., Karasawa, T., Noda, J., Tate, A. & Watanabe, M. 2002 Irregular effects on the transition from regular to Mach reflection of shock waves in wind tunnel flows. J. Fluid Mech. 459, 167–185.CrossRefGoogle Scholar
Xiong, W. T., Zhu, Y. J. & Luo, X. S. 2016 On transition of type V interaction in double-wedge flow with non-equilibrium effects. Theor. Appl. Mech. Lett. 6, 282–285.CrossRefGoogle Scholar
Yao, Y., Li, S. G. & Wu, Z. N. 2013 Shock reflection in the presence of an upstream expansion wave and a downstream shock wave. J. Fluid Mech. 735, 61–90.CrossRefGoogle Scholar