Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-24T16:24:48.690Z Has data issue: false hasContentIssue false
  • English
  • Français

The linear instability of the stratified plane Couette flow

Published online by Cambridge University Press:  23 August 2018

Giulio Facchini ,
Benjamin Favier Open the ORCID record for Benjamin Favier [Opens in a new window] ,
Patrice Le Gal ,
Meng Wang Open the ORCID record for Meng Wang [Opens in a new window]  and
Michael Le Bars
Giulio Facchini*
Affiliation:
Aix Marseille Univ., CNRS, Centrale Marseille, IRPHE UMR 7342, 49 rue F. Joliot Curie, Marseille, 13013, France
Benjamin Favier
Affiliation:
Aix Marseille Univ., CNRS, Centrale Marseille, IRPHE UMR 7342, 49 rue F. Joliot Curie, Marseille, 13013, France
Patrice Le Gal
Affiliation:
Aix Marseille Univ., CNRS, Centrale Marseille, IRPHE UMR 7342, 49 rue F. Joliot Curie, Marseille, 13013, France
Meng Wang
Affiliation:
Department of Mechanical Engineering, University of California Berkeley, 6121 Etcheverry Hall, Berkeley, CA 94720-1740, USA
Michael Le Bars
Affiliation:
Aix Marseille Univ., CNRS, Centrale Marseille, IRPHE UMR 7342, 49 rue F. Joliot Curie, Marseille, 13013, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present the stability analysis of a plane Couette flow which is stably stratified in the vertical direction orthogonal to the horizontal shear. Interest in such a flow comes from geophysical and astrophysical applications where background shear and vertical stable stratification commonly coexist. We perform the linear stability analysis of the flow in a domain which is periodic in the streamwise and vertical directions and confined in the cross-stream direction. The stability diagram is constructed as a function of the Reynolds number $Re$ and the Froude number $Fr$, which compares the importance of shear and stratification. We find that the flow becomes unstable when shear and stratification are of the same order (i.e. 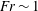$Fr\sim 1$) and above a moderate value of the Reynolds number 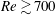$Re\gtrsim 700$. The instability results from a wave resonance mechanism already known in the context of channel flows – for instance, unstratified plane Couette flow in the shallow-water approximation. The result is confirmed by fully nonlinear direct numerical simulations and, to the best of our knowledge, constitutes the first evidence of linear instability in a vertically stratified plane Couette flow. We also report the study of a laboratory flow generated by a transparent belt entrained by two vertical cylinders and immersed in a tank filled with salty water, linearly stratified in density. We observe the emergence of a robust spatio-temporal pattern close to the threshold values of 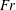$Fr$ and 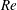$Re$ indicated by linear analysis, and explore the accessible part of the stability diagram. With the support of numerical simulations we conclude that the observed pattern is a signature of the same instability predicted by the linear theory, although slightly modified due to streamwise confinement.

JFM classification

Waves/Free-surface Flows: Channel flow Instability: Instability Geophysical and Geological Flows: Internal waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 853 , 25 October 2018 , pp. 205 - 234
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acheson, D. J. 1990 Elementary Fluid Dynamics. Oxford University Press.Google Scholar
Arratia, C.2011 Non-modal instability mechanisms in stratified and homogeneous shear flow. Theses, Ecole Polytechnique X.Google Scholar
Baines, P. G. & Mitsudera, H. 1994 On the mechanism of shear flow instabilities. J. Fluid Mech. 276, 327–342.Google Scholar
Bakas, N. A. & Farrell, B. F. 2009 Gravity waves in a horizontal shear flow. Part ii: interaction between gravity waves and potential vorticity perturbations. J. Phys. Oceanogr. 39 (3), 497–511.Google Scholar
Barkley, D. & Tuckerman, L. S. 2005 Computational study of turbulent laminar patterns in Couette flow. Phys. Rev. Lett. 94, 014502.Google Scholar
Bayly, B. J., Orszag, A. & Herbert, T. 1988 Instability mechanisms in shear-flow transition. Annu. Rev. Fluid Mech. 20 (1), 359–391.Google Scholar
Boulanger, N., Meunier, P. & Le Dizès, S. 2008 Tilt-induced instability of a stratified vortex. J. Fluid Mech. 596, 1–20.Google Scholar
Candelier, J., Le Dizès, S. & Millet, C. 2011 Shear instability in a stratified fluid when shear and stratification are not aligned. J. Fluid Mech. 685, 191–201.Google Scholar
Caulfield, C.-C. P. 1994 Multiple linear instability of layered stratified shear flow. J. Fluid Mech. 258, 255–285.Google Scholar
Chen, J.2016 Stabilité d’un écoulement stratifié sur une paroi et dans un canal. PhD thesis, École Centrale Marseille.Google Scholar
Chen, J., Bai, Y. & Le Dizès, S. 2016 Instability of a boundary layer flow on a vertical wall in a stably stratified fluid. J. Fluid Mech. 795, 262–277.Google Scholar
Davey, A. 1973 On the stability of plane Couette flow to infinitesimal disturbances. J. Fluid Mech. 57 (2), 369–380.Google Scholar
Deloncle, A., Chomaz, J.-M. & Billant, P. 2007 Three-dimensional stability of a horizontally sheared flow in a stably stratified fluid. J. Fluid Mech. 570, 297–305.Google Scholar
Dengler, M. & Quadfasel, D. 2002 Equatorial deep jets and abyssal mixing in the Indian Ocean. J. Phys. Oceanogr. 32 (4), 1165–1180.Google Scholar
d’Orgeville, M., Hua, B. L., Schopp, R. & Bunge, L. 2004 Extended deep equatorial layering as a possible imprint of inertial instability. Geophys. Res. Lett. 31 (22), l22303.Google Scholar
Dubrulle, B., Marié, L., Normand, C., Richard, D., Hersant, F. & Zahn, J.-P. 2005 An hydrodynamic shear instability in stratified disks. J. Astron. Astrophys. 429, 1–13.Google Scholar
Dunkerton, T. J. 1981 On the inertial stability of the equatorial middle atmosphere. J. Atmos. Sci. 38 (11), 2354–2364.Google Scholar
Fischer, P. F. 1997 An overlapping Schwarz method for spectral element solution of the incompressible Navier–Stokes equations. J. Comput. Phys. 133 (1), 84–101.Google Scholar
Fischer, P. F., Loth, F., Lee, S. E., Lee, S.-W., Smith, D. S. & Bassiouny, H. S. 2007 Simulation of high-Reynolds number vascular flows. Comput. Meth. Appl. Mech. Engng 196 (31), 3049–3060.Google Scholar
Heisenberg, W. 1924 Über Stabilität und Turbulenz von Flüssigkeitsströmen. Ann. Phys. 379, 577–627.Google Scholar
Helmholtz, H. L. F. v. 1868 XLIII. On discontinuous movements of fluids. Phil. Mag. 36 (244), 337–346.Google Scholar
Holmboe, J. 1962 On the behaviour of symmetric waves in stratified shear layers. Geofys. Publ. 24, 67–113.Google Scholar
Howard, L. N. 1961 Note on a paper of John W. Miles. J. Fluid Mech. 10 (4), 509–512.Google Scholar
Ibanez, R., Swinney, H. L. & Rodenborn, B. 2016 Observations of the stratorotational instability in rotating concentric cylinders. Phys. Rev. Fluids 1, 053601.Google Scholar
Lord Kelvin 1871 XLVI. Hydrokinetic solutions and observations. Phil. Mag. 42 (281), 362–377.Google Scholar
Kushner, P. J., McIntyre, M. E. & Shepherd, T. G. 1998 Coupled Kelvin-wave and mirage-wave instabilities in semigeostrophic dynamics. J. Phys. Oceanogr. 28 (3), 513–518.Google Scholar
Le Bars, M. & Le Gal, P. 2007 Experimental analysis of the stratorotational instability in a cylindrical Couette flow. Phys. Rev. Lett. 99, 064502.Google Scholar
Lin, C. 1966 The Theory of Hydrodynamic Stability. Cambridge University Press, xi, 155 pp.Google Scholar
Lucas, D. & Caulfield, C. P. 2017 Irreversible mixing by unstable periodic orbits in buoyancy dominated stratified turbulence. J. Fluid Mech. 832, R1.Google Scholar
Lucas, D., Caulfield, C. P. & Kerswell, R. R. 2017 Layer formation in horizontally forced stratified turbulence: connecting exact coherent structures to linear instabilities. J. Fluid Mech. 832, 409–437.Google Scholar
Marcus, P. S., Pei, S., Jiang, C.-H. & Hassanzadeh, P. 2013 Three-dimensional vortices generated by self-replication in stably stratified rotating shear flows. Phys. Rev. Lett. 111, 084501.Google Scholar
Meunier, P. & Leweke, T. 2003 Analysis and treatment of errors due to high velocity gradients in particle image velocimetry. Exp. Fluids 35 (5), 408–421.Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10 (4), 496–508.Google Scholar
Molemaker, M. J., McWilliams, J. C. & Yavneh, Irad 2001 Instability and equilibration of centrifugally stable stratified Taylor–Couette flow. Phys. Rev. Lett. 86, 5270–5273.Google Scholar
Oglethorpe, R. L. F., Caulfield, C. P. & Woods, A. W. 2013 Spontaneous layering in stratified turbulent Taylor–Couette flow. J. Fluid Mech. 721, R3.Google Scholar
Orr, W. M’F. 1907 The stability or instability of the steady motions of a perfect liquid and of a viscous liquid. Part I: a perfect liquid. Proc. R. Irish Acad. A 27, 9–68.Google Scholar
Orszag, S. A. 1971 Accurate solution of the Orr–Sommerfeld stability equation. J. Fluid Mech. 50 (4), 689–703.Google Scholar
Oster, G. 1965 Density gradients. Sci. Am. 213, 70–76.Google Scholar
Park, J. & Billant, P. 2013 The stably stratified Taylor–Couette flow is always unstable except for solid-body rotation. J. Fluid Mech. 725, 262–280.Google Scholar
Lord Rayleigh 1879 On the stability, or instability, of certain fluid motions. Proc. Lond. Math. Soc. s1–11 (1), 57–72.Google Scholar
Lord Rayleigh 1917 On the dynamics of revolving fluids. Proc. R. Soc. Lond. A 93 (648), 148–154.Google Scholar
Reynolds, O. 1883 An experimental investigation of the circumstances which determine whether the motion of water shall be direct or sinuous, and of the law of resistance in parallel channels. Phil. Trans. R. Soc. Lond. 174, 935–982.Google Scholar
Romanov, V. A. 1973 Stability of plane-parallel Couette flow. Funct. Anal. Applics. 7 (2), 137–146.Google Scholar
Satomura, T. 1981 An investigation of shear instability in a shallow water. J. Met. Soc. Japan. II 59 (1), 148–167.Google Scholar
Schlichting, H. 1933 Zur Enstehung der Turbulenz bei der Plattenstrmung. Nachr. Ges. Wiss. Göttingen 1933, 181–208.Google Scholar
Taylor, G. I. 1931 Effect of variation in density on the stability of superposed streams of fluid. Proc. R. Soc. Lond. A 132 (820), 499–523.Google Scholar
Thorpe, S. A. 2016 Layers and internal waves in uniformly stratified fluids stirred by vertical grids. J. Fluid Mech. 793, 380–413.Google Scholar
Vanneste, J. & Yavneh, I. 2007 Unbalanced instabilities of rapidly rotating stratified shear flows. J. Fluid Mech. 584, 373–396.Google Scholar
Woods, A. W., Caulfield, C. P., Landel, J. R. & Kuesters, A. 2010 Non-invasive turbulent mixing across a density interface in a turbulent Taylor–Couette flow. J. Fluid Mech. 663, 347–357.Google Scholar
Yavneh, I., McWilliams, J. C. & Molemaker, M. J. 2001 Non-axisymmetric instability of centrifugally stable stratified Taylor–Couette flow. J. Fluid Mech. 448, 1–21.Google Scholar