Published online by Cambridge University Press: 23 August 2012
Flow instability in the non-orthogonal swept attachment-line boundary layer is addressed in a linear analysis framework via solution of the pertinent global (BiGlobal) partial differential equation (PDE)-based eigenvalue problem. Subsequently, a simple extension of the extended Görtler–Hämmerlin ordinary differential equation (ODE)-based polynomial model proposed by Theofilis et al. (2003) for orthogonal flow, which includes previous models as special cases and recovers global instability analysis results, is presented for non-orthogonal flow. Direct numerical simulations have been used to verify the analysis results and unravel the limits of validity of the basic flow model analysed. The effect of the angle of attack, 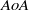 , on the critical conditions of the non-orthogonal problem has been documented; an increase of the angle of attack, from
, on the critical conditions of the non-orthogonal problem has been documented; an increase of the angle of attack, from 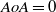 (orthogonal flow) up to values close to
(orthogonal flow) up to values close to 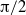 which make the assumptions under which the basic flow is derived questionable, is found to systematically destabilize the flow. The critical conditions of non-orthogonal flows at
which make the assumptions under which the basic flow is derived questionable, is found to systematically destabilize the flow. The critical conditions of non-orthogonal flows at 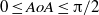 are shown to be recoverable from those of orthogonal flow, via a simple algebraic transformation involving
are shown to be recoverable from those of orthogonal flow, via a simple algebraic transformation involving 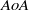 .
.