Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T08:27:53.931Z Has data issue: false hasContentIssue false
  • English
  • Français

Laboratory-scale investigation of a periodically forced stratified basin with inclined endwalls

Published online by Cambridge University Press:  02 December 2021

Sara Marković Open the ORCID record for Sara Marković [Opens in a new window]  and
Vincenzo Armenio Open the ORCID record for Vincenzo Armenio [Opens in a new window]
Sara Marković*
Affiliation:
Dipartimento di Ingegneria e Architettura, University of Trieste, Piazzale Europa 1, 34127Trieste, Italy
Vincenzo Armenio
Affiliation:
Dipartimento di Ingegneria e Architettura, University of Trieste, Piazzale Europa 1, 34127Trieste, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

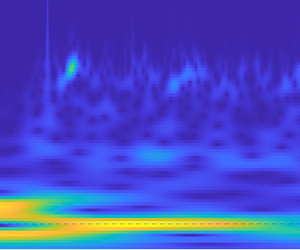
We present results of numerical simulations of a stratified reservoir with a three-layer stratification, subject to an oscillating surface shear stress. We investigate the effect of sloped endwalls on mixing and internal wave adjustment to forcing within the basin, for three different periods of forcing. The simulations are carried out at a laboratory scale, using large-eddy simulation. We solve the three-dimensional Navier–Stokes equations under the Boussinesq approximation using a second-order-accurate finite-volume solver. The model was validated by reproducing experimental results for the response of a reservoir to surface shear stress and resonant frequencies of internal waves. We find interesting combinations of wave modes and mixing under variation of the forcing frequencies and of the inclination of the endwalls. When the frequency of the forcing is close to the fundamental mode-one wave frequency, a resonant internal seiche occurs and the response is characterized by the first vertical mode. For forcing periods twice and three times the fundamental period, the dominant response is in terms of the second vertical mode. Adjustment to forcing via the second vertical mode is accompanied by the cancellation of the fundamental wave and energy transfer to higher-frequency waves. The study shows that the slope of the endwalls dramatically affects the location of mixing, which has a feedback on the wave field by promoting the generation of higher vertical modes.

JFM classification

Geophysical and Geological Flows: Internal waves Geophysical and Geological Flows: Stratified flows Mixing: Turbulent mixing
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 932 , 10 February 2022 , A13
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aghsaee, P., Boegman, L. & Lamb, K.G. 2010 Breaking of shoaling internal solitary waves. J. Fluid Mech. 659, 289–317.CrossRefGoogle Scholar
Antenucci, J.P., Imberger, J. & Saggio, A. 2000 Seasonal evolution of the basin-scale internal wave field in a large stratified lake. Limnol. Oceanogr. 45 (7), 1621–1638.CrossRefGoogle Scholar
Armenio, V. & Piomelli, U. 2000 A lagrangian mixed subgrid-scale model in generalized coordinates. Flow Turbul. Combust. 65 (1), 51–81.CrossRefGoogle Scholar
Arthur, R.S. & Fringer, O.B. 2014 The dynamics of breaking internal solitary waves on slopes. J. Fluid Mech. 761, 360–398.CrossRefGoogle Scholar
Arthur, R.S. & Fringer, O.B. 2016 Transport by breaking internal gravity waves on slopes. J. Fluid Mech. 789, 93–126.CrossRefGoogle Scholar
Aucan, J., Merrifield, M.A., Luther, D.S. & Flament, P. 2006 Tidal mixing events on the deep flanks of Kaena Ridge, Hawaii. J. Phys. Oceanogr. 36 (6), 1202–1219.CrossRefGoogle Scholar
Boegman, L. 2009 Currents in stratified water bodies 2: internal waves. In Encyclopedia of Inland Waters (ed. G.E. Likens), pp. 539–558. Academic Press.CrossRefGoogle Scholar
Boegman, L., Imberger, J., Ivey, G.N. & Antenucci, J.P. 2003 High-frequency internal waves in large stratified lakes. Limnol. Oceanogr. 48 (2), 895–919.CrossRefGoogle Scholar
Boegman, L. & Ivey, G.N. 2007 Experiments on internal wave resonance in periodically forced lakes. In 5th International Symposium on Environmental Hydraulics, Tempe, Arizona.Google Scholar
Boegman, L. & Ivey, G.N. 2009 Flow separation and resuspension beneath shoaling nonlinear internal waves. J. Geophys. Res.: Oceans 114 (C2), C02018.CrossRefGoogle Scholar
Boegman, L. & Ivey, G.N. 2012 The dynamics of internal wave resonance in periodically forced narrow basins. J. Geophys. Res.: Oceans 117 (C11), C11002.CrossRefGoogle Scholar
Boegman, L., Ivey, G. & Imberger, J. 2005 a The degeneration of internal waves in lakes with sloping topography. Limnol. Oceanogr. 50 (5), 1620–1637.CrossRefGoogle Scholar
Boegman, L., Ivey, G. & Imberger, J. 2005 b The energetics of large-scale internal wave degeneration in lakes. J. Fluid Mech. 531, 159–180.CrossRefGoogle Scholar
Boehrer, B. & Schultze, M. 2008 Stratification of lakes. Rev. Geophys. 46 (2), RG2005.CrossRefGoogle Scholar
Bouffard, D. & Boegman, L. 2013 A diapycnal diffusivity model for stratified environmental flows. Dyn. Atmos. Oceans 61, 14–34.CrossRefGoogle Scholar
Caulfield, C. 2021 Layering, instabilities, and mixing in turbulent stratified flows. Annu. Rev. Fluid Mech. 53 (1), 113–145.CrossRefGoogle Scholar
Chen, G., Xiong, Q., Morris, P.J., Paterson, E.G., Sergeev, A. & Wang, Y. 2014 OpenFOAM for computational fluid dynamics. Not. Am. Math. Soc. 61 (4), 354–363.CrossRefGoogle Scholar
Cintolesi, C., Petronio, A. & Armenio, V. 2015 Large eddy simulation of turbulent buoyant flow in a confined cavity with conjugate heat transfer. Phys. Fluids 27 (9), 095109.CrossRefGoogle Scholar
Cooker, M., Weidman, P. & Bale, D. 1997 Reflection of a high-amplitude solitary wave at a vertical wall. J. Fluid Mech. 342, 141–158.CrossRefGoogle Scholar
Crank, J. & Nicolson, P. 1947 A practical method for numerical evaluation of solutions of partial differential equations of the heat-conduction type. In Mathematical Proceedings of the Cambridge Philosophical Society, vol. 43, pp. 50–67. Cambridge University Press.CrossRefGoogle Scholar
Csanady, G. 1982 On the structure of transient upwelling events. J. Phys. Oceanogr. 12 (1), 84–96.2.0.CO;2>CrossRefGoogle Scholar
Farmer, D.M. 1978 Observations of long nonlinear internal waves in a lake. J. Phys. Oceanogr. 8 (1), 63–73.2.0.CO;2>CrossRefGoogle Scholar
Farrow, D.E. & Stevens, C.L. 2003 Numerical modelling of a surface-stress driven density-stratified fluid. J. Engng Maths 47 (1), 1–16.CrossRefGoogle Scholar
Fricker, P.D. & Nepf, H.M. 2000 Bathymetry, stratification, and internal seiche structure. J. Geophys. Res.: Oceans 105 (C6), 14237–14251.CrossRefGoogle Scholar
Garrett, C. & Kunze, E. 2007 Internal tide generation in the deep ocean. Annu. Rev. Fluid Mech. 39, 57–87.CrossRefGoogle Scholar
Gayen, B. & Sarkar, S. 2010 Turbulence during the generation of internal tide on a critical slope. Phys. Rev. Lett. 104 (21), 218502.CrossRefGoogle ScholarPubMed
Gayen, B. & Sarkar, S. 2011 Direct and large-eddy simulations of internal tide generation at a near-critical slope. J. Fluid Mech. 681, 48–79.CrossRefGoogle Scholar
Grimshaw, R., Pelinovsky, E., Talipova, T. & Kurkina, O. 2010 Internal solitary waves: propagation, deformation and disintegration. Nonlinear Process. Geophys. 17 (6), 633–649.CrossRefGoogle Scholar
Helfrich, K.R. 1992 Internal solitary wave breaking and run-up on a uniform slope. J. Fluid Mech. 243, 133–154.CrossRefGoogle Scholar
Horn, D., Imberger, J. & Ivey, G. 2001 The degeneration of large-scale interfacial gravity waves in lakes. J. Fluid Mech. 434, 181–207.CrossRefGoogle Scholar
Horn, W., Mortimer, C.H. & Schwab, D.J. 1986 Wind-induced internal seiches in Lake Zurich observed and modeled 1. Limnol. Oceanogr. 31 (6), 1232–1254.CrossRefGoogle Scholar
Imberger, J. & Hamblin, P.F. 1982 Dynamics of lakes, reservoirs, and cooling ponds. Annu. Rev. Fluid Mech. 14 (1), 153–187.CrossRefGoogle Scholar
Issa, R.I., Gosman, A. & Watkins, A. 1986 The computation of compressible and incompressible recirculating flows by a non-iterative implicit scheme. J. Comput. Phys. 62 (1), 66–82.CrossRefGoogle Scholar
Jasak, H. 1996 Error Analysis and Estimation for the Finite Volume Method with Applications to Fluid Flows. PhD Thesis, University College London.Google Scholar
Koop, C.G. & Butler, G. 1981 An investigation of internal solitary waves in a two-fluid system. J. Fluid Mech. 112, 225–251.CrossRefGoogle Scholar
LaZerte, B.D. 1980 The dominating higher order vertical modes of the internal seiche in a small lake. Limnol. Oceanogr. 25 (5), 846–854.CrossRefGoogle Scholar
Lienhard, J.H. & Atta, V. 1990 The decay of turbulence in thermally stratified flow. J. Fluid Mech. 210, 57–112.CrossRefGoogle Scholar
Linden, P.F. 2018 Turbulence and mixing in flows dominated by buoyancy. In Mixing and Dispersion in Flows Dominated by Rotation and Buoyancy, pp. 25–60. Springer.CrossRefGoogle Scholar
Liu, H.-T. 1995 Energetics of grid turbulence in a stably stratified fluid. J. Fluid Mech. 296, 127–157.CrossRefGoogle Scholar
Liu, F. 2016 A thorough description of how wall functions are implemented in OpenFOAM. Proc. CFD OpenSour. Softw. 1–33.Google Scholar
Lorke, A. 2007 Boundary mixing in the thermocline of a large lake. J. Geophys. Res.: Oceans 112 (C9), C09019.CrossRefGoogle Scholar
Lorke, A., Peeters, F. & Wüest, A. 2005 Shear-induced convective mixing in bottom boundary layers on slopes. Limnol. Oceanogr. 50 (5), 1612–1619.CrossRefGoogle Scholar
Lorke, A., Umlauf, L., Jonas, T. & Wüest, A. 2002 Dynamics of turbulence in low-speed oscillating bottom-boundary layers of stratified basins. Environ. Fluid Mech. 2 (4), 291–313.CrossRefGoogle Scholar
MacIntyre, S., Clark, J.F., Jellison, R. & Fram, J.P. 2009 Turbulent mixing induced by nonlinear internal waves in Mono Lake, California. Limnol. Oceanogr. 54 (6), 2255–2272.CrossRefGoogle Scholar
MacIntyre, S., Flynn, K.M., Jellison, R. & Romero, J.R. 1999 Boundary mixing and nutrient fluxes in Mono Lake, California. Limnol. Oceanogr. 44 (3), 512–529.CrossRefGoogle Scholar
Magni, D., Chinaglia, N., Maffotti, A., Borasi, L., Lefebvre, P., Zanella, D., Brancelj, A., Mori, N., Pagon, P. & Urbanc-Berčič, O. 2008 Alpine Lakes: A Common Approach to the Characterization of Lakes and their Catchmet Area.Google Scholar
Meneveau, C., Lund, T.S. & Cabot, W.H. 1996 A lagrangian dynamic subgrid-scale model of turbulence. J. Fluid Mech. 319, 353–385.CrossRefGoogle Scholar
Michallet, H. & Ivey, G. 1999 Experiments on mixing due to internal solitary waves breaking on uniform slopes. J. Geophys. Res.: Oceans 104 (C6), 13467–13477.CrossRefGoogle Scholar
Monismith, S.G. 1985 Wind-forced motions in stratified lakes and their effect on mixed-layer shear. Limnol. Oceanogr. 30 (4), 771–783.CrossRefGoogle Scholar
Monismith, S. 1986 An experimental study of the upwelling response of stratified reservoirs to surface shear stress. J. Fluid Mech. 171, 407–439.CrossRefGoogle Scholar
Monismith, S. 1987 Modal response of reservoirs to wind stress. J. Hydraul. Engng ASCE 113 (10), 1290–1304.CrossRefGoogle Scholar
Mortimer, C. 1953 The resonant response of stratified lakes to wind. Aquat. Sci. 15 (1), 94–151.CrossRefGoogle Scholar
Münnich, M. 1996 The influence of bottom topography on internal seiches in stratified media. Dyn. Atmos. Oceans 23 (1-4), 257–266.CrossRefGoogle Scholar
Münnich, M., Wüest, A. & Imboden, D.M. 1992 Observations of the second vertical mode of the internal seiche in an alpine lake. Limnol. Oceanogr. 37 (8), 1705–1719.CrossRefGoogle Scholar
Nakayama, K., Sato, T., Shimizu, K. & Boegman, L. 2019 Classification of internal solitary wave breaking over a slope. Phys. Rev. Fluids 4 (1), 014801.CrossRefGoogle Scholar
Osborn, T.R. & Cox, C.S. 1972 Oceanic fine structure. Geophys. Astrophys. Fluid Dyn. 3 (1), 321– 345.CrossRefGoogle Scholar
Peltier, W. & Caulfield, C. 2003 Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech. 35 (1), 135–167.CrossRefGoogle Scholar
Robertson, E., Choudhury, V., Bhushan, S. & Walters, D.K. 2015 Validation of OpenFOAM numerical methods and turbulence models for incompressible bluff body flows. Comput. Fluids 123, 122–145.CrossRefGoogle Scholar
Roget, E., Salvadé, G. & Zamboni, F. 1997 Internal seiche climatology in a small lake where transversal and second vertical modes are usually observed. Limnol. Oceanogr. 42 (4), 663–673.CrossRefGoogle Scholar
Rozas, C., de la Fuente, A., Ulloa, H., Davies, P. & Niño, Y. 2014 Quantifying the effect of wind on internal wave resonance in Lake Villarrica, Chile. Environ. Fluid Mech. 14 (4), 849–871.Google Scholar
Saggio, A. & Imberger, J. 2001 Mixing and turbulent fluxes in the metalimnion of a stratified lake. Limnol. Oceanogr. 46 (2), 392–409.CrossRefGoogle Scholar
Salon, S., Armenio, V. & Crise, A. 2007 A numerical investigation of the stokes boundary layer in the turbulent regime. J. Fluid Mech. 570, 253–296.CrossRefGoogle Scholar
Sarkar, S. & Scotti, A. 2017 From topographic internal gravity waves to turbulence. Annu. Rev. Fluid Mech. 49, 195–220.CrossRefGoogle Scholar
Scotti, A. & White, B. 2014 Diagnosing mixing in stratified turbulent flows with a locally defined available potential energy. J. Fluid Mech. 740, 114–135.CrossRefGoogle Scholar
Shih, L.H., Koseff, J.R., Ivey, G.N. & Ferziger, J.H. 2005 Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech. 525, 193–214.CrossRefGoogle Scholar
Shintani, T., de la Fuente, A., Niño, Y. & Imberger, J. 2010 Generalizations of the Wedderburn number: parameterizing upwelling in stratified lakes. Limnol. Oceanogr. 55 (3), 1377–1389.CrossRefGoogle Scholar
Smagorinsky, J. 1963 General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weath. Rev. 91 (3), 99–164.2.3.CO;2>CrossRefGoogle Scholar
Spalart, P.R. 2000 Strategies for turbulence modelling and simulations. Intl J. Heat Fluid Flow 21 (3), 252–263.CrossRefGoogle Scholar
Spigel, R.H. & Imberger, J. 1980 The classification of mixed-layer dynamics of lakes of small to medium size. J. Phys. Oceanogr. 10 (7), 1104–1121.2.0.CO;2>CrossRefGoogle Scholar
Stevens, C. & Imberger, J. 1996 The initial response of a stratified lake to a surface shear stress. J. Fluid Mech. 312, 39–66.CrossRefGoogle Scholar
Tang, C., Patel, V. & Landweber, L. 1990 Viscous effects on propagation and reflection of solitary waves in shallow channels. J. Comput. Phys. 88 (1), 86–113.CrossRefGoogle Scholar
Thompson, R. & Imberger, J. 1980 Response of a numerical model of a stratified lake to wind stress. In Proceedings of Second International Symposium on Stratified Flows, IAHR, 1980. pp. 562–570.Google Scholar
Thorpe, S. 1998 Some dynamical effects of internal waves and the sloping sides of lakes. Coast. Estuar. Stud. 441–460.Google Scholar
Ulloa, H.N., Constantinescu, G., Chang, K., Horna-Munoz, D., Hames, O. & Wüest, A. 2020 Horizontal transport under wind-induced resonance in stratified waterbodies. Phys. Rev. Fluids 5 (5), 054503.CrossRefGoogle Scholar
Ulloa, H.N., Constantinescu, G., Chang, K., Horna-Munoz, D., Steiner, O.S., Bouffard, D. & Wüest, A. 2019 Hydrodynamics of a periodically wind-forced small and narrow stratified basin: a large-eddy simulation experiment. Environ. Fluid Mech. 19 (3), 667–698.CrossRefGoogle Scholar
Valerio, G., Pilotti, M., Marti, C.L. & Imberger, J. r. 2012 The structure of basin-scale internal waves in a stratified lake in response to lake bathymetry and wind spatial and temporal distribution: Lake Iseo, Italy. Limnol. Oceanogr. 57 (3), 772–786.CrossRefGoogle Scholar
Van Leer, B. 1974 Towards the ultimate conservative difference scheme. ii. monotonicity and conservation combined in a second-order scheme. J. Comput. Phys. 14 (4), 361–370.CrossRefGoogle Scholar
VanDine, A., Pham, H.T. & Sarkar, S. 2021 Turbulent shear layers in a uniformly stratified background: DNS at high Reynolds number. J. Fluid Mech. 916, A42.CrossRefGoogle Scholar
Vidal, J., Rueda, F.J. & Casamitjana, X. 2007 The seasonal evolution of high vertical-mode internal waves in a deep reservoir. Limnol. Oceanogr. 52 (6), 2656–2667.CrossRefGoogle Scholar
Villermaux, E. 2019 Mixing versus stirring. Annu. Rev. Fluid Mech. 51, 245–273.CrossRefGoogle Scholar
Vlasenko, V. & Hutter, K. 2002 Transformation and disintegration of strongly nonlinear internal waves by topography in stratified lakes. In Annales Geophysicae, vol. 20, pp. 2087–2103. Copernicus GmbH.CrossRefGoogle Scholar
Wain, D.J., Kohn, M.S., Scanlon, J.A. & Rehmann, C.R. 2013 Internal wave-driven transport of fluid away from the boundary of a lake. Limnol. Oceanogr. 58 (2), 429–442.CrossRefGoogle Scholar
Wain, D.J. & Rehmann, C.R. 2010 Transport by an intrusion generated by boundary mixing in a lake. Water Resour. Res. 46 (8), W08517.CrossRefGoogle Scholar
Weller, H.G., Tabor, G., Jasak, H. & Fureby, C. 1998 A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 12 (6), 620–631.CrossRefGoogle Scholar
Wiegand, R.C. & Chamberlain, V. 1987 Internal waves of the second vertical mode in a stratified lake. Limnol. Oceanogr. 32 (1), 29–42.CrossRefGoogle Scholar
Winters, K.B., Lombard, P.N., Riley, J.J. & D'Asaro, E.A. 1995 Available potential energy and mixing in density-stratified fluids. J. Fluid Mech. 289, 115–128.CrossRefGoogle Scholar