Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T18:34:09.842Z Has data issue: false hasContentIssue false
  • English
  • Français

Kelvin–Helmholtz billows above Richardson number 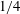$1/4$

Published online by Cambridge University Press:  23 September 2019

J. P. Parker Open the ORCID record for J. P. Parker [Opens in a new window] ,
C. P. Caulfield Open the ORCID record for C. P. Caulfield [Opens in a new window]  and
R. R. Kerswell
J. P. Parker*
Affiliation:
Department of Applied Mathematics and Theoretical Physics, Centre for Mathematical Sciences, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK
C. P. Caulfield
Affiliation:
Department of Applied Mathematics and Theoretical Physics, Centre for Mathematical Sciences, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK BP Institute, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
R. R. Kerswell
Affiliation:
Department of Applied Mathematics and Theoretical Physics, Centre for Mathematical Sciences, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the dynamical system of a two-dimensional, forced, stratified mixing layer at finite Reynolds number 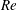$Re$, and Prandtl number $Pr=1$. We consider a hyperbolic tangent background velocity profile in the two cases of hyperbolic tangent and uniform background buoyancy stratifications, in a domain of fixed, finite width and height. The system is forced in such a way that these background profiles are a steady solution of the governing equations. As is well known, if the minimum gradient Richardson number of the flow, 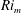$Ri_{m}$, is less than a certain critical value 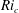$Ri_{c}$, the flow is linearly unstable to Kelvin–Helmholtz instability in both cases. Using Newton–Krylov iteration, we find steady, two-dimensional, finite-amplitude elliptical vortex structures – i.e. ‘Kelvin–Helmholtz billows’ – existing above $Ri_{c}$. Bifurcation diagrams are produced using branch continuation, and we explore how these diagrams change with varying 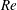$Re$. In particular, when 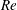$Re$ is sufficiently high we find that finite-amplitude Kelvin–Helmholtz billows exist when $Ri_{m}>1/4$ for the background flow, which is linearly stable by the Miles–Howard theorem. For the uniform background stratification, we give a simple explanation of the dynamical system, showing the dynamics can be understood on a two-dimensional manifold embedded in state space, and demonstrate the cases in which the system is bistable. In the case of a hyperbolic tangent stratification, we also describe a new, slow-growing, linear instability of the background profiles at finite 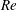$Re$, which complicates the dynamics.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Geophysical and Geological Flows: Stratified flows Instability: Nonlinear instability
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 879 , 25 November 2019 , R1
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Brown, S. N., Rosen, A. S. & Maslowe, S. A. 1981 The evolution of a quasi-steady critical layer in a stratified viscous shear layer. Proc. R. Soc. Lond. A 375 (1761), 271–293.Google Scholar
Chandler, G. J. & Kerswell, R. R. 2013 Invariant recurrent solutions embedded in a turbulent two-dimensional Kolmogorov flow. J. Fluid Mech. 722, 554–595.10.1017/jfm.2013.122Google Scholar
Churilov, S. M. & Shukhman, I. G. 1987 Nonlinear stability of a stratified shear flow: a viscous critical layer. J. Fluid Mech. 180, 1–20.10.1017/S0022112087001708Google Scholar
Defina, A., Lanzoni, S. & Susin, F. M. 1999 Stability of a stratified viscous shear flow in a tilted tube. Phys. Fluids 11 (2), 344–355.10.1063/1.869884Google Scholar
Dijkstra, H. A., Wubs, F. W., Cliffe, A. K., Doedel, E., Dragomirescu, I. F., Eckhardt, B., Gelfgat, A. Y., Hazel, A. L., Lucarini, V., Salinger, A. G. et al. 2014 Numerical bifurcation methods and their application to fluid dynamics: Analysis beyond simulation. Commun. Comput. Phys. 15 (1), 1–45.10.4208/cicp.240912.180613aGoogle Scholar
Drazin, P. G. 1958 The stability of a shear layer in an unbounded heterogeneous inviscid fluid. J. Fluid Mech. 4 (2), 214–224.10.1017/S0022112058000409Google Scholar
Edwards, W. S., Tuckerman, L. S., Friesner, R. A. & Sorensen, D. C. 1994 Krylov methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 110 (1), 82–102.10.1006/jcph.1994.1007Google Scholar
Haines, P. E., Hewitt, R. E. & Hazel, A. L. 2011 The Jeffery–Hamel similarity solution and its relation to flow in a diverging channel. J. Fluid Mech. 687, 404–430.10.1017/jfm.2011.362Google Scholar
Hazel, P. 1972 Numerical studies of the stability of inviscid stratified shear flows. J. Fluid Mech. 51 (1), 39–61.10.1017/S0022112072001065Google Scholar
Holmboe, J.1960 Unpublished lecture notes.Google Scholar
Howard, L. N. 1961 Note on a paper of John W. Miles. J. Fluid Mech. 10 (4), 509–512.10.1017/S0022112061000317Google Scholar
Howland, C. J., Taylor, J. R. & Caulfield, C. P. 2018 Testing linear marginal stability in stratified shear layers. J. Fluid Mech. 839, R4.10.1017/jfm.2018.79Google Scholar
Kaminski, A. K., Caulfield, C. P. & Taylor, J. R. 2014 Transient growth in strongly stratified shear layers. J. Fluid Mech. 758, R4.10.1017/jfm.2014.552Google Scholar
Kaminski, A. K., Caulfield, C. P. & Taylor, J. R. 2017 Nonlinear evolution of linear optimal perturbations of strongly stratified shear layers. J. Fluid Mech. 825, 213–244.10.1017/jfm.2017.396Google Scholar
Keller, H. B. 1977 Numerical solution of bifurcation and nonlinear eigenvalue problems. In Applications of Bifurcation Theory (ed. Rabinowitz, P. H.), pp. 359–384. Academic Press.Google Scholar
Klaassen, G. P. & Peltier, W. R. 1985 Evolution of finite amplitude Kelvin–Helmholtz billows in two spatial dimensions. J. Atmos. Sci. 42 (12), 1321–1339.10.1175/1520-0469(1985)042<1321:EOFAKB>2.0.CO;22.0.CO;2>Google Scholar
Lott, F. & Teitelbaum, H. 1992 Nonlinear dissipative critical level interaction in a stratified shear flow: instabilities and gravity waves. Geophys. Astrophys. Fluid Dyn. 66 (1–4), 133–167.10.1080/03091929208229054Google Scholar
Mallier, R. 2003 Stuart vortices in a stratified mixing layer: the Holmboe model. J. Engng. Maths. 47 (2), 121–136.10.1023/A:1025890823745Google Scholar
Maslowe, S. A. 1973 Finite-amplitude Kelvin–Helmholtz billows. Boundary-Layer Meteorol. 5 (1), 43–52.10.1007/BF02188310Google Scholar
Maslowe, S. A. 1977 Weakly nonlinear stability theory of stratified shear flows. Q. J. R. Meteorol. Soc. 103 (438), 769–783.10.1002/qj.49710343817Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10 (4), 496–508.10.1017/S0022112061000305Google Scholar
Miles, J. W. 1963 On the stability of heterogeneous shear flows. Part 2. J. Fluid Mech. 16 (2), 209–227.10.1017/S0022112063000707Google Scholar
Miller, R. L. & Lindzen, R. S. 1988 Viscous destabilization of stratified shear flow for Ri > 1/4. Geophys. Astrophys. Fluid Dyn. 42 (1–2), 49–91.10.1080/03091928808208858+1/4.+Geophys.+Astrophys.+Fluid+Dyn.+42+(1–2),+49–91.10.1080/03091928808208858>Google Scholar
Mkhinini, N., Dubos, T. & Drobinski, P. 2013 On the nonlinear destabilization of stably stratified shear flow. J. Fluid Mech. 731, 443–460.10.1017/jfm.2013.374Google Scholar
Nagata, M. 1990 Three-dimensional finite-amplitude solutions in plane Couette flow: bifurcation from infinity. J. Fluid Mech. 217, 519–527.10.1017/S0022112090000829Google Scholar
Net, M. & Sánchez, J. 2015 Continuation of bifurcations of periodic orbits for large-scale systems. SIAM J. Appl. Dyn. Syst. 14 (2), 674–698.10.1137/140981010Google Scholar
Saad, Y. & Schultz, M. H. 1986 GMRES: a generalized minimal residual algorithm for solving nonsymmetric linear systems. SIAM J. Sci. Comput. 7 (3), 856–869.Google Scholar
Salinger, A. G., Bou-Rabee, N. M., Burroughs, E. A., Pawlowski, R. P., Lehoucq, R. B., Romero, L. & Wilkes, E. D.2002 LOCA 1.0 Library of continuation algorithms: theory and implementation manual.10.2172/800778Google Scholar
Sánchez, J. & Net, M. 2016 Numerical continuation methods for large-scale dissipative dynamical systems. Eur. Phys. J. Spec. Top. 225 (13), 2465–2486.Google Scholar
Smyth, W. D. & Carpenter, J. R. 2019 Instability in Geophysical Flows. Cambridge University Press.10.1017/9781108640084Google Scholar
Smyth, W. D., Nash, J. D. & Moum, J. N. 2019 Self-organized criticality in geophysical turbulence. Sci. Rep. 9 (1), 3747.Google Scholar
Smyth, W. D. & Peltier, W. R. 1991 Instability and transition in finite-amplitude Kelvin–Helmholtz and Holmboe waves. J. Fluid Mech. 228, 387–415.Google Scholar
Strogatz, S. H. 2014 Nonlinear Dynamics and Chaos: with Applications to Physics, Biology, Chemistry, and Engineering. CRC Press.Google Scholar
Taylor, J. R.2008 Numerical simulations of the stratified oceanic bottom layer. PhD thesis.Google Scholar
Thorpe, S. A., Smyth, W. D. & Li, L. 2013 The effect of small viscosity and diffusivity on the marginal stability of stably stratified shear flows. J. Fluid Mech. 731, 461–476.10.1017/jfm.2013.378Google Scholar