Article contents
Intermittency in turbulent emulsions
Published online by Cambridge University Press: 06 October 2023
Abstract
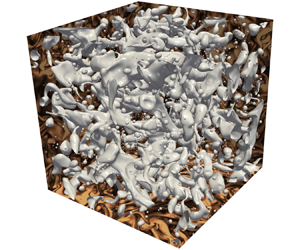
We investigate the statistics of turbulence in emulsions of two immiscible fluids of the same density. We compute velocity increments between points conditioned to be located in the same phase or in different phases, and examine their probability density functions (PDFs) and the associated structure functions (SFs). This enables us to demonstrate that the presence of the interface reduces the skewness of the PDF at small scales and therefore the magnitude of the energy flux towards the dissipative scales, which is quantified by the third-order SF. The analysis of the higher-order SFs shows that multiphase turbulence is more intermittent than single-phase turbulence. In particular, the local scaling exponents of the SFs display a saturation below the Kolmogorov–Hinze scale, which indicates the presence of large velocity gradients across the interface. Interestingly, the statistics of the velocity differences in the carrier phase recovers that of single-phase turbulence when the viscosity of the dispersed phase is high.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 3
- Cited by



