Published online by Cambridge University Press: 19 September 2019
The hydrodynamic lift velocity of a neutrally buoyant fibre in a simple shear flow near a wall is determined for small, but non-zero, fibre Reynolds number, illustrating the role of non-sphericity in lift. The rotational motion and effects of fibre orientation on lift are treated for fibre positions that induce and do not induce solid-body wall contacts. When the fibre does not contact the wall its lift velocity can be obtained in terms of the Stokes flow field by using a generalized reciprocal theorem. The Stokes velocity field is determined using slender-body theory with the no-slip velocity at the wall enforced using the method of images. To leading order the lift velocity at distances large compared with the fibre length and small compared with the Oseen length is found to be 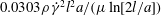 $0.0303\unicode[STIX]{x1D70C}\dot{\unicode[STIX]{x1D6FE}}^{2}l^{2}a/(\unicode[STIX]{x1D707}\ln [2l/a])$, where
$0.0303\unicode[STIX]{x1D70C}\dot{\unicode[STIX]{x1D6FE}}^{2}l^{2}a/(\unicode[STIX]{x1D707}\ln [2l/a])$, where 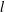 $l$ and
$l$ and  $a$ are the fibre half-length and radius,
$a$ are the fibre half-length and radius, 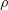 $\unicode[STIX]{x1D70C}$ is the density,
$\unicode[STIX]{x1D70C}$ is the density,  $\dot{\unicode[STIX]{x1D6FE}}$ is the shear rate and
$\dot{\unicode[STIX]{x1D6FE}}$ is the shear rate and 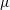 $\unicode[STIX]{x1D707}$ is the viscosity of the fluid. When the fibre is close enough to the wall to make solid-body contact during its rotational motion, a process known as pole vaulting coupled with inertially induced changes of fibre orientation determines the lift velocity. The order of magnitude of the lift in this case is larger by a factor of
$\unicode[STIX]{x1D707}$ is the viscosity of the fluid. When the fibre is close enough to the wall to make solid-body contact during its rotational motion, a process known as pole vaulting coupled with inertially induced changes of fibre orientation determines the lift velocity. The order of magnitude of the lift in this case is larger by a factor of 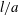 $l/a$ than when the fibre does not contact the wall and it reaches a maximum of
$l/a$ than when the fibre does not contact the wall and it reaches a maximum of 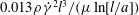 $0.013\unicode[STIX]{x1D70C}\dot{\unicode[STIX]{x1D6FE}}^{2}l^{3}/(\unicode[STIX]{x1D707}\ln [l/a])$ for the case of a highly frictional contact and about half that value for a frictionless contact. These results are used to illustrate how particle shape can contribute to separation methods such as those in microfluidic channels or cross-flow filtration processes.
$0.013\unicode[STIX]{x1D70C}\dot{\unicode[STIX]{x1D6FE}}^{2}l^{3}/(\unicode[STIX]{x1D707}\ln [l/a])$ for the case of a highly frictional contact and about half that value for a frictionless contact. These results are used to illustrate how particle shape can contribute to separation methods such as those in microfluidic channels or cross-flow filtration processes.