Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-28T18:35:39.840Z Has data issue: false hasContentIssue false
  • English
  • Français

High Rayleigh number convection in a three-dimensional porous medium

Published online by Cambridge University Press:  09 May 2014

Duncan R. Hewitt ,
Jerome A. Neufeld  and
John R. Lister
Duncan R. Hewitt*
Affiliation:
Institute of Theoretical Geophysics, Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK
Jerome A. Neufeld
Affiliation:
Institute of Theoretical Geophysics, Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK Bullard Laboratories, Department of Earth Science, University of Cambridge, Cambridge CB3 0EZ, UK BP Institute, University of Cambridge, Cambridge CB3 0EZ, UK
John R. Lister
Affiliation:
Institute of Theoretical Geophysics, Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

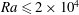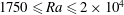High-resolution numerical simulations of statistically steady convection in a three-dimensional porous medium are presented for Rayleigh numbers $Ra \leqslant 2 \times 10^4$. Measurements of the Nusselt number $Nu$ in the range $1750 \leqslant Ra \leqslant 2 \times 10^4$ are well fitted by a relationship of the form 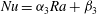$Nu = \alpha _3 Ra + \beta _3$, for 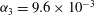$\alpha _3 = 9.6 \times 10^{-3}$ and 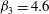$\beta _3 = 4.6$. This fit indicates that the classical linear scaling $Nu \sim Ra$ is attained, and that 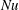$Nu$ is asymptotically approximately $40\, \%$ larger than in two dimensions. The dynamical flow structure in the range 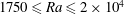$1750 \leqslant Ra \leqslant 2\times 10^4$ is analysed, and the interior of the flow is found to be increasingly well described as $Ra \to \infty $ by a heat-exchanger model, which describes steady interleaving columnar flow with horizontal wavenumber 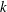$k$ and a linear background temperature field. Measurements of the interior wavenumber are approximately fitted by 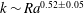$k\sim Ra^{0.52 \pm 0.05}$, which is distinguishably stronger than the two-dimensional scaling of $k\sim Ra^{0.4}$.

JFM classification

Convection: Convection Convection: Convection in porous media Low-Reynolds-number flows: Porous media
Type
Papers
Information
Journal of Fluid Mechanics , Volume 748 , 10 June 2014 , pp. 879 - 895
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.CrossRefGoogle Scholar
Bickle, M. J. 2009 Geological carbon storage. Nature Geosci. 2, 815–819.Google Scholar
Brian, P. L. T. 1961 A finite-difference method of high-order accuracy for the solution of three-dimensional transient heat conduction problems. AIChE J. 7, 367–370.Google Scholar
Corson, L. T.2011 Maximising the heat flux in steady unicellular porous media convection. In Proceedings of the 2011 Geophysical Fluid Dynamics Summer Program, Woods Hole Oceanographic Institution, pp. 389–412.Google Scholar
Cross, M. C. & Hohenberg, P. C. 1993 Pattern formation outside of equilibrium. Rev. Mod. Phys. 65, 851–1112.Google Scholar
E, W. & Liu, J. G. 1997 Finite difference methods for 3D viscous incompressible flows in the vorticity-vector potential formulation on nonstaggered grids. J. Comput. Phys. 138, 57–82.CrossRefGoogle Scholar
Elder, J. W. 1967 Steady free convection in a porous medium heated from below. J. Fluid Mech. 27, 29–48.Google Scholar
Fu, X., Cueto-Felgueroso, L. & Juanes, R. 2013 Pattern formation and coarsening dynamics in three-dimensional convective mixing in porous media. Phil. Trans. R. Soc. A 371, 20120355.Google Scholar
Graham, M. D. & Steen, P. H. 1994 Plume formation and resonant bifurcations in porous-media convection. J. Fluid Mech. 272, 67–89.Google Scholar
Hewitt, D. R., Neufeld, J. A. & Lister, J. R. 2012 Ultimate regime of high Rayleigh number convection in a porous medium. Phys. Rev. Lett. 108, 224503.Google Scholar
Hewitt, D. R., Neufeld, J. A. & Lister, J. R. 2013a Convective shutdown in a porous medium at high Rayleigh number. J. Fluid Mech. 719, 551–586.Google Scholar
Hewitt, D. R., Neufeld, J. A. & Lister, J. R. 2013b Stability of columnar convection in a porous medium. J. Fluid Mech. 737, 205–231.Google Scholar
Holst, P. H. & Aziz, K. 1972 Transient three-dimensional natural convection in confined porous media. Intl J. Heat Mass Transfer 15, 73–90.Google Scholar
Howard, L. N.1964 Convection at high Rayleigh number. In Applied Mechanics, Proc. 11th Intl Cong. Appl. Math. (ed. H. Görtler), pp. 1109–1115.Google Scholar
Huppert, H. E. & Neufeld, J. A. 2014 The fluid mechanics of carbon dioxide sequestration. Annu. Rev. Fluid Mech. 46, 255–272.Google Scholar
Kadanoff, L. P. 2001 Turbulent heat flow: structures and scaling. Phys. Today 54, 34–39.Google Scholar
Kimura, S., Schubert, G. & Straus, J. M. 1989 Time-dependent convection in a fluid-saturated porous cube heated from below. J. Fluid Mech. 207, 153–189.CrossRefGoogle Scholar
Lapwood, E. R. 1948 Convection of a fluid in a porous medium. Math. Proc. Cambridge Philos. Soc. 44, 508–521.Google Scholar
Lister, C. R. B. 1990 An explanation for the multivalued heat transport found experimentally for convection in a porous medium. J. Fluid Mech. 214, 287–320.Google Scholar
Metz, B., Davidson, O., de Coninck, H. C., Loos, M. & Meyer, L.2005 IPCC special report on carbon dioxide capture and storage. Cambridge University Press.Google Scholar
Nield, D. A. & Bejan, A. 2006 Convection in Porous Media. 3rd edn Springer.Google Scholar
Otero, J., Dontcheva, L. A., Johnston, H., Worthing, R. A., Kurganov, A., Petrova, G. & Doering, C. R. 2004 High-Rayleigh-number convection in a fluid-saturated porous layer. J. Fluid Mech. 500, 263–281.Google Scholar
Pau, G. S. H., Bell, J. B., Pruess, K., Almgren, A. S., Lijewski, M. J. & Zhang, K. 2010 High-resolution simulation and characterization of density-driven flow in ${\mathrm{CO}_{2}}$ storage in saline aquifers. Adv. Water Resour. 33, 443–455.Google Scholar
Schubert, G. & Straus, J. M. 1979 Three-dimensional and multicellular steady and unsteady convection in fluid-saturated porous media at high Rayleigh numbers. J. Fluid Mech. 94, 25–38.Google Scholar
Straus, J. M. & Schubert, G. 1979 Three-dimensional convection in a cubic box of fluid-saturated porous material. J. Fluid Mech. 91, 155–165.Google Scholar
Straus, J. M. & Schubert, G. 1981 Modes of finite-amplitude three-dimensional convection in rectangular boxes of fluid-saturated porous material. J. Fluid Mech. 103, 23–32.Google Scholar
Wen, B., Chini, G. P., Dianati, N. & Doering, C. R. 2013 Computational approaches to aspect-ratio-dependent upper bounds and heat flux in porous medium convection. Phys. Lett. A 377, 2931–2938.Google Scholar