Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-12-01T01:16:48.632Z Has data issue: false hasContentIssue false
  • English
  • Français

Generation of steady longitudinal vortices in hypersonic boundary layer

Published online by Cambridge University Press:  24 July 2013

A. I. Ruban  and
M. A. Kravtsova
A. I. Ruban*
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen’s Gate, London SW7 2BZ, UK
M. A. Kravtsova
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen’s Gate, London SW7 2BZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we study the three-dimensional perturbations produced in a hypersonic boundary layer by a small wall roughness. The flow analysis is performed under the assumption that the Reynolds number, 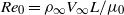$R{e}_{0} = {\rho }_{\infty } {V}_{\infty } L/ {\mu }_{0} $, and Mach number, 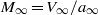${M}_{\infty } = {V}_{\infty } / {a}_{\infty } $, are large, but the hypersonic interaction parameter, 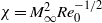$\chi = { M}_{\infty }^{2} R{ e}_{0}^{- 1/ 2} $, is small. Here 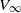${V}_{\infty } $, 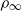${\rho }_{\infty } $ and 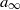${a}_{\infty } $ are the flow velocity, gas density and speed of sound in the free stream, 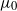${\mu }_{0} $ is the dynamic viscosity coefficient at the ‘stagnation temperature’, and 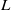$L$ is the characteristic distance the boundary layer develops along the body surface before encountering a roughness. We choose the longitudinal and spanwise dimensions of the roughness to be 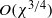$O({\chi }^{3/ 4} )$ quantities. In this case the flow field around the roughness may be described in the framework of the hypersonic viscous–inviscid interaction theory, also known as the triple-deck model. Our main interest in this paper is the nonlinear behaviour of the perturbations. We study these by means of numerical solution of the triple-deck equations, for which purpose a modification of the ‘skewed shear’ technique suggested by Smith (United Technologies Research Center Tech. Rep. 83-46, 1983) has been used. The technique requires global iterations to adjust the viscous and inviscid parts of the flow. Convergence of such iterations is known to be a major problem in viscous–inviscid calculations. In order to achieve improved stability of the method, both the momentum equation for the viscous part of the flow, and the equations describing the interaction with the flow outside the boundary layer, are treated implicitly in this study. The calculations confirm the fact that in this sort of flow the perturbations are capable of propagating upstream in the boundary layer, resulting in a perturbation field which surrounds the roughness on all sides. We found that the perturbations decay rather fast with the distance from the roughness everywhere except in the wake behind the roughness. We found that if the height of the roughness is small, then the perturbations also decay in the wake, though much more slowly than outside the wake. However, if the roughness height exceeds some critical value, then two symmetric counter-rotating vortices form in the wake. They appear to support themselves and grow as the distance from the roughness increases.

JFM classification

Compressible Flows: Compressible boundary layers Wakes/Jets: Separated flows Boundary Layers: Boundary layer receptivity
Type
Papers
Information
Journal of Fluid Mechanics , Volume 729 , 25 August 2013 , pp. 702 - 731
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bogolepov, V. V. 1986 General scheme of three-dimensional local flow regimes. Zh. Prikl. Mech. Tekh. Fiz. 6, 80–91.Google Scholar
Bogolepov, V. V. & Lipatov, I. I. 1985 Locally three-dimensional laminar flows. Zh. Prikl. Mech. Tekh. Fiz. 1, 28–36.Google Scholar
Bogolepov, V. V. & Neiland, V. Y. 1971 Supersonic flow over a surface with local distortions. TsAGI Trans. 1363, 1–12.Google Scholar
Bos, S., Kravtsova, M. A., Ruban, A. I. & Walker, J. D. A. 2000 Numerical analysis of 3D viscous–inviscid interaction and boundary layer separation. University of Manchester Tech. Rep. CLSCM-2000-001.Google Scholar
Choudhari, M. & Duck, P. W. 1996 Nonlinear excitation of inviscid stationary vortex instabilities in boundary layer flow. In Proceedings of the IUTAM Symposium on Nonlinear Instability and Transition in Three-Dimensional Boundary Layers (ed. Duck, P. W. & Hall, P.), pp. 409–422. Kluwer.Google Scholar
Duck, P. W. & Burggraf, O. R. 1986 Spectral solution for three-dimensional triple-deck flow over surface topography. J. Fluid Mech. 162, 1–21.Google Scholar
Goldstein, M. E., Sescu, A., Duck, P. W. & Choudhari, M. 2010 The long range persistence of wakes behind a row of roughness elements. J. Fluid Mech. 644, 123–163.CrossRefGoogle Scholar
Goldstein, M. E., Sescu, A., Duck, P. W. & Choudhari, M. 2011 Algebraic/transcendental disturbance growth behind a row of roughness elements. J. Fluid Mech. 668, 236–266.Google Scholar
Korolev, G. L. 2007 Numerical method for solving three-dimensional viscous–inviscid interaction equations. J. Vych. Mat. Mat. Fiz. 47 (3), 506–529.Google Scholar
Kozlova, I. G. & Mikhailov, V. V. 1970 On strong viscous interaction on Delta and swept wings. Izv. Akad. Nauk SSSR Mech. Zhidk. Gaza 94–99.Google Scholar
Lipatov, I. I. & Vinogradov, I. V. 2000 Three-dimensional flow over surface distortions. Phil. Trans. R. Soc. Lond. A 358, 3037–3045.CrossRefGoogle Scholar
Messiter, A. F. 1970 Boundary-layer flow near the trailing edge of a flat plate. SIAM J. Appl. Maths 18 (1), 241–257.CrossRefGoogle Scholar
Neiland, V. Y. 1969 Theory of laminar boundary layer separation in supersonic flow. Izv. Akad. Nauk SSSR Mech. Zhidk. Gaza (4), 53–57.Google Scholar
Neiland, V. Y. 1970 Upstream propagation of disturbances in the boundary layer interacting with hypersonic flow. Izv. Akad. Nauk SSSR Mech. Zhidk. Gaza 40–49.Google Scholar
Neiland, V. Y., Bogolepov, V. V., Dudin, G. & Lipatov, I. I. 2007 Asymptotic Theory of Supersonic Viscous Gas Flows. Elsevier.Google Scholar
Rozhko, S. B. & Ruban, A. I. 1987 Criss-cross interaction in a three-dimensional boundary layer. Izv. Akad. Nauk SSSR Mech. Zhidk. Gaza (3), 42–50.Google Scholar
Rozhko, S. B., Ruban, A. I. & Timoshin, S. N. 1988 Interaction of a three-dimensional boundary layer with a small aspect ratio obstacle. Izv. Akad. Nauk SSSR Mech. Zhidk. Gaza (1), 39–48.Google Scholar
Ruban, A. I. & Sychev, V. V. 1973 Hypersonic viscous gas flow over a small aspect ratio wing. Uch. Zap. TsAGI 4, 18–25.Google Scholar
Smith, F. T. 1976 Pipeflows distorted by non-symmetric indentation or branching. Mathematika 23, 62–83.Google Scholar
Smith, F. T. 1980 A three-dimensional boundary-layer separation. J. Fluid Mech. 99, 185–176.Google Scholar
Smith, F. T. 1983 Properties, and a finite-difference approach, for interactive three-dimensional boundary layers. Tech. Rep. 83-46. United Technologies Research Center.Google Scholar
Smith, F. T. & Gajjar, J. 1984 Flow past wing–body junctions. J. Fluid Mech. 144, 191–215.Google Scholar
Smith, F. T., Sykes, R. I. & Brighton, P. W. M. 1977 A two-dimensional boundary layer encountering a three-dimensional hump. J. Fluid Mech. 83, 163–176.Google Scholar
Stewartson, K. 1969 On the flow near the trailing edge of a flat plate. Mathematika 16 (1), 106–121.Google Scholar
Stewartson, K. & Williams, P. G. 1969 Self-induced separation. Proc. R. Soc. Lond. A 312, 181–206.Google Scholar
Sychev, V. V., Ruban, A. I., Sychev, Vic. V. & Korolev, G. L. 1998 Asymptotic Theory of Separated Flows. Cambridge University Press.CrossRefGoogle Scholar
Sykes, R. I. 1978 Stratification effects in boundary layer flow over hills. Proc. R. Soc. A 361, 225–243.Google Scholar
Sykes, R. I. 1980 On three-dimensional boundary layer flow over surface irregularities. Proc. R. Soc. A 373, 311–329.Google Scholar
Wang, K. C. 1971 On the determination of the zones of influence and dependence for three-dimensional boundary-layer equations. J. Fluid Mech. 48, 397–404.CrossRefGoogle Scholar