Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T03:45:34.528Z Has data issue: false hasContentIssue false
  • English
  • Français

General solutions of linear poro-viscoelastic materials in spherical coordinates

Published online by Cambridge University Press:  04 August 2022

Moslem Moradi Open the ORCID record for Moslem Moradi [Opens in a new window] ,
Wenzheng Shi Open the ORCID record for Wenzheng Shi [Opens in a new window]  and
Ehssan Nazockdast Open the ORCID record for Ehssan Nazockdast [Opens in a new window]
Moslem Moradi
Affiliation:
Department of Applied Physical Sciences, University of North Carolina at Chapel Hill, Chapel Hill, NC 27599-3250, USA
Wenzheng Shi
Affiliation:
Department of Applied Physical Sciences, University of North Carolina at Chapel Hill, Chapel Hill, NC 27599-3250, USA
Ehssan Nazockdast*
Affiliation:
Department of Applied Physical Sciences, University of North Carolina at Chapel Hill, Chapel Hill, NC 27599-3250, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

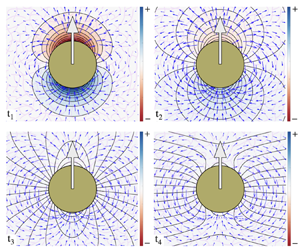
The cell cytoskeleton is a dynamic assembly of semi-flexible filaments and motor proteins. The cytoskeleton mechanics is a determining factor in many cellular processes, including cell division, cell motility and migration, mechanotransduction and intracellular transport. Mechanical properties of the cell, which are determined partly by its cytoskeleton, are also used as biomarkers for disease diagnosis and cell sorting. Experimental studies suggest that in whole cell scale, the cell cytoskeleton and its permeating cytosol may be modelled as a two-phase poro-viscoelastic (PVE) material composed of a viscoelastic (VE) network permeated by a viscous cytosol. We present the first general solution to this two-phase system in spherical coordinates, where we assume that both the fluid and network phases are in their linear response regime. Specifically, we use generalized linear incompressible and compressible VE constitutive equations to describe the stress in the fluid and network phases, respectively. We assume a constant permeability that couples the fluid and network displacements. We use these general solutions to study the motion of a rigid sphere moving under a constant force inside a two-phase system, composed of a linear elastic network and a Newtonian fluid. It is shown that the network compressibility introduces a slow relaxation of the sphere and non-monotonic network displacements with time along the direction of the applied force. Our results can be applied to particle-tracking microrheology to differentiate between PVE and VE materials, and to measure the fluid permeability as well as VE properties of the fluid and the network phases.

JFM classification

Low-Reynolds-number flows: Porous media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 946 , 10 September 2022 , A22
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abate, J. & Whitt, W. 1992 The Fourier-series method for inverting transforms of probability distributions. Queueing Syst. 10 (1), 5–87.CrossRefGoogle Scholar
Arfken, G.B. & Weber, H.J. 1999 Mathematical Methods for Physicists. American Association of Physics Teachers.Google Scholar
Aridor, M. & Hannan, L.A. 2000 Traffic jam: a compendium of human diseases that affect intracellular transport processes. Traffic 1 (11), 836–851.CrossRefGoogle ScholarPubMed
Aridor, M. & Hannan, L.A. 2002 Traffic jams II: an update of diseases of intracellular transport. Traffic 3 (11), 781–790.CrossRefGoogle ScholarPubMed
Banerjee, S. & Marchetti, M.C. 2011 Instabilities and oscillations in isotropic active gels. Soft Matt. 7 (2), 463–473.CrossRefGoogle Scholar
Beicker, K., O'Brien, E.T., Falvo, M.R. & Superfine, R. 2018 Vertical light sheet enhanced side-view imaging for AFM cell mechanics studies. Sci. Rep. 8 (1), 1–12.CrossRefGoogle ScholarPubMed
Ben-Menahem, A. & Singh, S.J. 1968 Eigenvector expansions of Green's dyads with applications to geophysical theory. Geophys. J. Intl 16 (4), 417–452.CrossRefGoogle Scholar
Biot, M.A. 1941 General theory of three-dimensional consolidation. J. Appl. Phys. 12 (2), 155–164.CrossRefGoogle Scholar
Biot, M.A. 1955 Theory of elasticity and consolidation for a porous anisotropic solid. J. Appl. Phys. 26 (2), 182–185.CrossRefGoogle Scholar
Charras, G.T., Coughlin, M., Mitchison, T.J. & Mahadevan, L. 2008 Life and times of a cellular bleb. Biophys. J. 94 (5), 1836–1853.CrossRefGoogle ScholarPubMed
Charras, G.T., Mitchison, T.J. & Mahadevan, L. 2009 Animal cell hydraulics. J. Cell Sci. 122 (18), 3233–3241.CrossRefGoogle ScholarPubMed
Charras, G.T., Yarrow, J.C., Horton, M.A., Mahadevan, L. & Mitchison, T.J. 2005 Non-equilibration of hydrostatic pressure in blebbing cells. Nature 435 (7040), 365–369.CrossRefGoogle ScholarPubMed
Cheng, A.H.-D. 2016 Poroelasticity, Vol. 27. Springer.CrossRefGoogle Scholar
Copos, C.A. & Guy, R.D. 2018 A porous viscoelastic model for the cell cytoskeleton. ANZIAM J. 59 (4), 472–498.Google Scholar
Darling, E.M. & Di Carlo, D. 2015 High-throughput assessment of cellular mechanical properties. Annu. Rev. Biomed. Engng 17, 35–62.CrossRefGoogle ScholarPubMed
Delarue, M., et al. 2018 mTORC1 controls phase separation and the biophysical properties of the cytoplasm by tuning crowding. Cell 174 (2), 338–349.CrossRefGoogle ScholarPubMed
Detournay, E. & Cheng, A.H.-D. 1993 Fundamentals of poroelasticity. In Analysis and Design Methods (ed. C. Fairhurst), pp. 113–171. Elsevier.CrossRefGoogle Scholar
Di Carlo, D. 2012 A mechanical biomarker of cell state in medicine. J. Lab. Autom. 17 (1), 32–42.CrossRefGoogle ScholarPubMed
Diamant, H. 2015 Response of a polymer network to the motion of a rigid sphere. Eur. Phys. J. E 38 (5), 1–11.CrossRefGoogle Scholar
Doi, M. 2009 Gel dynamics. J. Phys. Soc. Japan 78 (5), 052001.CrossRefGoogle Scholar
Feng, J.J. & Young, Y.-N. 2020 Boundary conditions at a gel–fluid interface. Phys. Rev. Fluids 5 (12), 124304.CrossRefGoogle Scholar
Fiore, A.M. & Swan, J.W. 2019 Fast Stokesian dynamics. J. Fluid Mech. 878, 544–597.CrossRefGoogle Scholar
Fletcher, D.A. & Mullins, R.D. 2010 Cell mechanics and the cytoskeleton. Nature 463 (7280), 485–492.CrossRefGoogle ScholarPubMed
Fu, H.C., Shenoy, V.B. & Powers, T.R. 2008 Role of slip between a probe particle and a gel in microrheology. Phys. Rev. E 78 (6), 061503.CrossRefGoogle Scholar
Fu, H.C., Shenoy, V.B. & Powers, T.R. 2010 Low-Reynolds-number swimming in gels. Europhys. Lett. 91 (2), 24002.CrossRefGoogle Scholar
Gurtin, M.E. 1973 The linear theory of elasticity. In Linear Theories of Elasticity and Thermoelasticity (ed. C. Truesdell), pp. 1–295. Springer.CrossRefGoogle Scholar
Haase, K. & Pelling, A.E. 2015 Investigating cell mechanics with atomic force microscopy. J. R. Soc. Interface 12 (104), 20140970.CrossRefGoogle ScholarPubMed
Hao, Y., Cheng, S., Tanaka, Y., Hosokawa, Y., Yalikun, Y. & Li, M. 2020 Mechanical properties of single cells: measurement methods and applications. Biotechnol. Adv. 45, 107648.CrossRefGoogle ScholarPubMed
Happel, J. & Brenner, H. 2012 Low Reynolds Number Hydrodynamics: With Special Applications to Particulate Media, Vol. 1. Springer Science & Business Media.Google Scholar
Hobson, C.M., Falvo, M.R. & Superfine, R. 2021 A survey of physical methods for studying nuclear mechanics and mechanobiology. APL Bioengng 5 (4), 041508.CrossRefGoogle ScholarPubMed
Howard, J. 2001 Mechanics of Motor Proteins and the Cytoskeleton. Sinauer Associates.Google Scholar
Irianto, J., Pfeifer, C.R., Bennett, R.R., Xia, Y., Ivanovska, I.L., Liu, A.J., Greenberg, R.A. & Discher, D.E. 2016 Nuclear constriction segregates mobile nuclear proteins away from chromatin. Mol. Biol. Cell 27 (25), 4011–4020.CrossRefGoogle ScholarPubMed
Kim, S. & Karrila, S.J. 2013 Microhydrodynamics: Principles and Selected Applications. Courier Corporation.Google Scholar
Levine, A.J. & Lubensky, T.C. 2000 One- and two-particle microrheology. Phys. Rev. Lett. 85 (8), 1774.CrossRefGoogle ScholarPubMed
Levine, A.J. & Lubensky, T.C. 2001 Response function of a sphere in a viscoelastic two-fluid medium. Phys. Rev. E 63 (4), 041510.CrossRefGoogle Scholar
MacKintosh, F.C. & Levine, A.J. 2008 Nonequilibrium mechanics and dynamics of motor-activated gels. Phys. Rev. Lett. 100 (1), 018104.CrossRefGoogle ScholarPubMed
Mason, T.G. & Weitz, D.A. 1995 Optical measurements of frequency-dependent linear viscoelastic moduli of complex fluids. Phys. Rev. Lett. 74 (7), 1250.CrossRefGoogle ScholarPubMed
Mitchison, T.J., Charras, G.T. & Mahadevan, L. 2008 Implications of a poroelastic cytoplasm for the dynamics of animal cell shape. Semin. Cell Dev. Biol. 19, 215–223.CrossRefGoogle ScholarPubMed
Moeendarbary, E., Valon, L., Fritzsche, M., Harris, A.R., Moulding, D.A., Thrasher, A.J., Stride, E., Mahadevan, L. & Charras, G.T. 2013 The cytoplasm of living cells behaves as a poroelastic material. Nat. Mater. 12 (3), 253.CrossRefGoogle ScholarPubMed
Mogilner, A. & Manhart, A. 2018 Intracellular fluid mechanics: coupling cytoplasmic flow with active cytoskeletal gel. Annu. Rev. Fluid Mech. 50, 347–370.CrossRefGoogle Scholar
Mogre, S.S., Brown, A.I. & Koslover, E.F. 2020 Getting around the cell: physical transport in the intracellular world. Phys. Biol. 17 (6), 061003.CrossRefGoogle Scholar
Morse, P.M. & Feshbach, H. 1953 Methods of Theoretical Physics. McGraw-Hill.Google Scholar
Needleman, D. & Dogic, Z. 2017 Active matter at the interface between materials science and cell biology. Nat. Rev. Mater. 2 (9), 1–14.CrossRefGoogle Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.CrossRefGoogle Scholar
Prost, J., Jülicher, F. & Joanny, J.-F. 2015 Active gel physics. Nat. Phys. 11 (2), 111–117.CrossRefGoogle Scholar
Rosenbluth, M.J., Crow, A., Shaevitz, J.W. & Fletcher, D.A. 2008 Slow stress propagation in adherent cells. Biophys. J. 95 (12), 6052–6059.CrossRefGoogle ScholarPubMed
Sangani, A.S. & Acrivos, A. 1982 Slow flow past periodic arrays of cylinders with application to heat transfer. Intl J. Multiphase Flow 8 (3), 193–206.CrossRefGoogle Scholar
Shelley, M.J. 2016 The dynamics of microtubule/motor-protein assemblies in biology and physics. Annu. Rev. Fluid Mech. 48, 487–506.CrossRefGoogle Scholar
Sonn-Segev, A., Bernheim-Groswasser, A., Diamant, H. & Roichman, Y. 2014 Viscoelastic response of a complex fluid at intermediate distances. Phys. Rev. Lett. 112 (8), 088301.CrossRefGoogle Scholar
Squires, T.M. & Mason, T.G. 2010 Fluid mechanics of microrheology. Annu. Rev. Fluid Mech. 42, 413–438.CrossRefGoogle Scholar
Strychalski, W. 2021 3D computational modeling of bleb initiation dynamics. Front. Phys. 9, 775465.CrossRefGoogle Scholar
Strychalski, W., Copos, C.A., Lewis, O.L. & Guy, R.D. 2015 A poroelastic immersed boundary method with applications to cell biology. J. Comput. Phys. 282, 77–97.CrossRefGoogle Scholar
Strychalski, W. & Guy, R.D. 2016 Intracellular pressure dynamics in blebbing cells. Biophys. J. 110 (5), 1168–1179.CrossRefGoogle ScholarPubMed
Weihs, D., Mason, T.G. & Teitell, M.A. 2006 Bio-microrheology: a frontier in microrheology. Biophys. J. 91 (11), 4296–4305.CrossRefGoogle ScholarPubMed