Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Orchini, Alessandro
Rigas, Georgios
and
Juniper, Matthew P.
2016.
Weakly nonlinear analysis of thermoacoustic bifurcations in the Rijke tube.
Journal of Fluid Mechanics,
Vol. 805,
Issue. ,
p.
523.
Orchini, Alessandro
and
Juniper, Matthew P.
2016.
Linear stability and adjoint sensitivity analysis of thermoacoustic networks with premixed flames.
Combustion and Flame,
Vol. 165,
Issue. ,
p.
97.
Orchini, Alessandro
and
Juniper, Matthew P.
2016.
Flame Double Input Describing Function analysis.
Combustion and Flame,
Vol. 171,
Issue. ,
p.
87.
Singaravelu, Balasubramanian
and
Mariappan, Sathesh
2016.
Stability analysis of thermoacoustic interactions in vortex shedding combustors using Poincaré map.
Journal of Fluid Mechanics,
Vol. 801,
Issue. ,
p.
597.
Jaensch, Stefan
and
Polifke, Wolfgang
2017.
Uncertainty encountered when modelling self-excited thermoacoustic oscillations with artificial neural networks.
International Journal of Spray and Combustion Dynamics,
Vol. 9,
Issue. 4,
p.
367.
Jaensch, S.
Merk, M.
Gopalakrishnan, E.A.
Bomberg, S.
Emmert, T.
Sujith, R.I.
and
Polifke, W.
2017.
Hybrid CFD/low-order modeling of nonlinear thermoacoustic oscillations.
Proceedings of the Combustion Institute,
Vol. 36,
Issue. 3,
p.
3827.
Kashinath, Karthik
Li, Larry K. B.
and
Juniper, Matthew P.
2018.
Forced synchronization of periodic and aperiodic thermoacoustic oscillations: lock-in, bifurcations and open-loop control.
Journal of Fluid Mechanics,
Vol. 838,
Issue. ,
p.
690.
Ghirardo, Giulio
Juniper, Matthew P.
and
Bothien, Mirko R.
2018.
The effect of the flame phase on thermoacoustic instabilities.
Combustion and Flame,
Vol. 187,
Issue. ,
p.
165.
Orchini, Alessandro
Mensah, Georg A.
and
Moeck, Jonas P.
2019.
Effects of Nonlinear Modal Interactions on the Thermoacoustic Stability of Annular Combustors.
Journal of Engineering for Gas Turbines and Power,
Vol. 141,
Issue. 2,
Zhang, Zhengyuan
Zhang, Jiazhong
Fan, Pengyu
and
He, Yongsen
2019.
Devil’s staircases in a thermoacoustic system with sinusoidal excitations.
The European Physical Journal Special Topics,
Vol. 228,
Issue. 9,
p.
1891.
Huhn, Francisco
and
Magri, Luca
2020.
Optimisation of chaotically perturbed acoustic limit cycles.
Nonlinear Dynamics,
Vol. 100,
Issue. 2,
p.
1641.
Xing, Xue
Xu, Qi
Du, Jingtao
Cheng, Li
and
Liu, Zhigang
2020.
A Modified Fourier Series Solution for a Thermo-Acoustic Tube with Arbitrary Impedance Boundaries.
International Journal of Applied Mechanics,
Vol. 12,
Issue. 05,
p.
2050047.
Meindl, M.
Albayrak, A.
and
Polifke, W.
2020.
A state-space formulation of a discontinuous Galerkin method for thermoacoustic stability analysis.
Journal of Sound and Vibration,
Vol. 481,
Issue. ,
p.
115431.
Guan, Yu
Gupta, Vikrant
and
Li, Larry K. B.
2020.
Intermittency route to self-excited chaotic thermoacoustic oscillations.
Journal of Fluid Mechanics,
Vol. 894,
Issue. ,
Huhn, Francisco
and
Magri, Luca
2020.
Stability, sensitivity and optimisation of chaotic acoustic oscillations.
Journal of Fluid Mechanics,
Vol. 882,
Issue. ,
Polifke, Wolfgang
2020.
Modeling and analysis of premixed flame dynamics by means of distributed time delays.
Progress in Energy and Combustion Science,
Vol. 79,
Issue. ,
p.
100845.
Orchini, Alessandro
Silva, Camilo F.
Mensah, Georg A.
and
Moeck, Jonas P.
2020.
Thermoacoustic modes of intrinsic and acoustic origin and their interplay with exceptional points.
Combustion and Flame,
Vol. 211,
Issue. ,
p.
83.
von Saldern, Jakob G. R.
Orchini, Alessandro
and
Moeck, Jonas P.
2021.
Analysis of Thermoacoustic Modes in Can-Annular Combustors Using Effective Bloch-Type Boundary Conditions.
Journal of Engineering for Gas Turbines and Power,
Vol. 143,
Issue. 7,
Sujith, R. I.
and
Pawar, Samadhan A.
2021.
Thermoacoustic Instability.
p.
1.
Gant, Francesco
Ghirardo, Giulio
and
Bothien, Mirko R.
2021.
On the importance of time delay and noise in thermoacoustic modeling.
Journal of Sound and Vibration,
Vol. 501,
Issue. ,
p.
116067.
 $G$-equation, with a state-space realization of the linearized acoustic equations. However, so far the
$G$-equation, with a state-space realization of the linearized acoustic equations. However, so far the 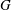 $G$-equation has been coupled only with straight ducts with uniform mean acoustic properties, which is a simplistic configuration. In this study, we incorporate a wave-based model of the acoustic network, containing area and temperature variations and frequency-dependent boundary conditions. We cast the linear acoustics into state-space form using a different approach from that in the existing literature. We then use this state-space form to investigate the stability of the thermoacoustic system, both in the frequency and time domains, using the flame position as a control parameter. We observe frequency-locked, quasiperiodic and chaotic oscillations. We identify the location of Neimark–Sacker bifurcations with Floquet theory. We also find the Ruelle–Takens–Newhouse route to chaos with nonlinear time series analysis techniques. We highlight important differences between the nonlinear response predicted by the frequency domain and the time domain methods. This reveals deficiencies with the frequency domain technique, which is commonly used in academic and industrial studies of thermoacoustic systems. We then demonstrate a more accurate approach based on continuation analysis applied to time domain techniques.
$G$-equation has been coupled only with straight ducts with uniform mean acoustic properties, which is a simplistic configuration. In this study, we incorporate a wave-based model of the acoustic network, containing area and temperature variations and frequency-dependent boundary conditions. We cast the linear acoustics into state-space form using a different approach from that in the existing literature. We then use this state-space form to investigate the stability of the thermoacoustic system, both in the frequency and time domains, using the flame position as a control parameter. We observe frequency-locked, quasiperiodic and chaotic oscillations. We identify the location of Neimark–Sacker bifurcations with Floquet theory. We also find the Ruelle–Takens–Newhouse route to chaos with nonlinear time series analysis techniques. We highlight important differences between the nonlinear response predicted by the frequency domain and the time domain methods. This reveals deficiencies with the frequency domain technique, which is commonly used in academic and industrial studies of thermoacoustic systems. We then demonstrate a more accurate approach based on continuation analysis applied to time domain techniques.


