Article contents
The enhancement of viscous fingering with bidisperse particle suspension
Published online by Cambridge University Press: 07 December 2018
Abstract
Viscous fingering is observed experimentally when a bidisperse suspension displaces air inside a Hele-Shaw cell, despite the stabilising viscosity ratio between the invading (suspension) and defending (air) phases. Careful experiments are carried out to characterise this instability by either systematically varying the large-particle concentrations  $\unicode[STIX]{x1D719}_{l0}$ at constant total concentrations
$\unicode[STIX]{x1D719}_{l0}$ at constant total concentrations 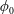 $\unicode[STIX]{x1D719}_{0}$, or changing
$\unicode[STIX]{x1D719}_{0}$, or changing  $\unicode[STIX]{x1D719}_{0}$ with fixed
$\unicode[STIX]{x1D719}_{0}$ with fixed 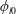 $\unicode[STIX]{x1D719}_{l0}$. Leading to the instability, we observe that larger particles consistently enrich the fluid–fluid interface at a faster rate than small particles. This size-dependent enrichment of the interface leads to an earlier onset of the fingering instability for bidisperse suspensions, compared to their monodisperse counterpart of all small particles. In particular, even the small presence of large particles is shown to effectively lower the total particle concentration needed for fingering, compared to the all-small-particle case. We hypothesise that the key mechanism behind this enhanced viscous fingering is the size-dependent nature of shear-induced migration of particles far upstream from the interface. A reduced equilibrium model is derived based on the modified suspension balance model to verify this hypothesis, in reasonable agreement with experiments.
$\unicode[STIX]{x1D719}_{l0}$. Leading to the instability, we observe that larger particles consistently enrich the fluid–fluid interface at a faster rate than small particles. This size-dependent enrichment of the interface leads to an earlier onset of the fingering instability for bidisperse suspensions, compared to their monodisperse counterpart of all small particles. In particular, even the small presence of large particles is shown to effectively lower the total particle concentration needed for fingering, compared to the all-small-particle case. We hypothesise that the key mechanism behind this enhanced viscous fingering is the size-dependent nature of shear-induced migration of particles far upstream from the interface. A reduced equilibrium model is derived based on the modified suspension balance model to verify this hypothesis, in reasonable agreement with experiments.
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 10
- Cited by


