Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-01-10T18:54:40.029Z Has data issue: false hasContentIssue false
  • English
  • Français

Energy transfer structures associated with large-scale motions in a turbulent boundary layer

Published online by Cambridge University Press:  13 November 2020

Wenkang Wang Open the ORCID record for Wenkang Wang [Opens in a new window] ,
Chong Pan  and
Jinjun Wang Open the ORCID record for Jinjun Wang [Opens in a new window]
Wenkang Wang
Affiliation:
Institute of Fluid Mechanics, Beihang University, Beijing, 100191, PR China
Chong Pan*
Affiliation:
Institute of Fluid Mechanics, Beihang University, Beijing, 100191, PR China Aircraft and Propulsion Laboratory, Ningbo Institute of Technology, Beihang University, Ningbo, 315800, PR China
Jinjun Wang
Affiliation:
Institute of Fluid Mechanics, Beihang University, Beijing, 100191, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

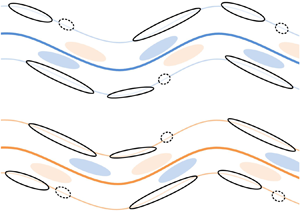
The role of large-scale motions (LSMs) in energy transfer is investigated by analysing wall-parallel velocity fields at low-to-moderate Reynolds number ($Re_{\tau }=1200\text {--}3500$), which are obtained via a two-dimensional (2-D) particle image velocimetry measurement with large field-of-view. Two types of energy flux, i.e. local interscale energy flux and in-plane spatial energy flux are inspected in detail. Targeting the energy transfer in large-scale regime, an anisotropic filter is designed based on the zero-crossing scale boundary in a 2-D energy transfer spectrum, across which the net energy flux is the maximum. This ‘optimal’ energy flux boundary separates the scale space into an energy donating large-scale part and an energy receiving small-scale one. The crossover energy flux, as well as the associated flow field structures, are studied by conditional statistics and linear stochastic estimation, in which the statistical spanwise symmetry is deliberately broken by designing special velocity gradient conditions for event probing. A strong connection between large-scale energy flux events and LSMs are found. Namely, forward scatter events have higher probability to reside on the wavy flank of low-momentum LSMs, if compared with the scenario of being clamped in the middle of two streamwise-aligned high- and low-momentum LSMs (Natrajan & Christensen, Phys. Fluids, vol. 18, issue 6, 2006, pp. 299–325). Meanwhile, pairs of positive and negative spatial transfer events tend to locate inside LSMs. It is thus argued that the meandering nature of LSMs, which forms the necessary velocity gradient, might play a determining role in the process of large-scale energy transfer. The spatial correlation between them is then schematized in a conceptual model, which explains most of the present observations.

JFM classification

Turbulent Flows: Turbulent boundary layers Boundary Layers: Boundary layer structure
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 906 , 10 January 2021 , A14
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adrian, R. J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19 (4), 041301.CrossRefGoogle Scholar
Adrian, R. J. & Moin, P. 1988 Stochastic estimation of organized turbulent structure: homogeneous shear flow. J. Fluid Mech. 190, 531–559.CrossRefGoogle Scholar
Agostini, L. & Leschziner, M. 2017 Spectral analysis of near-wall turbulence in channel flow at ${\mathrm {Re}}_{{\tau }}=4200$ with emphasis on the attached-eddy hypothesis. Phys. Rev. Fluids 2, 014603.CrossRefGoogle Scholar
del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.CrossRefGoogle Scholar
Alexakisa, A. & Biferale, L. 2018 Cascades and transitions in turbulent flows. Phys. Rep. 767–769, 1–101.CrossRefGoogle Scholar
Baars, W. J., Hutchins, N. & Marusic, I. 2017 Self-similarity of wall-attached turbulence in boundary layers. J. Fluid Mech. 823, R2.CrossRefGoogle Scholar
Biferale, L. 2003 Shell models of energy cascade in turbulence. Annu. Rev. Fluid Mech. 35 (1), 441–468.CrossRefGoogle Scholar
Boffetta, G. & Ecke, R. E. 2012 Two-dimensional turbulence. Annu. Rev. Fluid Mech. 44 (1), 427–451.CrossRefGoogle Scholar
Cardesa, J. I., Vela-Martín, A. & Jiménez, J. 2017 The turbulent cascade in five dimensions. Science 357 (6353), 782–784.CrossRefGoogle ScholarPubMed
Carper, M. A. & Porté-Agel, F. 2004 The role of coherent structures in subfilter-scale dissipation of turbulence measured in the atmospheric surface layer. J. Turbul. 5, 32–32.CrossRefGoogle Scholar
Chandran, D., Baidya, R., Monty, J. P. & Marusic, I. 2017 Two-dimensional energy spectra in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 826, R1.CrossRefGoogle Scholar
Chauhan, K. A., Monkewitz, P. A. & Nagib, H. M. 2009 Criteria for assessing experiments in zero pressure gradient boundary layers. Fluid Dyn. Res. 41 (2), 021404.CrossRefGoogle Scholar
Cho, M., Hwang, Y. & Choi, H. 2018 Scale interactions and spectral energy transfer in turbulent channel flow. J. Fluid Mech. 854, 474–504.CrossRefGoogle Scholar
Cimarelli, A., De Angelis, E. & Casciola, C. M. 2013 Paths of energy in turbulent channel flows. J. Fluid Mech. 715, 436–451.CrossRefGoogle Scholar
Cimarelli, A., De Angelis, E., Jiménez, J. & Casciola, C. M. 2016 Cascades and wall-normal fluxes in turbulent channel flows. J. Fluid Mech. 796, 417–436.CrossRefGoogle Scholar
Cimarelli, A., De Angelis, E., Schlatter, P., Brethouwer, G., Talamelli, A. & Casciola, C. M. 2015 Sources and fluxes of scale energy in the overlap layer of wall turbulence. J. Fluid Mech. 771, 407–423.CrossRefGoogle Scholar
Cuvier, C., Srinath, S., Stanislas, M., Foucaut, J.-M., Laval, J.-P., Kähler, C. J., Hain, R., Scharnowski, S., Schröder, A., Geisler, R., et al. 2017 Extensive characterisation of a high Reynolds number decelerating boundary layer using advanced optical metrology. J. Turbul. 18 (10), 929–972.CrossRefGoogle Scholar
Dong, S. W., Huang, Y. X., Yuan, X. & Lozano-Durán, A. 2020 The coherent structure of the kinetic energy transfer in shear turbulence. J. Fluid Mech. 892, A22.CrossRefGoogle Scholar
Dunn, D. C. & Morrison, J. F. 2003 Anisotropy and energy flux in wall turbulence. J. Fluid Mech. 491, 353–378.CrossRefGoogle Scholar
Fang, L. & Ouellette, N. T. 2016 Advection and the efficiency of spectral energy transfer in two-dimensional turbulence. Phys. Rev. Lett. 117 (10), 104501.CrossRefGoogle ScholarPubMed
de Giovanetti, M., Sung, H. J. & Hwang, Y. 2017 Streak instability in turbulent channel flow: the seeding mechanism of large-scale motions. J. Fluid Mech. 832, 483–513.CrossRefGoogle Scholar
Härtle, C., Klesier, L., Unger, F. & Friedrich, R. 1994 Subgrid-scale energy transfer in the near-wall region of turbulent flows. Phys. Fluids 6 (9), 3130–3143.CrossRefGoogle Scholar
Hong, J., Joseph, K., Charles, M. & Schultz, M. P. 2012 Coherent structures and associated subgrid-scale energy transfer in a rough-wall turbulent channel flow. J. Fluid Mech. 712 (6), 92–128.CrossRefGoogle Scholar
Hong, J., Katz, J. & Schultz, M. P. 2011 Near-wall turbulence statistics and flow structures over three-dimensional roughness in a turbulent channel flow. J. Fluid Mech. 667, 1–37.CrossRefGoogle Scholar
Hultmark, M., Vallikivi, M., Bailey, S. C. C. & Smits, A. J. 2013 Logarithmic scaling of turbulence in smooth- and rough-wall pipe flow. J. Fluid Mech. 728, 376–395.CrossRefGoogle Scholar
Hutchins, N. & Marusic, I. 2007 Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.CrossRefGoogle Scholar
Hwang, Y. & Cossu, C. 2010 Self-sustained process at large scales in turbulent channel flow. Phys. Rev. Lett. 105, 044505.CrossRefGoogle ScholarPubMed
Hwang, J. & Sung, H. J. 2018 Wall-attached structures of velocity fluctuations in a turbulent boundary layer. J. Fluid Mech. 856, 958–983.CrossRefGoogle Scholar
Jiménez, J. 2018 Coherent structures in wall-bounded turbulence. J. Fluid Mech. 842, P1.CrossRefGoogle Scholar
Jiménez, J. 2012 Cascades in wall-bounded turbulence. Annu. Rev. Fluid Mech. 44, 27–45.CrossRefGoogle Scholar
Kevin, K., Monty, J. & Hutchins, N. 2019 The meandering behaviour of large-scale structures in turbulent boundary layers. J. Fluid Mech. 865, R1.CrossRefGoogle Scholar
Klewicki, J., Fife, P. & Wei, T. 2009 On the logarithmic mean profile. J. Fluid Mech. 638, 73–93.CrossRefGoogle Scholar
Kolmogorov, A. N. 1941 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. C. R. Acad. Sci. URSS 30, 301–305.Google Scholar
Kraichnan, R. H. 1976 Eddy viscosity in two and three dimensions. J. Atmos. Sci. 33 (33), 1521–1536.2.0.CO;2>CrossRefGoogle Scholar
Lee, M. & Moser, R. D. 2019 Spectral analysis of the budget equation in turbulent channel flows at high Reynolds number. J. Fluid Mech. 860, 886–938.CrossRefGoogle Scholar
Leslie, D. C. & Quarini, G. L. 1979 The application of turbulence theory to the formulation of subgrid modelling procedures. J. Fluid Mech. 91 (1), 65–91.CrossRefGoogle Scholar
Liao, Y. & Ouellette, N. 2013 Spatial structure of spectral transport in two-dimensional flow. J. Fluid Mech. 725 (3), 281–298.CrossRefGoogle Scholar
Lin, C.-L. 1999 Near-grid-scale energy transfer and coherent structures in the convective planetary boundary layer. Phys. Fluids 11 (11), 3482–3494.CrossRefGoogle Scholar
Marusic, I., Mathis, R. & Hutchins, N. 2010 Predictive model for wall-bounded turbulent flow. Science 329 (5988), 193–196.CrossRefGoogle ScholarPubMed
Marusic, I. & Monty, J. P. 2019 Attached eddy model of wall turbulence. Annu. Rev. Fluid Mech. 51, 49–74.CrossRefGoogle Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, R3.CrossRefGoogle Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2011 A predictive inner–outer model for streamwise turbulence statistics in wall-bounded flows. J. Fluid Mech. 681, 537–566.CrossRefGoogle Scholar
McKeon, B. J. 2017 The engine behind (wall) turbulence: perspectives on scale interactions. J. Fluid Mech. 817, P1.CrossRefGoogle Scholar
Mizuno, Y. 2016 Spectra of energy transport in turbulent channel flows for moderate Reynolds numbers. J. Fluid Mech. 805, 171–187.CrossRefGoogle Scholar
Monin, A. S. & Yaglom, A. M. 1975 Statistical Fluid Mechanics: Mechanics of Turbulence, vol. 2. MIT Press.Google Scholar
Motoori, Y. & Goto, S. 2019 Generation mechanism of a hierarchy of vortices in a turbulent boundary layer. J. Fluid Mech. 865, 1085–1109.CrossRefGoogle Scholar
Natrajan, V. K. & Christensen, K. T. 2006 The role of coherent structures in subgrid-scale energy transfer within the log layer of wall turbulence. Phys. Fluids 18 (6), 299–325.CrossRefGoogle Scholar
Osaka, H., Kameda, T. & Mochizuki, S. 1998 Re-examination of the Reynolds-number-effect on the mean flow quantities in a smooth wall turbulent boundary layer. JSME Intl J. 41 (1), 123–129.CrossRefGoogle Scholar
Pan, C., Xue, D., Xu, Y., Wang, J. J. & Wei, R. J. 2015 Evaluating the accuracy performance of Lucas–Kanade algorithm in the circumstance of PIV application. Sci. China Phys. Mech. Astron. 58 (10), 1–16.CrossRefGoogle Scholar
Piomelli, U., Cabot, W. H., Moin, P. & Lee, S. 1991 Subgrid-scale backscatter in turbulent and transitional flows. Phys. Fluids A 3 (7), 1766–1771.CrossRefGoogle Scholar
Piomelli, U., Yu, Y. & Adrian, R. J. 1996 Subgrid-scale energy transfer and near-wall turbulence structure. Phys. Fluids 8 (1), 215–224.CrossRefGoogle Scholar
Schlatter, P. & Örlü, R. 2012 Turbulent boundary layers at moderate Reynolds numbers: inflow length and tripping effects. J. Fluid Mech. 710, 5–34.CrossRefGoogle Scholar
Sillero, J. A., Jiménez, J. & Moser, R. D. 2013 One-point statistics for turbulent wall-bounded flows at Reynolds numbers up to $\delta ^{+}\approx 2000$. Phys. Fluids 25 (10), 105102.CrossRefGoogle Scholar
Sillero, J. A., Jiménez, J. & Moser, R. D. 2014 Two-point statistics for turbulent boundary layers and channels at Reynolds numbers up to $\delta ^{+}\approx 2000$. Phys. Fluids 26 (10), 105109.CrossRefGoogle Scholar
de Silva, C. M., Chandran, D., Baidya, R., Hutchins, N. & Marusic, I. 2020 Periodicity of large-scale coherence in turbulent boundary layers. Intl J. Heat Fluid Flow 83, 108575.CrossRefGoogle Scholar
de Silva, C. M., Gnanamanickam, E. P., Atkinson, C., Buchmann, N. A., Hutchins, N., Soria, J. & Marusic, I. 2014 High spatial range velocity measurements in a high Reynolds number turbulent boundary layer. Phys. Fluids 26 (2), 025117.CrossRefGoogle Scholar
de Silva, C. M., Kevin, K., Baidya, R., Hutchins, N. & Marusic, I. 2018 Large coherence of spanwise velocity in turbulent boundary layers. J. Fluid Mech. 847, 161–185.CrossRefGoogle Scholar
Smits, A. J., Mckeon, B. J. & Marusic, I. 2011 High-Reynolds number wall turbulence. Annu. Rev. Fluid Mech. 43, 353–375.CrossRefGoogle Scholar
Stanislas, M., Perret, L. & Foucaut, J.-M. 2008 Vortical structures in the turbulent boundary layer: a possible route to a universal representation. J. Fluid Mech. 602, 327–382.CrossRefGoogle Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.CrossRefGoogle Scholar
Tomkins, C. D. & Adrian, R. J. 2003 Spanwise structure and scale growth in turbulent boundary layers. J. Fluid Mech. 490, 37–74.CrossRefGoogle Scholar
Wang, W., Pan, C. & Wang, J. 2018 b Quasi-bivariate variational mode decomposition as a tool of scale analysis in wall-bounded turbulence. Exp. Fluids 59 (1), 1.CrossRefGoogle Scholar
Wang, J., Wan, M., Chen, S. & Chen, S. 2018 a Kinetic energy transfer in compressible isotropic turbulence. J. Fluid Mech. 841, 581–613.CrossRefGoogle Scholar
Willert, C. E., Cuvier, C., Foucaut, J.-M., Klinner, J., Stanislas, M., Laval, J.-P., Srinath, S., Soria, J., Amili, O., Atkinson, C., et al. 2018 Experimental evidence of near-wall reverse flow events in a zero pressure gradient turbulent boundary layer. Expl Therm. Fluid Sci. 91, 320–328.CrossRefGoogle Scholar
Xiao, Z., Wan, M., Chen, S. & Eyink, G. L. 2009 Physical mechanism of the inverse energy cascade of two-dimensional turbulence: a numerical investigation. J. Fluid Mech. 619, 1–44.CrossRefGoogle Scholar
Zhou, Q., Huang, Y. X., Lu, Z. M., Liu, Y. L. & Ni, R. 2016 Scale-to-scale energy and enstrophy transport in two-dimensional Rayleigh–Taylor turbulence. J. Fluid Mech. 786, 294–308.CrossRefGoogle Scholar
Zhu, Y., Lee, C., Chen, X., Wu, J., Chen, S. & Gad-el-Hak, M. 2018 Newly identified principle for aerodynamic heating in hypersonic flows. J. Fluid Mech. 855, 152–180.CrossRefGoogle Scholar