Published online by Cambridge University Press: 24 September 2021
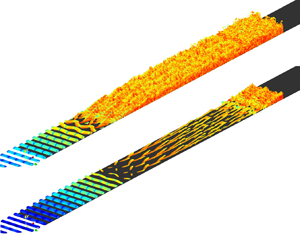
Phase effect on the modal interaction of flow instabilities is investigated for laminar-to-turbulent transition in a flat-plate boundary-layer flow. Primary and secondary three-dimensional (3-D) oblique waves at various initial phase differences between these two instability modes. Three numerical methods are used for a systematic approach for the entire transition process, i.e. before the onset of transition well into fully turbulent flow. Floquet analysis predicts the subharmonic resonance where a subharmonic mode locally resonates for a given basic flow composed of the steady laminar flow and the fundamental mode. Because Floquet analysis is limited to the resonating subharmonic mode, nonlinear parabolised stability equation analysis (PSE) is conducted with various phase shifts of the subharmonic mode with respect to the given fundamental mode. The application of PSE offers insights on the modal interaction affected by the phase difference up to the weakly nonlinear stage of transition. Large-eddy simulation (LES) is conducted for a complete transition to turbulent boundary layer because PSE becomes prohibitively expensive in the late nonlinear stage of transition. The modulation of the subharmonic resonance with the initial phase difference leads to a significant delay in the transition location up to  $\Delta Re_{x, tr} \simeq 4\times 10^5$ as predicted by the current LES. Effects of the initial phase difference on the spatial evolution of the modal shape of the subharmonic mode are further investigated. The mechanism of the phase evolution is discussed, based on current numerical results and relevant literature data.
$\Delta Re_{x, tr} \simeq 4\times 10^5$ as predicted by the current LES. Effects of the initial phase difference on the spatial evolution of the modal shape of the subharmonic mode are further investigated. The mechanism of the phase evolution is discussed, based on current numerical results and relevant literature data.
M. Kim and S. Kim contributed equally to this work.