Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gautier, N.
and
Aider, J.-L.
2013.
Control of the separated flow downstream of a backward-facing step using visual feedback.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 469,
Issue. 2160,
p.
20130404.
Gautier, Nicolas
and
Aider, Jean-Luc
2014.
Upstream open loop control of the recirculation area downstream of a backward-facing step
.
Comptes Rendus. Mécanique,
Vol. 342,
Issue. 6-7,
p.
382.
Davoust, Samuel
Jacquin, Laurent
and
Leclaire, Benjamin
2014.
New results on the structure of turbulence in a mixing layer with and without swirl.
International Journal of Heat and Fluid Flow,
Vol. 49,
Issue. ,
p.
11.
Gautier, N.
and
Aider, J.-L.
2014.
Feed-forward control of a perturbed backward-facing step flow.
Journal of Fluid Mechanics,
Vol. 759,
Issue. ,
p.
181.
Gand, F.
Brunet, V.
and
Mancel, G.
2015.
Progress in Hybrid RANS-LES Modelling.
Vol. 130,
Issue. ,
p.
141.
Gautier, N.
and
Aider, J.-L.
2015.
Frequency-lock reactive control of a separated flow enabled by visual sensors.
Experiments in Fluids,
Vol. 56,
Issue. 1,
Pan, Chong
Xue, Dong
Xu, Yang
Wang, JinJun
and
Wei, RunJie
2015.
Evaluating the accuracy performance of Lucas-Kanade algorithm in the circumstance of PIV application.
Science China Physics, Mechanics & Astronomy,
Vol. 58,
Issue. 10,
Gautier, N.
Aider, J.-L.
Duriez, T.
Noack, B. R.
Segond, M.
and
Abel, M.
2015.
Closed-loop separation control using machine learning.
Journal of Fluid Mechanics,
Vol. 770,
Issue. ,
p.
442.
Gautier, N.
and
Aider, J. L.
2015.
Real-time planar flow velocity measurements using an optical flow algorithm implemented on GPU.
Journal of Visualization,
Vol. 18,
Issue. 2,
p.
277.
Gand, F.
2016.
Investigation of turbulence development in incompressible jets with zonal detached eddy simulation (ZDES) and synthetic turbulent inflow.
International Journal of Heat and Fluid Flow,
Vol. 61,
Issue. ,
p.
425.
Beneddine, Samir
Yegavian, Robin
Sipp, Denis
and
Leclaire, Benjamin
2017.
Unsteady flow dynamics reconstruction from mean flow and point sensors: an experimental study.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
174.
Mullyadzhanov, R.
Abdurakipov, S.
and
Hanjalić, K.
2017.
Helical Structures in the Near Field of a Turbulent Pipe Jet.
Flow, Turbulence and Combustion,
Vol. 98,
Issue. 2,
p.
367.
Gand, F.
Verrière, J.
and
Deck, S.
2018.
Progress in Hybrid RANS-LES Modelling.
Vol. 137,
Issue. ,
p.
79.
Mullyadzhanov, R.
Abdurakipov, S.
and
Hanjalić, K.
2018.
Direct and Large-Eddy Simulation X.
Vol. 24,
Issue. ,
p.
553.
Varon, Eliott
Aider, Jean-Luc
Eulalie, Yoann
Edwige, Stephie
and
Gilotte, Philippe
2019.
Adaptive control of the dynamics of a fully turbulent bimodal wake using real-time PIV.
Experiments in Fluids,
Vol. 60,
Issue. 8,
Samie, Milad
Lavoie, Philippe
and
Pollard, Andrew
2020.
A scale-dependent coherence analysis of turbulent round jets including the effects of shear layer manipulation.
International Journal of Heat and Fluid Flow,
Vol. 82,
Issue. ,
p.
108524.
Alomar, Antoni
Nicole, Aurélie
Sipp, Denis
Rialland, Valérie
and
Vuillot, François
2020.
Reduced-order model of a reacting, turbulent supersonic jet based on proper orthogonal decomposition.
Theoretical and Computational Fluid Dynamics,
Vol. 34,
Issue. 1-2,
p.
49.
Kantharaju, Jahnavi
Courtier, Romain
Leclaire, Benjamin
and
Jacquin, Laurent
2020.
Interactions of large-scale structures in the near field of round jets at high Reynolds numbers.
Journal of Fluid Mechanics,
Vol. 888,
Issue. ,
Pickering, Ethan
Rigas, Georgios
Nogueira, Petrônio A. S.
Cavalieri, André V. G.
Schmidt, Oliver T.
and
Colonius, Tim
2020.
Lift-up, Kelvin–Helmholtz and Orr mechanisms in turbulent jets.
Journal of Fluid Mechanics,
Vol. 896,
Issue. ,
Nidhan, S.
Chongsiripinyo, K.
Schmidt, O. T.
and
Sarkar, S.
2020.
Spectral proper orthogonal decomposition analysis of the turbulent wake of a disk at Re = 50 000.
Physical Review Fluids,
Vol. 5,
Issue. 12,
 and low-Mach-number cylindrical jet has been investigated by means of a high-speed stereo PIV setup that provides the spatio-temporal velocity field in a transverse plane, two diameters downstream of the jet exit. Proper orthogonal decomposition (POD) and spatio-temporal correlations are used to identify some of the main dynamical features of this flow. We show that the flow is dominated by streamwise vortices whose production and spatial organization can be related to
and low-Mach-number cylindrical jet has been investigated by means of a high-speed stereo PIV setup that provides the spatio-temporal velocity field in a transverse plane, two diameters downstream of the jet exit. Proper orthogonal decomposition (POD) and spatio-temporal correlations are used to identify some of the main dynamical features of this flow. We show that the flow is dominated by streamwise vortices whose production and spatial organization can be related to 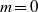 and
and  perturbations, and to the mean shear of the mixing layer. A dynamical scenario is proposed which describes this interaction, in accordance with our observations.
perturbations, and to the mean shear of the mixing layer. A dynamical scenario is proposed which describes this interaction, in accordance with our observations.

