Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-24T16:21:54.648Z Has data issue: false hasContentIssue false
  • English
  • Français

The drag on a flattened bubble moving across a plane substrate

Published online by Cambridge University Press:  01 March 2012

L. R. White  and
S. L. Carnie
L. R. White
Affiliation:
School of Mathematics and Statistics, University of South Australia, Mawson Lakes, SA 5095, Australia Department of Mathematics and Statistics, University of Melbourne, Parkville 3010, Australia
S. L. Carnie*
Affiliation:
Department of Mathematics and Statistics, University of Melbourne, Parkville 3010, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The equilibrium shape of an axisymmetric liquid drop or gas bubble in an immiscible supporting liquid held under gravity against a horizontal plane rigid surface is derived. A thin film of supporting liquid remains between the drop/bubble surface and the rigid substrate at equilibrium, of thickness 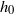determined by the balance of the disjoining pressure 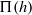between the drop and the substrate and the internal Laplace pressure of the drop/bubble. The interface is macroscopically flat around the drop axis out to a radius but matches smoothly into the outer shape of radius through a boundary layer region of width 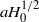where is a small parameter. The outer drop shape is determined by a balance of buoyancy forces and local Laplace pressure and is roughly spherical if 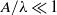, where 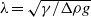is the capillary length in the interface with a logarithmic correction due to the action of the disjoining pressure across the flattened region. With the shape determined, we calculate the drag force on this flattened bubble to lowest order in the velocity as it moves across the rigid substrate using a lubrication approximation valid to terms of 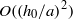as an integral over the flattened bubble surface of the hydrodynamic pressure. The lubrication theory of itself is not sufficient to determine the drag due to the divergence of that integral if the outer flow field properties are neglected. By using the known exact result for the drag force on an undistorted bubble, the drag on the flattened bubble can be computed as an integral over the lubrication region alone. We derive the drag as a series expansion in the small parameter by means of a fairly intricate boundary layer analysis. The logarithmic divergence of the translational drag with film thickness for the undistorted bubble is replaced by the stronger divergence 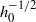to leading order for the flattened bubble case. We present explicit numerical results for the first few terms in the expansion for the case of an exponentially repulsive disjoining pressure, and analytic expressions for these terms in the limit of very short-range disjoining pressure forces. The results of this calculation are compared with recent work (Hodges, Jensen & Rallison, J. Fluid Mech., vol. 512, 2004, p. 95) where disjoining pressure is neglected and hydrodynamic pressure is balanced against buoyancy and Laplace forces. The limits of validity of this linear drag theory are also presented.

JFM classification

Interfacial Flows (free surface): Capillary flows Drops and Bubbles: Drops and Bubbles Interfacial Flows (free surface): Thin films
Type
Papers
Information
Journal of Fluid Mechanics , Volume 696 , 10 April 2012 , pp. 345 - 373
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Abramowitz, M. & Stegun, I. A. 1965 Handbook of Mathematical Functions. Dover.Google Scholar
2. Ajaev, V. S. & Homsy, G. M. 2006 Annu. Rev. Fluid Mech. 38, 277.CrossRefGoogle Scholar
3. Ajaev, V. S., Tsekov, R. & Vinogradova, O. I. 2007 Phys. Fluids 19, 061702.CrossRefGoogle Scholar
4. Ajaev, V. S., Tsekov, R. & Vinogradova, O. I. 2008 Phys. Rev. E 78, 031602.CrossRefGoogle Scholar
5. Aussillous, P. & Quere, D. 2002 Europhys. Lett. 59, 370.CrossRefGoogle Scholar
6. Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
7. Bender, C. M. & Orszag, S. A. 1978 Advanced Mathematical Methods for Scientists and Engineers. McGraw-Hill.Google Scholar
8. Bretherton, F. P. 1961 J. Fluid Mech. 10, 166.CrossRefGoogle Scholar
9. Chan, D. Y. C., Dagastine, R. R. & White, L. R. 2001 J. Colloid Interface Sci. 236, 141.CrossRefGoogle Scholar
10. Chaoui, M. & Feuillebois, F. 2003 Q. J. Mech. Appl. Maths 56, 381.CrossRefGoogle Scholar
11. Clasohm, L. Y., Connor, J. N., Vinogradova, O. I. & Horn, R. G. 2005 Langmuir 21, 8243.CrossRefGoogle Scholar
12. Dagastine, R. R., Manica, R., Carnie, S. L., Chan, D. Y. C., Stevens, G. W. & Grieser, F. 2006 Science 313, 210.CrossRefGoogle Scholar
13. Del Castillo, L. A., Ohnishi, S., White, L. R., Carnie, S. L. & Horn, R. G. 2011 J. Colloid Interface Sci. 364, 505.CrossRefGoogle Scholar
14. Denkov, N. D., Subramanian, V., Gurovich, D. & Lips, A. 2005 Colloids Surf. A 263, 129.CrossRefGoogle Scholar
15. Deryaguin, B. V. & Kussakov, M. 1939 Acta Phys. USSR 10, 251.Google Scholar
16. Goldman, A. J., Cox, R. G. & Brenner, H. 1967 Chem. Engng Sci. 22, 637.CrossRefGoogle Scholar
17. Griggs, A. J., Zinchenko, A. Z. & Davis, R. H. 2008 Intl J. Multiphase Flow 34, 408.CrossRefGoogle Scholar
18. Hinch, E. J. 1991 Perturbation Methods. Cambridge University Press.CrossRefGoogle Scholar
19. Hodges, S. R., Jensen, O. E. & Rallison, J. M. 2004 J. Fluid Mech. 512, 95.CrossRefGoogle Scholar
20. Leal, L. G. 2007 Advanced Transport Phenomena. Cambridge University Press.CrossRefGoogle Scholar
21. Legendre, D., Colin, C. & Coquard, T. 2008 Proc. R. Soc. A 366, 2233.Google Scholar
22. Manica, R., Connor, J. N., Carnie, S. L., Horn, R. G. & Chan, D. Y. C. 2007 Langmuir 23, 626.CrossRefGoogle Scholar
23. Manor, O, Vakarelski, I. U., Stevens, G. W., Grieser, F, Dagastine, R. R. & Chan, D. Y. C. 2008 Langmuir 24, 11533.CrossRefGoogle Scholar
24. Maruvada, S. R. K. & Park, C.-W. 1996 Phys. Fluids 8, 217.CrossRefGoogle Scholar
25. Meyappan, M. & Subramanian, R. S. 1987 J. Colloid Interface Sci. 115, 206.CrossRefGoogle Scholar
26. O’Neill, M. E. & Stewartson, K. 1967 J. Fluid Mech. 27, 705.CrossRefGoogle Scholar
27. Pushkarova, R. A. & Horn, R. G. 2008 Langmuir 24, 8726.CrossRefGoogle Scholar
28. Quere, D. 2005 Rep. Prog. Phys. 68, 2495.CrossRefGoogle Scholar
29. Rabaud, D., Thibault, P., Raven, J.-P., Hogon, O., lacot, E. & Marmottant, P. 2011 Phys. Fluids 23, 042003.CrossRefGoogle Scholar
30. Raufaste, C., Dollet, B., Cox, S., Jiang, Y. & Graner, F. 2007 Eur. Phys. J. E 23, 217.CrossRefGoogle Scholar
31. Teletzke, G. F., Davis, H. T. & Scriven, L. E. 1988 Rev. Phys. Appl. 23, 989.CrossRefGoogle Scholar
32. White, L. R. 1983 J. Colloid Interface Sci. 95, 286.CrossRefGoogle Scholar
33. Yarin, A. L. 2006 Annu. Rev. Fluid Mech. 38, 159.CrossRefGoogle Scholar