Article contents
Direct simulation of surface roughness signature of internal wave with deterministic energy-conservative model
Published online by Cambridge University Press: 24 March 2020
Abstract
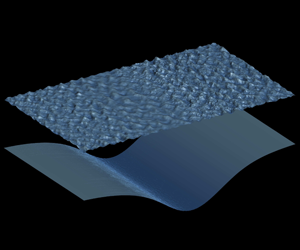
In this simulation-based study, we investigate the surface roughness signature induced by internal solitary waves in oceans. We present the first-ever effort to directly capture the surface roughness signature with a deterministic two-layer model to avoid the singularity encountered in the traditional wave–current interaction theory. By capturing over four million wave components, the simulation resolves the surface wave and internal wave dynamics simultaneously. The surface signature characterized by a rough region followed by a smooth region travelling with an internal wave is quantified by the local wave geometry variation and the wave energy change. The surface wave dynamics are analysed in the wavenumber–frequency slope spectrum calculated in the frame moving with the internal wave. The asymmetric behaviours of right-moving and left-moving surface waves are found to contribute to the surface signature formation. Our results show that the formation of the surface signature is essentially an energy-conservative process and justify the use of the wave-phase-resolved two-layer model.
- Type
- JFM Rapids
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 6
- Cited by


