Hostname: page-component-f554764f5-nt87m Total loading time: 0 Render date: 2025-04-19T01:59:38.171Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulations of turbulent viscoelastic jets

Published online by Cambridge University Press:  20 July 2020

Mateus C. Guimarães Open the ORCID record for Mateus C. Guimarães [Opens in a new window] ,
Nuno Pimentel ,
Fernando T. Pinho Open the ORCID record for Fernando T. Pinho [Opens in a new window]  and
Carlos B. da Silva Open the ORCID record for Carlos B. da Silva [Opens in a new window]
Mateus C. Guimarães
Affiliation:
IDMEC/LAETA, Instituto Superior Técnico, Universidade de Lisboa, 1049-001Lisboa, Portugal
Nuno Pimentel
Affiliation:
IDMEC/LAETA, Instituto Superior Técnico, Universidade de Lisboa, 1049-001Lisboa, Portugal
Fernando T. Pinho
Affiliation:
CEFT, Faculdade de Engenharia, Universidade do Porto, 4200-465Porto, Portugal
Carlos B. da Silva*
Affiliation:
IDMEC/LAETA, Instituto Superior Técnico, Universidade de Lisboa, 1049-001Lisboa, Portugal
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

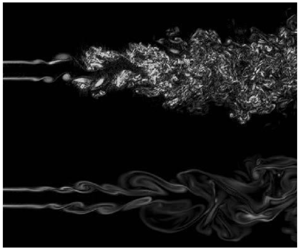
Direct numerical simulations (DNS) of spatially evolving turbulent planar jets of viscoelastic fluids described by the FENE-P model, such as those consisting of a Newtonian fluid solvent carrying long chain polymer molecules, are carried out in order to develop a theory for the far field of turbulent jets of viscoelastic fluids. New evolution relations for the jet shear-layer thickness $\unicode[STIX]{x1D6FF}(x)$, centreline velocity $U_{c}(x)$ and maximum polymer stresses $\unicode[STIX]{x1D70E}_{c}^{[p]}(x)$ are derived and validated by the new DNS data, yielding $\unicode[STIX]{x1D6FF}(x)\sim x$, $U_{c}(x)\sim x^{-1/2}$, and $\unicode[STIX]{x1D70E}_{c}^{[p]}(x)\sim x^{-5/2}$, respectively, where $x$ is the coordinate in the streamwise direction. It is shown that, compared with a classical (Newtonian) turbulent jet, the effect of the polymers is to reduce the spreading rate, centreline velocity decay, Reynolds stresses and viscous dissipation rate. The self-preserving character of the flow is analysed and it is shown that profiles of mean velocity, Reynolds stresses and polymer stresses are self-similar provided the proper scales are used in the normalisation of these quantities. A fundamental difference from the Newtonian jet in this regard is the necessity for two, instead of only one, different velocity and length scales to properly characterise the evolution of the turbulent flow. These extra velocity and length scales are directly related to a time scale associated with the characteristic fading memory property of viscoelastic fluids.

JFM classification

Wakes/Jets: Jets Non-Newtonian Flows: Viscoelasticity Turbulent Flows: Turbulence simulation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 899 , 25 September 2020 , A11
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Barker, S. J. 1973 Laser-doppler measurements on a round turbulent jet in dilute polymer solutions. J. Fluid Mech. 60 (4), 721–731.CrossRefGoogle Scholar
Berman, N. S. & Tan, H. 1985 Two-component laser doppler velocimeter studies of submerged jets of dilute polymer solutions. AIChE J. 31 (2), 208–215.CrossRefGoogle Scholar
Berman, N. S. 1986 Dispersion measurements in a polymer solution turbulent submerged jet. In AIP Conference Proceedings, vol. 137, pp. 129–133. AIP.CrossRefGoogle Scholar
Bird, R. B., Curtiss, C. F., Armstrong, R. C. & Hassager, O. 1987 Dynamics of Polymeric Liquids. Volume 2: Kinetic Theory. Wiley.Google Scholar
Bird, R. B., Dotson, P. J. & Johnson, N. L. 1980 Polymer solution rheology based on a finitely extensible bead-spring chain model. J. Non-Newtonian Fluid Mech. 7, 213–235.CrossRefGoogle Scholar
Browne, L. J. S., Antonia, R. A., Rajagopalan, S. & Chambers, A. J. 1982 Interaction region of a two-dimensional turbulent plane jet in still air. In Structure of Complex Turbulent Shear Flow, IUTAM Symp. Marseille (ed. Dumas, R. & Fulachier, L.). Springer.Google Scholar
Cafiero, G. & Vassilicos, J. C. 2019 Non-equilibrium turbulence scalings and self-similarity in turbulent planar jets. Phil. Trans. R. Soc. Lond. A 475, 0190038.Google ScholarPubMed
Cai, W., Li, D. & Zhang, H. 2010 DNS study of decaying homogeneous isotropic turbulence with polymer additives. J. Fluid. Mech. 665, 334–356.CrossRefGoogle Scholar
Canuto, C., Hussaini, M. Y., Quarteroni, A. & Zang, T. A. 1987 Spectral Methods in Fluid Dynamics. Springer.Google Scholar
Comte, P., Silvestrini, J. H. & Bégou, P. 1998 Streamwise vortices in large-eddy simulations of mixing layers. Eur. J. Mech. (B/Fluids) 17 (4), 615–639.CrossRefGoogle Scholar
Deo, R. C., Mi, J. & Nathan, G. J. 2008 The influence of Reynolds number on a plane jet. Phys. Fluids 20, 075108.CrossRefGoogle Scholar
Deo, R. C., Nathan, G. J. & Mi, J. 2013 Similarity analysis of the momentum field of a subsonic, plane air jet with varying jet-exit and local Reynolds numbers. Phys. Fluids 25, 015115.CrossRefGoogle Scholar
Ferreira, P. O., da Silva, C. B. & Pinho, F. T. 2016 Large-eddy simulations of forced isotropic turbulence with viscoelastic fluids described by the FENE-P model. Phys. Fluids 28 (12), 125104.CrossRefGoogle Scholar
Filipsson, L. G. R., Lagerstedt, J. H. T. & Bark, F. H. 1977 A note on the analogous behaviour of turbulent jets of dilute polymer solutions and fibre suspensions. J. Non-Newtonian Fluid Mech. 3 (1), 97–103.CrossRefGoogle Scholar
Gadd, G. E. 1965 Turbulence damping and drag reduction produced by certain additives in water. Nature 206 (4983), 463–467.CrossRefGoogle Scholar
Gadd, G. E. 1966 Reduction of turbulent friction in liquids by dissolved additives. Nature 212 (5065), 874–877.CrossRefGoogle Scholar
Goren, Y. & Norbury, J. F. 1967 Turbulent flow of dilute aqueous polymer solutions. J. Basic Engng 89 (4), 814–822.CrossRefGoogle Scholar
Graham, M. D. 2014 Drag reduction and the dynamics of turbulence in simple and complex fluids. Phys. Fluids 26 (10), 101301.CrossRefGoogle Scholar
Gutmark, E. & Wygnansky, I. 1976 The planar turbulent jet. J. Fluid Mech. 73, 465–495.CrossRefGoogle Scholar
Gyr, A. 1996 Effects of polymer additives on the large scale structure of a two-dimensional submerged jet. Appl. Sci. Res. 56 (1), 13–18.CrossRefGoogle Scholar
Horiuti, K., Matsumoto, K. & Fujiwara, K. 2013 Remarkable drag reduction in non-affine viscoelastic turbulent flows. Phys. Fluids 25, 015106.CrossRefGoogle Scholar
Hoyt, J. W., Taylor, J. J. & Runge, C. D. 1974 The structure of jets of water and polymer solution in air. J. Fluid Mech. 63 (4), 635–640.CrossRefGoogle Scholar
Jackley, D. N. 1967 Drag-reducing fluids in a free turbulent jet. Intl Shipbuild. Progr. 14 (152), 158–165.CrossRefGoogle Scholar
Kim, J. & Moin, P. 1985 Application of a fractional-step method to incompressible Navier–Stokes equations. J. Comput. Phys. 59, 308–323.CrossRefGoogle Scholar
Kim, K., Li, C., Sureshkumar, R., Balachandar, S. & Adrian, R. J. 2007 Effects of polymer stresses on eddy structures in drag-reduced turbulent channel flow. J. Fluid Mech. 584, 281–299.CrossRefGoogle Scholar
Koziol, K. & Glowacki, P. 1989 Turbulent jets of dilute polymer solutions. J. Non-Newtonian Fluid Mech. 32 (3), 311–328.CrossRefGoogle Scholar
Kurganov, A. & Tadmor, E. 2000 New high-resolution central schemes for nonlinear conservation laws and convection–diffusion equations. J. Comput. Phys. 160 (1), 241–282.CrossRefGoogle Scholar
Layek, G. C. & Sunita 2018 Non-Kolmogorov dissipation in a turbulent planar jet. Phys. Rev. Fluids 3, 124605.CrossRefGoogle Scholar
Lele, S. K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42.CrossRefGoogle Scholar
Lumley, J. L. 1973 Drag reduction in turbulent flow by polymer additives. J. Polymer Sci.: Macromol. Rev. 7 (1), 263–290.Google Scholar
Metzner, A. B. & Metzner, A. P. 1970 Stress levels in rapid extensional flows of polymeric fluids. Rheol. Acta 9 (2), 174–181.CrossRefGoogle Scholar
Orlanski, I. 1976 A simple boundary condition for unbounded hyperbolic flows. J. Comput. Phys. 21 (3), 251–269.CrossRefGoogle Scholar
Ouellette, N. T., Xu, H. & Bodenschatz, E. 2009 Bulk turbulence in dilute polymer solutions. J. Fluid Mech. 629, 375–385.CrossRefGoogle Scholar
Parvar, S., da Silva, C. B. & Pinho, F. T. 2020a Local similarity solution for steady laminar planar jet flow of viscoelastic FENE-P fluids. J. Non-Newtonian Fluid Mech. 279, 104–265.CrossRefGoogle Scholar
Parvar, S., da Silva, C. B. & Pinho, F. T. 2020b Corrigendum to ‘Local similarity solution for steady laminar planar jet flow of viscoelastic FENE-P fluids’. J. Non-Newtonian Fluid Mech. 281, 104–309.CrossRefGoogle Scholar
Perlekar, P., Mitra, D. & Pandit, R. 2006 Manifestations of drag reduction by polymer additives in decaying, homogeneous, isotropic turbulence. Phys. Rev. Lett. 97 (26), 264501.CrossRefGoogle ScholarPubMed
Perlekar, P., Mitra, D. & Pandit, R. 2010 Direct numerical simulations of statistically steady, homogeneous, isotropic fluid turbulence with polymer additives. Phys. Rev. E 82, 66313.Google ScholarPubMed
Pimentel, N.2019 Direct numerical simulation of turbulent planar jets with polymer additives. Master’s thesis, Instituto Superior Técnico, University of Lisbon.Google Scholar
Pinho, F. T., Li, C. F., Younis, B. A. & Sureshkumar, R. 2008 A low Reynolds number turbulence closure for viscoelastic fluids. J. Non-Newtonian Fluid Mech. 154 (2–3), 89–108.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Pownall, R. A. & Kiser, K. M. 1970 Rate of spread of jets of non-Newtonian fluids. Indust. Engng Chem. Fundam. 9 (2), 293–297.CrossRefGoogle Scholar
Ramaprian, R. & Chandrasekhara, M. S. 1985 LDA measurements in plane turbulent jets. Trans. ASME J. Fluids Engng 107 (2), 264–271.CrossRefGoogle Scholar
Sadeghi, H., Oberlack, M. & Gauding, M. 2018 On new scaling laws in a temporally evolving turbulent plane jet using Lie symmetry analysis and direct numerical simulation. J. Fluid Mech. 854, 233–260.CrossRefGoogle Scholar
Serth, R. W. & Kiser, K. M. 1970 The effect of turbulence on hot-film anemometer response in viscoelastic fluids. AIChE J. 16 (2), 163–168.CrossRefGoogle Scholar
Seyer, F. A. & Metzner, A. B. 1969 Turbulence phenomena in drag reducing systems. AIChE J. 15 (3), 426–434.CrossRefGoogle Scholar
Shul’Man, Z. P., Pokryvailo, N. A., Kovalevskaya, N. D. & Kulebyakin, V. V. 1973 Measurement of the turbulent flow structure of submerged jets of polymer solutions. J. Engng Phys. 25 (6), 1475–1482.CrossRefGoogle Scholar
da Silva, C. B.2001 The role of coherent structures in the control and interscale interactions of round, plane and coaxial jets. PhD thesis, INPG, Grenoble.Google Scholar
da Silva, C. B., Hunt, J. C. R., Eames, I. & Westerweel, J. 2014 Interfacial layers between regions of different turbulent intensity. Annu. Rev. Fluid Mech. 46, 567–590.CrossRefGoogle Scholar
da Silva, C. B., Lopes, D. C. & Raman, V. 2015 The effect of subgrid-scale models on the entrainment of a passive scalar in a turbulent planar jet. J. Turbul. 16 (4), 342–366.CrossRefGoogle Scholar
da Silva, C. B. & Métais, O. 2002 On the influence of coherent structures upon interscale interactions in turbulent plane jets. J. Fluid Mech. 473, 103–145.CrossRefGoogle Scholar
Stanley, S., Sarkar, S. & Mellado, J. P. 2002 A study of the flowfield evolution and mixing in a planar turbulent jet using direct numerical simulation. J. Fluid Mech. 450, 377–407.CrossRefGoogle Scholar
Sureshkumar, R., Beris, A. N. & Handler, R. A. 1997 Direct numerical simulation of the turbulent channel flow of a polymer solution. Phys. Fluids 9, 743–755.CrossRefGoogle Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.CrossRefGoogle Scholar
Thomas, F. O. & Chu, H. C. 1989 An experimental investigation of the transition of the planar jet: subharmonic suppression and upstream feedback. Phys. Fluids 1 (9), 1566–1587.CrossRefGoogle Scholar
Thomas, F. O. & Prakash, K. M. K. 1991 An investigation of the natural transition of an untuned planar jet. Phys. Fluids A 3 (1), 90–105.CrossRefGoogle Scholar
Tirtaatmadja, V. & Sridhar, T. 1993 A filament stretching device for measurement of extensional viscosity. J. Rheol. 37 (6), 1081–1102.CrossRefGoogle Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Usui, H., Sano, Y., Kawata, T. & Sakai, K. 1980 Turbulent round jet submerged in dilute drag reducing polymer solutions. Technol. Rep. Yamaguchi Univ. 2 (4), 399–408.Google Scholar
Vaithianathan, T., Robert, A., Brasseur, J. G. & Collins, L. R. 2006 An improved algorithm for simulating three-dimensional, viscoelastic turbulence. J. Non-Newtonian Fluid Mech. 140 (1), 3–22.CrossRefGoogle Scholar
Valente, P. C., da Silva, C. B. & Pinho, F. T. 2014 The effect of viscoelasticity on the turbulent kinetic cascade. J. Fluid. Mech. 760, 39–62.CrossRefGoogle Scholar
Valente, P. C., da Silva, C. B. & Pinho, F. T. 2016 Energy spectra in elasto-inertial turbulence. Phys. Fluids 28, 075108.CrossRefGoogle Scholar
Vincenzi, D., Perlekar, P., Biferale, L. & Toschi, F. 2015 Impact of the Peterlin approximation on polymer dynamics in turbulent flows. Phys. Rev. E 92 (5), 053004.Google ScholarPubMed
Vlasov, S. A., Isaeva, O. V. & Kalashnikov, V. N. 1973 Average and pulsation components of velocity in submerged jets of polymer solutions. J. Engng Phys. Thermophys. 25 (6), 1483–1487.CrossRefGoogle Scholar
Watanabe, T. & Gotoh, T. 2010 Coil-stretch transition in an ensemble of polymers in isotropic turbulence. Phys. Rev. E 81 (6), 066301.Google Scholar
White, A. 1969 Some observations on the flow characteristics of certain dilute macromolecular solutions. In Viscous Drag Reduction (ed. Wells, C. S.), pp. 297–311. Springer.CrossRefGoogle Scholar
White, A.1972 Turbulence and drag reduction with polymer additives. PhD thesis, Middlesex Polytechnic.Google Scholar
White, D. A. 1967 Velocity measurements in axisymmetric jets of dilute polymer solutions. J. Fluid Mech. 28 (1), 195–204.CrossRefGoogle Scholar
Williamson, J. H. 1980 Low-storage Runge–Kutta schemes. J. Comput. Phys. 35, 48–56.CrossRefGoogle Scholar
Wu, J. 1969 Drag reduction in external flows of additive solutions. In Viscous Drag Reduction (ed. Wells, C. S.), pp. 331–350. Springer.CrossRefGoogle Scholar
Xi, H.-D., Bodenschatz, E. & Xu, H. 2013 Elastic energy flux by flexible polymers in fluid turbulence. Phys. Rev. Lett. 111, 24501.CrossRefGoogle ScholarPubMed
Youssef, J.2012 Étude expérimentale d’un jet plan turbulent se développant dans un flux uniforme en co-courant. PhD thesis, ISAE-ENSMA Ecole Nationale Supérieure de Mécanique et d’Aérotechique-Poitiers.Google Scholar