Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-24T20:49:23.610Z Has data issue: false hasContentIssue false
  • English
  • Français

Dense-gas effects on compressible boundary-layer stability

Published online by Cambridge University Press:  22 April 2020

X. Gloerfelt Open the ORCID record for X. Gloerfelt [Opens in a new window] ,
J.-C. Robinet Open the ORCID record for J.-C. Robinet [Opens in a new window] ,
L. Sciacovelli Open the ORCID record for L. Sciacovelli [Opens in a new window] ,
P. Cinnella Open the ORCID record for P. Cinnella [Opens in a new window]  and
F. Grasso Open the ORCID record for F. Grasso [Opens in a new window]
X. Gloerfelt*
Affiliation:
DynFluid Laboratory, Arts et Métiers ParisTech, 75013Paris, France
J.-C. Robinet
Affiliation:
DynFluid Laboratory, Arts et Métiers ParisTech, 75013Paris, France
L. Sciacovelli
Affiliation:
DynFluid Laboratory, Arts et Métiers ParisTech, 75013Paris, France
P. Cinnella
Affiliation:
DynFluid Laboratory, Arts et Métiers ParisTech, 75013Paris, France
F. Grasso
Affiliation:
DynFluid Laboratory, Conservatoire National des Arts et Métiers, 75003Paris, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

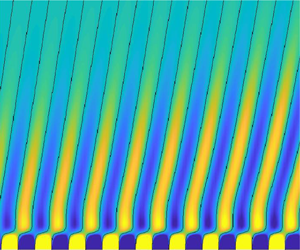
A study of dense-gas effects on the stability of compressible boundary-layer flows is conducted. From the laminar similarity solution, the temperature variations are small due to the high specific heat of dense gases, leading to velocity profiles close to the incompressible ones. Concurrently, the complex thermodynamic properties of dense gases can lead to unconventional compressibility effects. In the subsonic regime, the Tollmien–Schlichting viscous mode is attenuated by compressibility effects and becomes preferentially skewed in line with the results based on the ideal-gas assumption. However, the absence of a generalized inflection point precludes the sustainability of the first mode by inviscid mechanisms. On the contrary, the viscous mode can be completely stable at supersonic speeds. At very high speeds, we have found instances of radiating supersonic instabilities with substantial amplification rates, i.e. waves that travel supersonically relative to the free-stream velocity. This acoustic mode has qualitatively similar features for various thermodynamic conditions and for different working fluids. This shows that the leading parameters governing the boundary-layer behaviour for the dense gas are the constant-pressure specific heat and, to a minor extent, the density-dependent viscosity. A satisfactory scaling of the mode characteristics is found to be proportional to the height of the layer near the wall that acts as a waveguide where acoustic waves may become trapped. This means that the supersonic mode has the same nature as Mack’s modes, even if its frequency for maximal amplification is greater. Direct numerical simulation accurately reproduces the development of the supersonic mode and emphasizes the radiation of the instability waves.

JFM classification

Aerodynamics: High-speed flow Boundary Layers: Boundary layer stability Compressible Flows: Compressible boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 893 , 25 June 2020 , A19
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akasaka, R., Zhou, Y. & Lemmon, E. W. 2015 A fundamental equation of state for 1, 1, 1, 3, 3-pentafluoropropane (R-245fa). J. Phys. Chem. Ref. Data 44 (1), 1–11.Google Scholar
Anderson, J. D. 2002 Modern Compressible Flow: With Historical Perspective, 3rd edn. McGraw-Hill Higher Education.Google Scholar
Balakumar, P. & Malik, M. R. 1992 Discrete modes and continuous spectra in supersonic boundary layers. J. Fluid Mech. 239, 631–656.CrossRefGoogle Scholar
Bitter, N.2015 Stability of hypervelocity boundary layers. PhD thesis, California Institute of Technology, Pasadena, CA.CrossRefGoogle Scholar
Bitter, N. & Shepherd, J. 2015 Stability of highly cooled hypervelocity boundary layers. J. Fluid Mech. 778, 586–620.CrossRefGoogle Scholar
Brès, G., Inkman, M., Colonius, T. & Fedorov, A. V. 2013 Second-mode attenuation and cancellation by porous coatings in a high-speed boundary layer. J. Fluid Mech. 726, 312–337.CrossRefGoogle Scholar
Broyden, C. G. 1965 A class of methods for solving nonlinear simultaneous equations. Maths Comput. 19 (92), 577–593.CrossRefGoogle Scholar
Chen, X., Boldini, P. C. & Song, F. 2019 Research on hypersonic boundary-layer stability with high-temperature effects. In Proceedings of the 2018 Asia-Pacific International Symposium on Aerospace Technology (APISAT 2018), LNEE, pp. 499–512. Springer Nature.CrossRefGoogle Scholar
Chokani, N. 1999 Nonlinear spectral dynamics of hypersonic laminar boundary layer flow. Phys. Fluids 11 (12), 3846–3851.CrossRefGoogle Scholar
Chung, T. H., Ajlan, M., Lee, L. L. & Starling, K. E. 1988 Generalized multiparameter correlation for nonpolar and polar fluid transport properties. Ind. Engng Chem. Res. 27 (4), 671–679.CrossRefGoogle Scholar
Chuvakhov, P. V. & Fedorov, A. V. 2016 Spontaneous radiation of sound by instability of a highly cooled hypersonic boundary layer. J. Fluid Mech. 805, 188–206.CrossRefGoogle Scholar
Cinnella, P. & Congedo, P. M. 2007 Inviscid and viscous aerodynamics of dense gases. J. Fluid Mech. 580, 179–217.CrossRefGoogle Scholar
Colonna, P., Casati, E., Trapp, C., Mathijssen, T., Larjola, J., Turunen-Saaresti, T. & Uusitalo, A. 2015 Organic Rankine cycle power systems: from the concept to current technology, applications, and an outlook to the future. Trans. ASME J. Engng Gas Turbines Power 137 (10), 100801.CrossRefGoogle Scholar
Colonna, P. & Guardone, A. 2006 Molecular interpretation of nonclassical gas dynamics of dense vapors under the van der Waals model. Phys. Fluids 18, 056101.CrossRefGoogle Scholar
Colonna, P., Nannan, N. R. & Guardone, A. 2008 Multiparameter equations of state for siloxanes: [(CH3)3-Si-O1/2]2-[O-Si-(CH3)2]i = 1, …, 3, and [O-Si-(CH3)2]6. Fluid Phase Equilib. 263, 115–130.CrossRefGoogle Scholar
Craig, S. A., Humble, R. A., Hofferth, J. W. & Saric, W. S. 2019 Nonlinear behaviour of the Mack mode in a hypersonic boundary layer. J. Fluid Mech. 872, 74–99.CrossRefGoogle Scholar
Cramer, M. S. 1989 Negative nonlinearity in selected fluorocarbons. Phys. Fluids A 1 (11), 1894–1897.CrossRefGoogle Scholar
Cramer, M. S. 1991 Nonclassical dynamics of classical gases. In Nonlinear Waves in Real Fluids. International Centre for Mechanical Sciences (Courses and Lectures) (ed. Kluwick, A.), vol. 315, pp. 91–145. Springer.CrossRefGoogle Scholar
Cramer, M. S. & Kluwick, A. 1984 On the propagation of waves exhibiting both positive and negative nonlinearity. J. Fluid Mech. 142, 9–37.CrossRefGoogle Scholar
Cramer, M. S., Whitlock, S. T. & Tarkenton, G. M. 1996 Transonic and boundary layer similarity laws in dense gases. Trans. ASME J. Fluids Engng 118 (3), 481–485.CrossRefGoogle Scholar
Dunn, D. W. & Lin, C. C. 1955 On the stability of the laminar boundary layer in a compressible fluid. J. Aero. Sci. 22 (4), 455–477.CrossRefGoogle Scholar
Egorov, I. V., Fedorov, A. V. & Soudakov, V. G. 2006 Direct numerical simulation of disturbances generated by periodic suction-blowing in a hypersonic boundary layer. Theor. Comput. Fluid Dyn. 20 (1), 41–54.CrossRefGoogle Scholar
Fedorov, A. 2011 Transition and stability for high-speed boundary layers. Annu. Rev. Fluid Mech. 43, 79–95.CrossRefGoogle Scholar
Fedorov, A. & Tumin, A. 2011 High-speed boundary-layer instability: old terminilogy and a new framework. AIAA J. 49 (8), 1647–1657.CrossRefGoogle Scholar
Fedorov, A. V. 2003 Receptivity of a high-speed boundary layer to acoustic disturbances. J. Fluid Mech. 491, 101–129.CrossRefGoogle Scholar
Fedorov, A. V. & Khokhlov, A. P. 2001 Prehistory on instability in a hypersonic boundary layer. Theor. Comput. Fluid Dyn. 14, 339–375.Google Scholar
Franko, K. J. & Lele, S. K. 2013 Breakdown mechanisms and heat transfer overshoot in hypersonic zero pressure gradient boundary layers. J. Fluid Mech. 730, 491–532.CrossRefGoogle Scholar
Gloerfelt, X. & Robinet, J.-C. 2017 Silent inflow condition for turbulent boundary layers. Phys. Rev. F 2, 124603.Google Scholar
Govindarajan, R. & Sahu, K. C. 2014 Instabilities in viscosity-stratified flow. Annu. Rev. Fluid Mech. 46, 331–353.CrossRefGoogle Scholar
Guardone, A., Vigevano, L. & Argrow, B. M. 2004 Assessment of thermodynamic models for dense gas dynamics. Phys. Fluids 16 (11), 3878–3887.CrossRefGoogle Scholar
Gushchin, V. R. & Fedorov, A. V. 1989 Short-wave instability in a perfect-gas shock layer. Fluid Dyn. 24 (1), 7–10.Google Scholar
Gushchin, V. R. & Fedorov, A. V. 1990 Excitation and development of unstable disturbances in a supersonic boundary layer. Fluid Dyn. 25 (3), 344–352.Google Scholar
Han, Y., Liu, J. & Luo, J. 2016 Non-parallel effects on mode characteristics in hypersonic boundary layers. In 24th International Congress of Theoretical and Applied Mechanics (XXIV ICTAM, 21–26 August, Montreal, Canada) (ed. Floryan, J. M.), Contributions to the Foundations of Multidisciplinary Research in Mechanics, vol. 2, pp. 568–569. IUTAM.Google Scholar
Harinck, J., Guardone, A. & Colonna, P. 2009 The influence of molecular complexity on expanding flows of ideal and dense gases. Phys. Fluids 21, 086101.CrossRefGoogle Scholar
Huber, M. L., Laesecke, A. & Perkins, R. A. 2003 Model for the viscosity and thermal conductivity of refrigerants, including a new correlation for the viscosity of R134a. Ind. Engng Chem. Res. 42 (13), 3163–3178.CrossRefGoogle Scholar
Hudson, M. L., Chokani, N. & Candler, G. V. 1997 Linear stability of hypersonic flow in thermochemical nonequilibrium. AIAA J. 35 (6), 958–964.CrossRefGoogle Scholar
Kluwick, A. 1994 Interacting laminar boundary layers of dense gases. Acta Mechanica [Suppl] 4, 335–349.Google Scholar
Kluwick, A. 2004 Internal flows of dense gases. Acta Mechanica 169, 123–143.CrossRefGoogle Scholar
Kluwick, A. 2017 Non-ideal compressible fluid dynamics: a challenge for theory. J. Phys.: Conf. Ser. 821 (1), 012001.Google Scholar
Knisely, C. P. & Zhong, X. 2019a Significant supersonic modes and the wall temperature effect in hypersonic boundary layers. AIAA J. 57 (4), 1552–1566.CrossRefGoogle Scholar
Knisely, C. P. & Zhong, X. 2019b Sound radiation by supersonic unstable modes in hypersonic blunt cone boundary layers. I. Linear stability theory. Phys. Fluids 31, 024103.Google Scholar
Knisely, C. P. & Zhong, X. 2019c Sound radiation by supersonic unstable modes in hypersonic blunt cone boundary layers. II. Direct numerical simulation. Phys. Fluids 31, 024104.Google Scholar
Lees, L.1947 The stability of the laminar boundary layer in a compressible fluid. NACA Tech. Note 1360.Google Scholar
Lees, L. & Lin, C.C.1946 Investigation of the stability of the laminar boundary layer in a compressible fluid. NACA Tech. Note 1115.Google Scholar
Lemmon, E. W., Huber, M. L. & McLinden, M. O.2013 NIST standard reference database 23: Reference fluid thermodynamic and transport properties – REFPROP, Version 9.1. National Institute of Standards and Technology. Available at: http://www.nist.gov/srd/nist23.cfm.Google Scholar
Ma, Y. & Zhong, X. 2003 Receptivity of a supersonic boundary layer over a flat plate. Part 1. Wave structures and interactions. J. Fluid Mech. 488, 31–78.CrossRefGoogle Scholar
Mack, L. M. 1975 Linear stability theory and the problem of supersonic boundary-layer transition. AIAA J. 13 (3), 278–289.CrossRefGoogle Scholar
Mack, L. M.1984a Boundary layer linear stability theory. Tech. rep. 709, AGARD.Google Scholar
Mack, L. M. 1984b Remarks on disputed numerical results in compressible boundary-layer stability theory. Phys. Fluids 27 (2), 342–347.CrossRefGoogle Scholar
Mack, L. M. 1987 Review of linear compressible stability theory. In Stability of Time Dependent and Spatially Varying Flows (ed. Dwoyer, D. L. et al. ), pp. 167–187. Springer.Google Scholar
Mack, L. M. 1990 On the inviscid acoustic-mode instability of supersonic shear flows. Part 1. Two-dimensional waves. Theor. Comput. Fluid Dyn. 2, 97–123.Google Scholar
Malik, M. R. 1991 Prediction and control of transition in supersonic and hypersonic boundary layers. AIAA J. 27 (11), 1487–1493.CrossRefGoogle Scholar
Malik, M. R. & Anderson, E. C. 1991 Real gas effects on hypersonic boundary-layer stability. Phys. Fluids A 3 (5), 803–821.CrossRefGoogle Scholar
Malik, M. R. & Spall, R. E. 1991 On the stability of compressible flow past axisymmetric bodies. J. Fluid Mech. 228, 443–463.Google Scholar
Martin, J. J. & Hou, Y. C. 1955 Development of an equation of state for gases. AIChE J. 1 (2), 142–151.CrossRefGoogle Scholar
Marxen, O., Magin, T., Iaccarino, G. & Shaqfeh, E. S. G. 2011 A high-order numerical method to study hypersonic boundary-layer instability including high-temperature gas effects. Phys. Fluids 23, 084108.CrossRefGoogle Scholar
Mathijssen, T., Gallo, M., Casati, E., Nannan, N. R., Zamfirescu, C., Guardone, A. & Colonna, P. 2015 The flexible asymmetric shock tube (FAST): a Ludwieg tube facility for wave propagation measurements in high-temperature vapours of organic fluids. Exp. Fluids 56, 195-1–195-12.Google Scholar
Mayer, C. J. S., Von Terzi, D. A. & Fasel, H. F. 2011 Direct numerical simulation of complete transition to turbulence via oblique breakdown at Mach 3. J. Fluid Mech. 674, 5–42.CrossRefGoogle Scholar
Morkovin, M. V.1987 Transition at hypersonic speeds. NASA Contractor Rep., ICASE 178315. NASA Langley Research Center.Google Scholar
Mortensen, C. H. 2018 Toward and understanding of supersonic modes in boundary-layer transition for hypersonic flow over blunt cones. J. Fluid Mech. 846, 789–814.CrossRefGoogle Scholar
Mortensen, C. H. & Zhong, X. 2018 Real-gas and surface-ablation effects on hypersonic boundary–layer instability over a blunt cone. AIAA J. 54 (3), 980–998.CrossRefGoogle Scholar
Perkins, R. A., Huber, M. L. & Assael, M. J. 2016 Measurements of the thermal conductivity of 1, 1, 1, 3-3-pentafluoropropane (R-245fa) and correlations for the viscosity and thermal conductivity surfaces. J. Chem. Engng Data 61, 3286–3294.CrossRefGoogle Scholar
Reed, H. L. & Balakumar, P. 1990 Compressible boundary-layer stability theory. Phys. Fluids A 2 (8), 1341–1349.CrossRefGoogle Scholar
Ren, J., Fu, S. & Pecnik, R. 2019a Linear instability of Poiseuille flows with highly non-ideal fluids. J. Fluid Mech. 859, 89–125.CrossRefGoogle Scholar
Ren, J., Marxen, O. & Pecnik, R. 2019b Boundary-layer stability of supercritical fluids in the vicinity of the Widom line. J. Fluid Mech. 871, 831–864.CrossRefGoogle Scholar
Reshotko, E. 1976 Boundary-layer stability and transition. Annu. Rev. Fluid Mech. 8, 311–349.CrossRefGoogle Scholar
Robinet, J.-C. & Gloerfelt, X. 2019 Instabilities in non-ideal fluids. J. Fluid Mech., Focus on Fluids 880, 1–4.CrossRefGoogle Scholar
Romei, A., Vimercati, D., Persico, G. & Guardone, A. 2020 Non-ideal compressible flows in supersonic turbine cascades. J. Fluid Mech. 882, A12–1–A12–26.CrossRefGoogle Scholar
Saika, B., Ramachandran, A., Sinha, K. & Govindarajan, R. 2017 Effects of viscosity and conductivity stratification on the linear stability and transient growth within compressible Couette flow. Phys. Fluids 29, 024105.Google Scholar
Salemi, L. C. & Fasel, H. F. 2018 Synchronization of second-mode instability waves for high-enthalpy hypersonic boundary layers. J. Fluid Mech. 838, R2–1–R2–14.CrossRefGoogle Scholar
Schlichting, H. & Gersten, K. 2003 Boundary Layer Theory, 8th edn. Springer.Google Scholar
Schmid, P. J. & Henningson, D. S. 2001 Stability and Transition in Shear Flows, (Applied Mathematical Sciences), vol. 142. Springer.CrossRefGoogle Scholar
Sciacovelli, L., Cinnella, P., Content, C. & Grasso, F. 2016 Dense gas effects in inviscid homogeneous isotropic turbulence. J. Fluid Mech. 800, 140–179.CrossRefGoogle Scholar
Sciacovelli, L., Cinnella, P. & Gloerfelt, X. 2017a Direct numerical simulations of supersonic turbulent channel flows of dense gases. J. Fluid Mech. 821, 153–199.CrossRefGoogle Scholar
Sciacovelli, L., Cinnella, P. & Grasso, F. 2017b Small-scale dynamics of dense gas compressible homogeneous isotropic turbulence. J. Fluid Mech. 825, 515–549.CrossRefGoogle Scholar
Sivasubramanian, H. & Fasel, H. F. 2015 Direct numerical simulation of transition in a sharp cone boundary layer at Mach 6: fundamental breakdown. J. Fluid Mech. 768, 175–218.CrossRefGoogle Scholar
Spinelli, A., Cammi, G., Conti, C. C., Gallarini, S., Zocca, M., Cozzi, F., Gaetani, P., Dossena, V. & Guardone, A. 2019 Experimental observation and thermodynamic modeling of non-ideal expanding flows of siloxane MDM vapor for ORC applications. Energy 168, 285–294.CrossRefGoogle Scholar
Stetson, K. F. & Kimmel, R. L.1992 On hypersonic boundary-layer stability. AIAA Paper (92-0737).CrossRefGoogle Scholar
Stuckert, G. & Reed, H. L. 1994 Linear disturbances in hypersonic, chemically reacting shock layers. AIAA J. 32 (7), 1384–1393.CrossRefGoogle Scholar
Tam, C. K. W. & Hu, F. Q. 1989 On the three families of instability waves of high-speed jets. J. Fluid Mech. 201, 447–483.CrossRefGoogle Scholar
Thol, M., Dubberke, F. H., Baumhögger, E., Vrabec, J. & Span, R. 2017 Speed of sound measurements and fundamental equations of state for octamethyltrisiloxane and decamethyltetrasiloxane. J. Chem. Engng Data 62, 2633–2648.CrossRefGoogle Scholar
Thompson, P. A. & Lambrakis, K. C. 1973 Negative shock waves. J. Fluid Mech. 60 (1), 187–208.CrossRefGoogle Scholar
Tillner-Roth, R. & Baehr, H. D. 1994 An international standard formulation for the thermodynamic properties of 1, 1, 1, 2-tetrafluoroethane (HFC-134a) for temperatures from 170 K to 455 K and pressures up to 70 MPa. J. Phys. Chem. Ref. Data 23, 657–720.CrossRefGoogle Scholar
Wazzan, A. R., Taghavi, H. & Keltner, G. 1984 The effect of Mach number on the spatial stability of adiabatic flat plate flow to oblique disturbances. Phys. Fluids 27 (2), 331–341.CrossRefGoogle Scholar
Zamfirescu, C. & Dincer, I. 2009 Performance investigation of high-temperature heat pumps with various BZT working fluids. Thermochim. Acta 488 (1–2), 66–77.CrossRefGoogle Scholar
Zhong, X. & Wang, X. 2012 Direct numerical simulation on the receptivity, instability, and transition of hypersonic boundary layers. Annu. Rev. Fluid Mech. 44, 527–561.CrossRefGoogle Scholar