Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-27T23:37:50.927Z Has data issue: false hasContentIssue false
  • English
  • Français

Counter-flow orbiting of the vortex centre in turbulent thermal convection

Published online by Cambridge University Press:  26 January 2022

Yi-Zhen Li ,
Xin Chen Open the ORCID record for Xin Chen [Opens in a new window] ,
Ao Xu Open the ORCID record for Ao Xu [Opens in a new window]  and
Heng-Dong Xi Open the ORCID record for Heng-Dong Xi [Opens in a new window]
Yi-Zhen Li
Affiliation:
School of Aeronautics and Institute of Extreme Mechanics, Northwestern Polytechnical University, Xi'an, 710072, PR China
Xin Chen
Affiliation:
School of Aeronautics and Institute of Extreme Mechanics, Northwestern Polytechnical University, Xi'an, 710072, PR China
Ao Xu
Affiliation:
School of Aeronautics and Institute of Extreme Mechanics, Northwestern Polytechnical University, Xi'an, 710072, PR China
Heng-Dong Xi*
Affiliation:
School of Aeronautics and Institute of Extreme Mechanics, Northwestern Polytechnical University, Xi'an, 710072, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

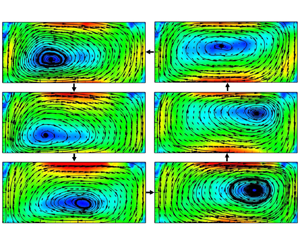
We present an experimental study of the large-scale vortex (or large-scale circulation, LSC) in turbulent Rayleigh–Bénard convection in a $\varGamma =\text {diameter}/\text {height}=2$ cylindrical cell. The working fluid is deionized water with Prandtl number ($Pr$) around 5.7, and the Rayleigh number ($Ra$) ranges from $7.64\times 10^7$ to $6.06\times 10^8$. We measured the velocity field in various vertical cross-sectional planes by using the planar particle image velocimetry technique. The velocity measurement in the LSC central plane shows that the flow is in the single-roll form, and the centre of the single-roll (vortex) does not always stay at the centre of the cell; instead, it orbits periodically in the direction opposite to the flow direction of the LSC, with its trajectory in the shape of an ellipse. The velocity measurements in the three vertical planes in parallel to the LSC central plane indicate that the flow is in the vortex tube form horizontally filling almost the whole cell, and the centre line of the vortex tube is consistent with the so-called ‘jump rope’ form proposed by a previous study that combined numerical simulation and local velocity measurements in the low $Pr$ case (Vogt et al., Proc. Natl Acad. Sci. USA, vol. 115, 2018, pp. 12674–12679). In addition, we found that the oscillation of the local velocity in $\varGamma =2$ cells originates from the periodical orbiting of the vortex centre. Our velocity measurements further indicate that the vortex centre orbiting is absent in $\varGamma =1$ cells, at least in the $Ra$ range of our experiments.

JFM classification

Convection: Plumes/thermals Turbulent Flows: Turbulent convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 935 , 25 March 2022 , A19
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.CrossRefGoogle Scholar
Bailon-Cuba, J., Emran, M.S. & Schumacher, J. 2010 Aspect ratio dependence of heat transfer and large-scale flow in turbulent convection. J. Fluid Mech. 655, 152–173.CrossRefGoogle Scholar
Brown, E. & Ahlers, G. 2009 The origin of oscillations of the large-scale circulation of turbulent Rayleigh–Bénard convection. J. Fluid Mech. 638, 383–400.CrossRefGoogle Scholar
Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Reorientation of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 95, 084503.CrossRefGoogle ScholarPubMed
Castaing, B., Gnuaratne, G., Heslot, F., Kadanoff, L., Libchaber, A., Thomae, S., Wu, X.-Z., Zaleski, S. & Zanetti, G. 1989 Scaling of hard thermal turbulence in Rayleigh–Bénard convection. J. Fluid Mech. 204, 1–30.CrossRefGoogle Scholar
Castillo-Castellanos, A., Podvin, A., Sergentand, B. & Rossi, M. 2019 Cessation and reversals of large-scale structures in square Rayleigh–Bénard cells. J. Fluid Mech. 877, 922–954.CrossRefGoogle Scholar
Chen, X., Huang, S.D., Xia, K.Q. & Xi, H.D. 2019 Emergence of substructures inside the large-scale circulation induces transition in flow reversals in turbulent thermal convection. J. Fluid Mech. 877, R1.CrossRefGoogle Scholar
Chen, X., Wang, D.P. & Xi, H.D. 2020 Reduced flow reversals in turbulent convection in the absence of corner vortices. J. Fluid Mech. 891, R5.CrossRefGoogle Scholar
Chilla, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.CrossRefGoogle ScholarPubMed
Funfschilling, D. & Ahlers, G. 2004 Plume motion and large-scale circulation in a cylindrical Rayleigh–Bénard cell. Phys. Rev. Lett. 92, 194502.CrossRefGoogle Scholar
Funfschilling, D., Brown, E. & Guenter, A. 2008 Torsional oscillations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 607, 119–139.CrossRefGoogle Scholar
Funfschilling, D., Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical samples with aspect ratio one and larger. J. Fluid Mech. 536, 145–154.CrossRefGoogle Scholar
Krishnamurti, R. & Howard, L.N. 1981 Large-scale flow generation in turbulent convection. Proc. Natl Acad. Sci. USA 78, 1981–1985.CrossRefGoogle ScholarPubMed
Lohse, D. & Xia, K.Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Lui, S.L. & Xia, K.Q. 1998 Spatial structure of the thermal boundary layer in turbulent convection. Phys. Rev. E 57, 5494–5503.CrossRefGoogle Scholar
Naert, A., Segawa, T. & Sano, M. 1997 High-Reynolds-number thermal turbulence in mercury. Phys. Rev. E 56, R1302–R1305.CrossRefGoogle Scholar
Ni, R., Huang, S.D. & Xia, K.Q. 2015 Reversals of the large-scale circulation in quasi-2D Rayleigh–Bénard convection. J. Fluid Mech. 778, R5.CrossRefGoogle Scholar
Niemela, J.J., Skrbek, L., Sreenivasan, K.R. & Donnelly, R.J. 2001 The wind in confined thermal convection. J. Fluid Mech. 449, 169–178.CrossRefGoogle Scholar
du Puits, R., Resagk, C. & Thess, A. 2007 Breakdown of wind in turbulent thermal convection. Phys. Rev. E 75, 016302.CrossRefGoogle ScholarPubMed
Qiu, X.L., Shang, X.D., Tong, P. & Xia, K.Q. 2004 Velocity oscillations in turbulent Rayleigh–Bénard convection. Phys. Fluids 16 (2), 412–423.CrossRefGoogle Scholar
Qiu, X.L. & Tong, P. 2001 a Large-scale velocity structures in turbulent thermal convection. Phys. Rev. E 64, 036304.CrossRefGoogle ScholarPubMed
Qiu, X.L. & Tong, P. 2001 b Onset of coherent oscillations in turbulent Rayleigh–Benard convection. Phys. Rev. Lett. 87 (9), 094501.CrossRefGoogle ScholarPubMed
Siggia, E.D. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26, 137–168.CrossRefGoogle Scholar
Sugiyama, K., Ni, R., Stevens, R.J., Chan, T.S., Zhou, S.Q., Xi, H.D., Sun, C., Grossmann, S., Xia, K.Q. & Lohse, D. 2010 Flow reversals in thermally driven turbulence. Phys. Rev. Lett. 105, 034503.CrossRefGoogle ScholarPubMed
Sun, C., Ren, L.Y., Song, H. & Xia, K.Q. 2005 a Heat transport by turbulent Rayleigh–Bénard convection in 1 m diameter cylindrical cells of widely varying aspect ratio. J. Fluid Mech. 542, 165–174.CrossRefGoogle Scholar
Sun, C. & Xia, K.Q. 2005 Scaling of the Reynolds number in turbulent thermal convection. Phys. Rev. E 72 (6 Pt 2), 067302.CrossRefGoogle ScholarPubMed
Sun, C., Xia, K.Q. & Tong, P. 2005 b Three-dimensional flow structures and dynamics of turbulent thermal convection in a cylindrical cell. Phys. Rev. E 72, 026302.CrossRefGoogle Scholar
Tsuji, Y., Mizuno, T., Mashiko, T. & Sano, M. 2005 Mean wind in convective turbulence of mercury. Phys. Rev. Lett. 94, 034501.CrossRefGoogle ScholarPubMed
Verma, M.C. & Mahendra, K. 2013 Flow reversals in turbulent convection via vortex reconnections. Phys. Rev. Lett. 110, 114503.Google Scholar
Vogt, T., Horn, S., Grannan, A.M. & Aurnou, J.M. 2018 Jump rope vortex in liquid metal convection. Proc. Natl Acad. Sci. USA 115, 12674–12679.CrossRefGoogle ScholarPubMed
Wang, Y., Lai, P.Y., Song, H. & Tong, P. 2018 Mechanism of large-scale flow reversals in turbulent thermal convection. Sci. Adv. 4 (11), eaat7480.CrossRefGoogle ScholarPubMed
Xi, H.D., Lam, S. & Xia, K.Q. 2004 From laminar plumes to organized flows: the onset of large-scale circulation in turbulent thermal convection. J. Fluid Mech. 503, 47–56.CrossRefGoogle Scholar
Xi, H.D. & Xia, K.Q. 2007 Cessations and reversals of the large-scale circulation in turbulent thermal convection. Phys. Rev. E 75, 066307.CrossRefGoogle ScholarPubMed
Xi, H.D. & Xia, K.Q. 2008 Azimuthal motion, reorientation, cessation, and reversal of the large-scale circulation in turbulent thermal convection: a comparative study in aspect ratio one and one-half geometries. Phys. Rev. E 78, 036326.CrossRefGoogle ScholarPubMed
Xi, H.D., Zhang, Y.B., Hao, J.T. & Xia, K.Q. 2016 Higher-order flow modes in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 805, 31–51.CrossRefGoogle Scholar
Xi, H.D., Zhou, Q. & Xia, K. -Q. 2006 Azimuthal motion of the mean wind in turbulent thermal convection. Phys. Rev. E 73, 056312.CrossRefGoogle ScholarPubMed
Xi, H.D., Zhou, S.Q., Zhou, Q., Chan, T.S. & Xia, K.Q. 2009 Origin of the temperature oscillation in turbulent thermal convection. Phys. Rev. Lett. 102, 044503.CrossRefGoogle ScholarPubMed
Xia, K.Q. 2013 Current trends and future directions in turbulent thermal convection. Theor. Appl. Mech. Lett. 3, 052001.CrossRefGoogle Scholar
Xia, K.Q., Sun, C. & Cheung, Y.H. 2008 Large scale velocity structures in turbulent thermal convection with widely varying aspect ratio. In Proceedings of the 14th International Symposium on Applications of Laser Techniques to Fluid Mechanics.Google Scholar
Xu, A., Chen, X. & Xi, H.D. 2021 Tristable flow states and reversal of the large-scale circulation in two-dimensional circular convection cells. J. Fluid Mech. 910, A33.CrossRefGoogle Scholar
Zhou, Q., Xi, H.D., Zhou, S.Q., Sun, C. & Xia, K.Q. 2009 Oscillations of the large-scale circulation in turbulent Rayleigh–Bénard convection: the sloshing mode and its relationship with the torsional mode. J. Fluid Mech. 630, 367–390.CrossRefGoogle Scholar
Zhu, X., Jiang, L.-F., Zhou, Q. & Sun, C. 2019 Turbulent Rayleigh–Bénard convection in an annular cell. J. Fluid Mech. 869, R5.CrossRefGoogle Scholar
Zürner, T., Schindler, F., Vogt, T., Eckert, S. & Schumacher, J. 2019 Combined measurement of velocity and temperature in liquid metal convection. J. Fluid Mech. 876, 1108–1128.CrossRefGoogle Scholar
Zwirner, L., Khalilov, R., Kolesnichenko, I., Mamykin, A., Mandrykin, S., Pavlinov, A., Shestakov, A., Teimurazov, A., Frick, P. & Shishkina, O. 2019 The influence of the cell inclination on the heat transport and large-scale circulation in liquid metal convection. J. Fluid Mech. 884, A18.CrossRefGoogle Scholar

Li et al. supplementary movie 1

See pdf file for movie caption

Download Li et al. supplementary movie 1(Video)
Video 3.7 MB

Li et al. supplementary movie 2

See pdf file for movie caption

Download Li et al. supplementary movie 2(Video)
Video 3.3 MB

Li et al. supplementary movie 3

See pdf file for movie caption

Download Li et al. supplementary movie 3(Video)
Video 6.2 MB
Supplementary material: PDF

Li et al. supplementary material

Captions for movies 1-3

Download Li et al. supplementary material(PDF)
PDF 14.4 KB