Article contents
Complex singularities near the intersection of a free surface and wall. Part 1. Vertical jets and rising bubbles
Published online by Cambridge University Press: 04 October 2018
Abstract
It is known that in steady-state potential flows, the separation of a gravity-driven free surface from a solid exhibits a number of peculiar characteristics. For example, it can be shown that the fluid must separate from the body so as to form one of three possible in-fluid angles: (i) 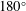 $180^{\circ }$, (ii)
$180^{\circ }$, (ii) 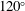 $120^{\circ }$ or (iii) an angle such that the surface is locally perpendicular to the direction of gravity. These necessary separation conditions were notably remarked upon by Dagan & Tulin (J. Fluid Mech., vol. 51 (3), 1972, pp. 529–543) in the context of ship hydrodynamics, but they are of crucial importance in many potential-flow applications. It is not particularly well understood why there is such a drastic change in the local separation behaviours when the global flow is altered. The question that motivates this work is the following: outside of a formal balance-of-terms argument, why must cases (i)–(iii) occur and furthermore, what are the connections between them? In this work, we seek to explain the transitions between the three cases in terms of the singularity structure of the associated solutions once they are extended into the complex plane. A numerical scheme is presented for the analytic continuation of a vertical jet (or alternatively a rising bubble). It will be shown that the transition between the three cases can be predicted by observing the coalescence of singularities as the speed of the jet is modified. A scaling law is derived for the coalescence rate of singularities.
$120^{\circ }$ or (iii) an angle such that the surface is locally perpendicular to the direction of gravity. These necessary separation conditions were notably remarked upon by Dagan & Tulin (J. Fluid Mech., vol. 51 (3), 1972, pp. 529–543) in the context of ship hydrodynamics, but they are of crucial importance in many potential-flow applications. It is not particularly well understood why there is such a drastic change in the local separation behaviours when the global flow is altered. The question that motivates this work is the following: outside of a formal balance-of-terms argument, why must cases (i)–(iii) occur and furthermore, what are the connections between them? In this work, we seek to explain the transitions between the three cases in terms of the singularity structure of the associated solutions once they are extended into the complex plane. A numerical scheme is presented for the analytic continuation of a vertical jet (or alternatively a rising bubble). It will be shown that the transition between the three cases can be predicted by observing the coalescence of singularities as the speed of the jet is modified. A scaling law is derived for the coalescence rate of singularities.
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 4
- Cited by



