Published online by Cambridge University Press: 28 February 2022
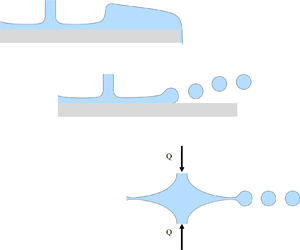
Recently, an unusual scaling law has been observed in circular hydraulic jumps and has been attributed to a supposed missing term in the local energy balance of the flow (Bhagat et al., J. Fluid Mech., vol. 851, 2018, R5). In this paper, we show that – though the experimental observation is valuable and interesting – this interpretation is presumably not the right one. When transposed to the case of an axial sheet formed by two impinging liquid jets, the assumed principle leads in fact to a velocity distribution in contradiction with the present knowledge for this kind of flow. We show here how to correct this approach by maintaining consistency with surface tension thermodynamics: for Savart–Taylor sheets, when adequately corrected, we recover the well-known  $1/r$ liquid thickness with a constant and uniform velocity dictated by Bernoulli's principle. In the case of circular hydraulic jumps, we propose here a simple approach based on Watson's description of the flow in the central region (Watson, J. Fluid Mech., vol. 20, 1964, pp. 481–499), combined with appropriate boundary conditions on the circular front formed. Depending on the specific condition, we find in turn the new scaling by Bhagat et al. (2018) and the more conventional scaling law found long ago by Bohr et al. (J. Fluid Mech., vol. 254, 1993, pp. 635–648). We clarify here a few situations in which one should hold rather than the other, hoping to reconcile the observations of Bhagat et al. with the present knowledge of circular hydraulic jump modelling. However, the question of a possible critical Froude number imposed at the jump exit and dictating logarithmic corrections to scaling remains an open and unsolved question.
$1/r$ liquid thickness with a constant and uniform velocity dictated by Bernoulli's principle. In the case of circular hydraulic jumps, we propose here a simple approach based on Watson's description of the flow in the central region (Watson, J. Fluid Mech., vol. 20, 1964, pp. 481–499), combined with appropriate boundary conditions on the circular front formed. Depending on the specific condition, we find in turn the new scaling by Bhagat et al. (2018) and the more conventional scaling law found long ago by Bohr et al. (J. Fluid Mech., vol. 254, 1993, pp. 635–648). We clarify here a few situations in which one should hold rather than the other, hoping to reconcile the observations of Bhagat et al. with the present knowledge of circular hydraulic jump modelling. However, the question of a possible critical Froude number imposed at the jump exit and dictating logarithmic corrections to scaling remains an open and unsolved question.