Article contents
Characterization of velocity-gradient dynamics in incompressible turbulence using local streamline geometry
Published online by Cambridge University Press: 15 May 2020
Abstract
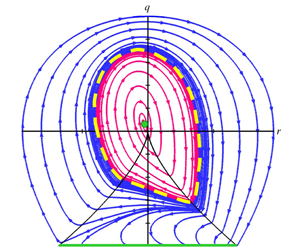
This study develops a comprehensive description of local streamline geometry and uses the resulting shape features to characterize velocity gradient ( $\unicode[STIX]{x1D608}_{ij}=\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{j}$) dynamics. The local streamline geometric shape parameters and scale factor (size) are extracted from
$\unicode[STIX]{x1D608}_{ij}=\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{j}$) dynamics. The local streamline geometric shape parameters and scale factor (size) are extracted from  $\unicode[STIX]{x1D608}_{ij}$ by extending the linearized critical point analysis. In the present analysis,
$\unicode[STIX]{x1D608}_{ij}$ by extending the linearized critical point analysis. In the present analysis,  $\unicode[STIX]{x1D608}_{ij}$ is factorized into its magnitude (
$\unicode[STIX]{x1D608}_{ij}$ is factorized into its magnitude ( $A\equiv \sqrt{\unicode[STIX]{x1D608}_{ij}\unicode[STIX]{x1D608}_{ij}}$) and normalized tensor
$A\equiv \sqrt{\unicode[STIX]{x1D608}_{ij}\unicode[STIX]{x1D608}_{ij}}$) and normalized tensor  $\unicode[STIX]{x1D623}_{ij}\equiv \unicode[STIX]{x1D608}_{ij}/A$. The geometric shape is shown to be determined exclusively by four
$\unicode[STIX]{x1D623}_{ij}\equiv \unicode[STIX]{x1D608}_{ij}/A$. The geometric shape is shown to be determined exclusively by four  $\unicode[STIX]{x1D623}_{ij}$ parameters: second invariant,
$\unicode[STIX]{x1D623}_{ij}$ parameters: second invariant,  $q$ (
$q$ ( $=Q/A^{2}$); third invariant,
$=Q/A^{2}$); third invariant,  $r$ (
$r$ ( $=R/A^{3}$); intermediate strain rate eigenvalue,
$=R/A^{3}$); intermediate strain rate eigenvalue,  $a_{2}$; and vorticity component along intermediate strain rate eigenvector,
$a_{2}$; and vorticity component along intermediate strain rate eigenvector,  $\unicode[STIX]{x1D714}_{2}$. Velocity gradient magnitude,
$\unicode[STIX]{x1D714}_{2}$. Velocity gradient magnitude,  $A$, plays a role only in determining the scale of the local streamline structure. Direct numerical simulation data of forced isotropic turbulence (
$A$, plays a role only in determining the scale of the local streamline structure. Direct numerical simulation data of forced isotropic turbulence ( $Re_{\unicode[STIX]{x1D706}}\sim 200{-}600$) is used to establish streamline shape and scale distribution, and then to characterize velocity-gradient dynamics. Conditional mean trajectories (CMTs) in
$Re_{\unicode[STIX]{x1D706}}\sim 200{-}600$) is used to establish streamline shape and scale distribution, and then to characterize velocity-gradient dynamics. Conditional mean trajectories (CMTs) in  $q$–
$q$– $r$ space reveal important non-local features of pressure and viscous dynamics which are not evident from the
$r$ space reveal important non-local features of pressure and viscous dynamics which are not evident from the  $\unicode[STIX]{x1D608}_{ij}$-invariants. Two distinct types of
$\unicode[STIX]{x1D608}_{ij}$-invariants. Two distinct types of  $q$–
$q$– $r$ CMTs demarcated by a separatrix are identified. The inner trajectories are dominated by inertia–pressure interactions and the viscous effects play a significant role only in the outer trajectories. Dynamical system characterization of inertial, pressure and viscous effects in the
$r$ CMTs demarcated by a separatrix are identified. The inner trajectories are dominated by inertia–pressure interactions and the viscous effects play a significant role only in the outer trajectories. Dynamical system characterization of inertial, pressure and viscous effects in the  $q$–
$q$– $r$ phase space is developed. Additionally, it is shown that the residence time of
$r$ phase space is developed. Additionally, it is shown that the residence time of  $q$–
$q$– $r$ CMTs through different topologies correlate well with the corresponding population fractions. These findings not only lead to improved understanding of non-local dynamics, but also provide an important foundation for developing Lagrangian velocity-gradient models.
$r$ CMTs through different topologies correlate well with the corresponding population fractions. These findings not only lead to improved understanding of non-local dynamics, but also provide an important foundation for developing Lagrangian velocity-gradient models.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 18
- Cited by


