Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Venaille, A.
2012.
Bottom-trapped currents as statistical equilibrium states above topographic anomalies.
Journal of Fluid Mechanics,
Vol. 699,
Issue. ,
p.
500.
Bouchet, Freddy
Laurie, Jason
and
Zaboronski, Oleg
2014.
Langevin Dynamics, Large Deviations and Instantons for the Quasi-Geostrophic Model and Two-Dimensional Euler Equations.
Journal of Statistical Physics,
Vol. 156,
Issue. 6,
p.
1066.
Bouchet, Freddy
Nardini, Cesare
and
Tangarifec, Tomás
2014.
5th Warsaw School of Statistical Physics.
Venaille, Antoine
Nadeau, Louis-Philippe
and
Vallis, Geoffrey
2014.
Ribbon turbulence.
Physics of Fluids,
Vol. 26,
Issue. 12,
Lucarini, Valerio
Blender, Richard
Herbert, Corentin
Ragone, Francesco
Pascale, Salvatore
and
Wouters, Jeroen
2014.
Mathematical and physical ideas for climate science.
Reviews of Geophysics,
Vol. 52,
Issue. 4,
p.
809.
Herbert, Corentin
2014.
Nonlinear energy transfers and phase diagrams for geostrophically balanced rotating-stratified flows.
Physical Review E,
Vol. 89,
Issue. 3,
Qi, W
and
Marston, J B
2014.
Hyperviscosity and statistical equilibria of Euler turbulence on the torus and the sphere.
Journal of Statistical Mechanics: Theory and Experiment,
Vol. 2014,
Issue. 7,
p.
P07020.
Galperin, Boris
Young, Roland M.B.
Sukoriansky, Semion
Dikovskaya, Nadejda
Read, Peter L.
Lancaster, Andrew J.
and
Armstrong, David
2014.
Cassini observations reveal a regime of zonostrophic macroturbulence on Jupiter.
Icarus,
Vol. 229,
Issue. ,
p.
295.
Harper, Katie L.
Nazarenko, Sergey V.
Medvedev, Sergey B.
and
Connaughton, Colm
2014.
Wave turbulence in the two-layer ocean model.
Journal of Fluid Mechanics,
Vol. 756,
Issue. ,
p.
309.
Herbert, Corentin
2015.
Stochastic Equations for Complex Systems.
p.
53.
O’Neill, Morgan E
Emanuel, Kerry A.
and
Flierl, Glenn R.
2016.
Weak Jets and Strong Cyclones: Shallow-Water Modeling of Giant Planet Polar Caps.
Journal of the Atmospheric Sciences,
Vol. 73,
Issue. 4,
p.
1841.
Renaud, A.
Venaille, A.
and
Bouchet, F.
2016.
Equilibrium Statistical Mechanics and Energy Partition for the Shallow Water Model.
Journal of Statistical Physics,
Vol. 163,
Issue. 4,
p.
784.
Chemke, R.
Dror, T.
and
Kaspi, Y.
2016.
Barotropic kinetic energy and enstrophy transfers in the atmosphere.
Geophysical Research Letters,
Vol. 43,
Issue. 14,
p.
7725.
Chemke, R.
2017.
Atmospheric energy transfer response to global warming.
Quarterly Journal of the Royal Meteorological Society,
Vol. 143,
Issue. 706,
p.
2296.
Venaille, A.
Gostiaux, L.
and
Sommeria, J.
2017.
A statistical mechanics approach to mixing in stratified fluids.
Journal of Fluid Mechanics,
Vol. 810,
Issue. ,
p.
554.
Lapeyre, Guillaume
2017.
Surface Quasi-Geostrophy.
Fluids,
Vol. 2,
Issue. 1,
p.
7.
David, Tomos W
Zanna, Laure
and
Marshall, David P
2018.
Eddy-mixing entropy and its maximization in forced-dissipative geostrophic turbulence.
Journal of Statistical Mechanics: Theory and Experiment,
Vol. 2018,
Issue. 7,
p.
073206.
Wang, Yan
and
Stewart, Andrew L.
2018.
Eddy dynamics over continental slopes under retrograde winds: Insights from a model inter-comparison.
Ocean Modelling,
Vol. 121,
Issue. ,
p.
1.
Yassin, Houssam
2021.
Normal modes with boundary dynamics in geophysical fluids.
Journal of Mathematical Physics,
Vol. 62,
Issue. 9,
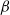 effect, i.e. of the existence of planetary vorticity gradients, is discussed. In particular, it is shown that increasing
effect, i.e. of the existence of planetary vorticity gradients, is discussed. In particular, it is shown that increasing 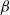 increases the tendency toward barotropization through turbulent stirring. The effectiveness of barotropization may be partially parameterized using the Rhines scale
increases the tendency toward barotropization through turbulent stirring. The effectiveness of barotropization may be partially parameterized using the Rhines scale  . As this parameter decreases (
. As this parameter decreases (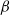 increases) then barotropization can progress further, because the
increases) then barotropization can progress further, because the  term provides enstrophy to each layer. However, if the
term provides enstrophy to each layer. However, if the 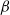 effect is too large then the statistical mechanical predictions fail and wave dynamics prevent complete barotropization.
effect is too large then the statistical mechanical predictions fail and wave dynamics prevent complete barotropization.

