Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-12-01T06:32:53.901Z Has data issue: false hasContentIssue false
  • English
  • Français

Bottom-pressure observations of deep-sea internal hydrostatic and non-hydrostatic motions

Published online by Cambridge University Press:  02 January 2013

Hans van Haren
Hans van Haren*
Affiliation:
NIOZ Royal Netherlands Institute for Sea Research, PO Box 59, 1790 AB Den Burg, the Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the ocean, sloping bottom topography is important for the generation and dissipation of internal waves. Here, the transition of such waves to turbulence is demonstrated using an accurate bottom-pressure sensor that was moored with an acoustic Doppler current profiler and high-resolution thermistor string on the sloping side of the ocean guyot ‘Great Meteor Seamount’ (water depth 549 m). The site is dominated by the passage of strong frontal bores, moving upslope once or twice every tidal period, with a trail of high-frequency internal waves. The bore amplitude and precise timing of bore passage vary every tide. A bore induces mainly non-hydrostatic pressure, while the trailing waves induce mainly internal hydrostatic pressure. These separate (internal wave) pressure terms are independently estimated using current and temperature data, respectively. In the bottom-pressure time series, the passage of a bore is barely visible, but the trailing high-frequency internal waves are. A bore is obscured by higher-frequency pressure variations up to 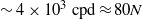${\sim} 4{\times} 1{0}^{3} ~\mathrm{cpd} \approx 80N$ (cpd, cycles per day; 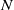$N$, the large-scale buoyancy frequency). These motions dominate the turbulent state of internal tides above a sloping bottom. In contrast with previous bottom-pressure observations in other areas, infra-gravity surface waves contribute little to these pressure variations in the same frequency range. Here, such waves do not incur observed pressure. This is verified in a consistency test for large-Reynolds-number turbulence using high-resolution temperature data. The high-frequency quasi-turbulent internal motions are visible in detailed temperature and acoustic echo images, revealing a nearly permanently wave-turbulent tide going up and down the bottom slope. Over the entire observational period, the spectral slope and variance of bottom pressure are equivalent to internal hydrostatic pressure due to internal waves in the lower 100 m above the bottom, by non-hydrostatic pressure due to high-frequency internal waves and large-scale overturning. The observations suggest a transition between large-scale internal waves, small-scale internal tidal waves residing on thin (${{\sim} }1~\mathrm{m} $) stratified layers and turbulence.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Ocean processes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 714 , 10 January 2013 , pp. 591 - 611
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aghsaee, P., Boegman, L. & Lamb, K. G. 2010 Breaking of shoaling internal solitary waves. J. Fluid Mech. 659, 289–317.Google Scholar
Bonnin, J., van Haren, H., Hosegood, P. & Brummer, G.-J. A. 2006 Burst resuspension of seabed material at the foot of the continental slope in the Rockall Channel. Mar. Geol. 226, 167–184.Google Scholar
Bromirski, P. D., Sergienko, O. V. & MacAyeal, D. R. 2010 Transoceanic infragravity waves impacting Antarctic ice shelves. Geophys. Res. Lett. 37, L02502.Google Scholar
Cuxart, J., Morales, G., Terradales, E. & Yagüe, C. 2002 Study of coherent structures and estimation of the pressure transport terms for the nocturnal stable boundary layer. Boundary-Layer Meteorol. 105, 305–328.CrossRefGoogle Scholar
D’Asaro, E. A. & Lien, R.-C. 2000a Lagrangian measurements of waves and turbulence in stratified flows. J. Phys. Oceanogr. 30, 641–655.Google Scholar
D’Asaro, E. A. & Lien, R.-C. 2000b The wave–turbulence transition for stratified flows. J. Phys. Oceanogr. 30, 1669–1678.2.0.CO;2>CrossRefGoogle Scholar
Filloux, J. H. 1980 Pressure fluctuations on the open-ocean floor over a broad frequency range: new program and early results. J. Phys. Oceanogr. 10, 1959–1971.Google Scholar
Fructus, D., Carr, M., Grue, J., Jensen, A. & Davies, P. A. 2009 Shear-induced breaking of large internal solitary waves. J. Fluid Mech. 620, 1–29.Google Scholar
Gayen, B. & Sarkar, S. 2010 Turbulence during the generation of internal tide on a critical slope. Phys. Rev. Lett. 104, 218502.Google Scholar
Gerkema, T., Zimmerman, J. T. F., Maas, L. R. M. & van Haren, H. 2008 Geophysical and astrophysical fluid dynamics beyond the traditional approximation. Rev. Geophys. 46, RG2004.Google Scholar
Gotoh, T. & Fukayama, D. 2001 Pressure spectrum in homogeneous turbulence. Phys. Rev. Lett. 86, 3775–3778.Google Scholar
Grue, J. 2005 Generation, ppropagation and breaking of internal solitary waves. Chaos 15, 037110.Google Scholar
Grue, J., Jensen, A., Rusås, P.-O. & Sveen, J. K. 2000 Breaking and broadening of internal solitary waves. J. Fluid Mech. 413, 181–217.Google Scholar
Grue, J. & Sveen, J. K. 2010 A scaling law of internal run-up duration. Ocean Dyn. 60, 993–1006.Google Scholar
Hosegood, P. & van Haren, H. 2004 Near-bed solibores over the continental slope in the Faeroe–Shetland Channel. Deep-Sea Res. II 51, 2943–2971.Google Scholar
Klymak, J. M. & Moum, J. N. 2003 Internal solitary waves of elevation advancing on a shoaling shelf. Geophys. Res. Lett. 30, 2045.Google Scholar
Lamb, K. G. 2003 Shoaling solitary internal waves: on a criterion for the formation of waves with trapped cores. J. Fluid Mech. 478, 81–100.Google Scholar
Lamb, K. G. & Farmer, D. 2011 Instabilities in an internal solitary-like wave on the Oregon shelf. J. Phys. Oceanogr. 41, 67–87.CrossRefGoogle Scholar
LeBlond, P. H. & Mysak, L. A. 1978 Waves in the Ocean. Elsevier.Google Scholar
Moum, J. N. & Smyth, W. D. 2006 The pressure disturbance of a nonlinear internal wave train. J. Fluid Mech. 558, 153–177.Google Scholar
Moum, J. N. & Nash, J. D. 2008 Seafloor pressure measurements of nonlinear internal waves. J. Phys. Oceanogr. 38, 481–491.Google Scholar
Okihiro, M. & Guza, R. T. 1995 Infragravity energy modulation by tides. J. Geophys. Res. 100, 16 143–16 148.Google Scholar
Perlin, A., Moum, J. N., Klymak, J. M., Levine, M. D., Boyd, T. & Kosro, M. H. 2005 A modified law-of-the-wall applied to oceanic boundary layers. J. Geophys. Res. 110, C10S10.Google Scholar
Pinkel, R. 1981 Observations of the near-surface internal wavefield. J. Phys. Oceanogr. 11, 1248–1257.Google Scholar
Shaw, R. H., Paw, K. T., Zhang, X. J., Gao, W., den Hartog, G. & Neumann, H. H. 1990 Retrieval of turbulent pressure fluctuations at the ground surface beneath a forest. Boundary-Layer Meteorol. 50, 319–338.CrossRefGoogle Scholar
Slinn, D. N. & Riley, J. J. 1996 Turbulent mixing in the oceanic boundary layer caused by internal wave reflection from sloping terrain. Dyn. Atmos. Oceans 24, 51–62.Google Scholar
Smith, W. H. F. & Sandwell, D. T. 1997 Global seafloor topography from satellite altimetry and ship depth soundings. Science 277, 1957–1962.Google Scholar
Thomas, A. S. W. & Bull, M. K. 1983 On the role of wall-pressure fluctuations in deterministic motions in the turbulent boundary layer. J. Fluid Mech. 128, 283–322.Google Scholar
Thorpe, S. A. 1977 Turbulence and mixing in a Scottish loch. Phil. Trans. R. Soc. Lond. A 286, 125–181.Google Scholar
Tsuji, Y., Fransson, J. H. M., Alfredsson, P. H. & Johansson, A. V. 2007 Pressure statistics and their scaling in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 585, 1–40.Google Scholar
Tsuji, Y. & Ishihara, T. 2003 Similarity scaling of pressure fluctuation in turbulence. Phys. Rev. E 68, 026309.Google Scholar
van Haren, H. 2009 High-frequency vertical current observations in stratified seas and ocean. Cont. Shelf Res. 29, 1251–1263.Google Scholar
van Haren, H. 2011 Internal wave-turbulence pressure above sloping sea bottoms. J. Geophys. Res 116, C12004.Google Scholar
van Haren, H. & Gostiaux, L. 2009 High-resolution open-ocean temperature spectra. J. Geophys. Res. 114, C05005.Google Scholar
van Haren, H. & Gostiaux, L. 2010 A deep-ocean Kelvin–Helmholtz billow train. Geophys. Res. Lett. 37, L03605.Google Scholar
van Haren, H. & Gostiaux, L. 2012a Detailed internal wave mixing above a deep-ocean slope. J. Mar. Res. 70, 179–197.Google Scholar
van Haren, H. & Gostiaux, L. 2012b Energy release through internal wave breaking. Oceanography 25 (2), 124–131.Google Scholar
van Haren, H., Laan, M., Buijsman, D.-J., Gostiaux, L., Smit, M. G. & Keijzer, E. 2009 NIOZ3: independent temperature sensors sampling yearlong data at a rate of 1 Hz. IEEE J. Ocean. Engng 34, 315–322.Google Scholar
Venayagamoorthy, S. K. & Fringer, O. B. 2007 On the formation and propagation of nonlinear internal boluses across a shelf break. J. Fluid Mech. 577, 137–159.Google Scholar
Vlasenko, V. & Hutter, K. 2002 Numerical experiments on the breaking of solitary internal waves over a slope-shelf topography. J. Phys. Oceanogr. 32, 1779–1793.Google Scholar
Webb, S. C. 1998 Broadband seismology and noise under the ocean. Rev. Geophys. 36, 105–142.Google Scholar
Willmarth, W. W. 1975 Pressure fluctuations beneath turbulent boundary layers. Annu. Rev. Fluid Mech. 7, 13–36.Google Scholar
Wyngaard, J. C. 1973 On surface layer turbulence. In Workshop on Micrometeorology (ed. Haugen, D. A.). pp. 101–149. AMS.Google Scholar
Xing, J. & Davies, A. M. 2006 Processes influencing tidal mixing in the region of sills. Geophys. Res. Lett 33, L04603.CrossRefGoogle Scholar