Article contents
Axisymmetric internal wave transmission and resonant interference in nonlinear stratifications
Published online by Cambridge University Press: 10 January 2020
Abstract
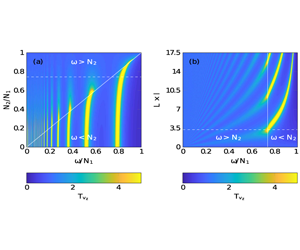
To date, the influence of nonlinear stratifications and two layer stratifications on internal wave propagation has been studied for two-dimensional wave fields in a Cartesian geometry. Here, we use a novel wave generator configuration to investigate transmission in nonlinear stratifications of an axisymmetric internal wave. We demonstrate that, despite the additional geometric complexity, with associated features such as an inhomogeneous spatial distribution of the energy flux, results for plane waves can be generalised to axisymmetric wave fields. Two configurations are studied, both theoretically and experimentally. In the case of a free incident wave, a transmission maximum is found in the vicinity of evanescent frequencies. In the case of a confined incident wave, resonant effects, in the sense of constructive interference, lead to enhanced transmission rates from an upper layer to a layer below. We consider the oceanographic relevance of these results by applying them to an example oceanic stratification, finding that there can be real-world implications.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 6
- Cited by


