Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
McKeon, B. J.
2020.
A basis for flow modelling.
Journal of Fluid Mechanics,
Vol. 904,
Issue. ,
Farghadan, Ali
Towne, Aaron
Martini, Eduardo
and
Cavalieri, Andrè
2021.
A randomized time-domain algorithm for efficiently computing resolvent modes.
Rosenberg, Kevin T.
Medina, Albert
Wang, Tso-Kang
and
Shoele, Kourosh
2021.
Resolvent Analysis of Morphing Bodies.
Jin, Bo
Symon, Sean
and
Illingworth, Simon J.
2021.
Energy transfer mechanisms and resolvent analysis in the cylinder wake.
Physical Review Fluids,
Vol. 6,
Issue. 2,
Herrmann, Benjamin
Baddoo, Peter J.
Semaan, Richard
Brunton, Steven L.
and
McKeon, Beverley J.
2021.
Data-driven resolvent analysis.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Tamilselvam, Prashanth
Asztalos, Katherine J.
and
Dawson, Scott T.
2022.
Transient growth analysis of flow over an airfoil for identifying high-amplification, spatially-localized inputs.
Wu, Wen
Meneveau, Charles
Mittal, Rajat
Padovan, Alberto
Rowley, Clarence W.
and
Cattafesta, Louis
2022.
Response of a turbulent separation bubble to zero-net-mass-flux jet perturbations.
Physical Review Fluids,
Vol. 7,
Issue. 8,
Padovan, Alberto
and
Rowley, Clarence W.
2022.
Analysis of the dynamics of subharmonic flow structures via the harmonic resolvent: Application to vortex pairing in an axisymmetric jet.
Physical Review Fluids,
Vol. 7,
Issue. 7,
Skene, Calum S.
Yeh, Chi-An
Schmid, Peter J.
and
Taira, Kunihiko
2022.
Sparsifying the resolvent forcing mode via gradient-based optimisation.
Journal of Fluid Mechanics,
Vol. 944,
Issue. ,
Franceschini, Lucas
Sipp, Denis
Marquet, Olivier
Moulin, Johann
and
Dandois, Julien
2022.
Identification and reconstruction of high-frequency fluctuations evolving on a low-frequency periodic limit cycle: application to turbulent cylinder flow.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Kantarakias, Kyriakos D.
and
Papadakis, George
2023.
Sensitivity analysis of chaotic systems using a frequency-domain shadowing approach.
Journal of Computational Physics,
Vol. 474,
Issue. ,
p.
111757.
Herrmann, Benjamin
Baddoo, Peter J.
Dawson, Scott T.M.
Semaan, Richard
Brunton, Steven L.
and
McKeon, Beverley J.
2023.
Interpolatory input and output projections for flow control.
Journal of Fluid Mechanics,
Vol. 971,
Issue. ,
Callaham, Jared L.
Loiseau, Jean-Christophe
and
Brunton, Steven L.
2023.
Multiscale model reduction for incompressible flows.
Journal of Fluid Mechanics,
Vol. 973,
Issue. ,
Unnikrishnan, S.
2023.
Recent advances in feature extraction techniques for high-speed flowfields.
Progress in Aerospace Sciences,
Vol. 140,
Issue. ,
p.
100918.
Liu, Chang
Shuai, Yu
Rath, Aishwarya
and
Gayme, Dennice F.
2023.
A structured input-output approach to characterizing optimal perturbations in wall-bounded shear flows.
p.
2319.
Lopez-Doriga, Barbara
Ballouz, Eric
Bae, Hyunji Jane
and
Dawson, Scott T.
2023.
A sparsity-promoting resolvent analysis for the identification of spatiotemporally-localized amplification mechanisms.
Ballouz, Eric
Lopez-Doriga, Barbara
Dawson, Scott T.
and
Bae, Hyunji Jane
2023.
Wavelet-based resolvent analysis for statistically-stationary and temporally-evolving flows.
Leclercq, Colin
and
Sipp, Denis
2023.
Mean resolvent operator of a statistically steady flow.
Journal of Fluid Mechanics,
Vol. 968,
Issue. ,
Lin, Ching-Te
Tsai, Min-Lin
and
Tsai, Hsieh-Chen
2023.
Flow control of a plunging cylinder based on resolvent analysis.
Journal of Fluid Mechanics,
Vol. 967,
Issue. ,
Padovan, Alberto
and
Rowley, Clarence W.
2024.
Continuous-time balanced truncation for time-periodic fluid flows using frequential Gramians.
Journal of Computational Physics,
Vol. 496,
Issue. ,
p.
112597.
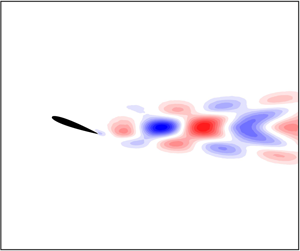
 $\omega$ are coupled with perturbations at frequency
$\omega$ are coupled with perturbations at frequency  $\alpha$ through the base flow at frequency
$\alpha$ through the base flow at frequency  $\omega -\alpha$. We draw a connection with resolvent analysis, which is a special case of the harmonic resolvent when evaluated about a steady base flow. We show that the left and right singular vectors of the harmonic resolvent are the optimal response and forcing modes, which can be understood as full spatio-temporal signals that reveal space–time amplification characteristics of the flow. Finally, we illustrate the method on examples, including a three-dimensional system of ordinary differential equations and the flow over an airfoil at near-stall angle of attack.
$\omega -\alpha$. We draw a connection with resolvent analysis, which is a special case of the harmonic resolvent when evaluated about a steady base flow. We show that the left and right singular vectors of the harmonic resolvent are the optimal response and forcing modes, which can be understood as full spatio-temporal signals that reveal space–time amplification characteristics of the flow. Finally, we illustrate the method on examples, including a three-dimensional system of ordinary differential equations and the flow over an airfoil at near-stall angle of attack.

