Article contents
Problems in the Theory of Optimal Capital Structure
Published online by Cambridge University Press: 19 October 2009
Extract
This paper considers several related problems in the theory of optimal capital structure for corporations. It is divided into four sections, which may be briefly summarized as follows.
1. Modigliani and Miller (MM) proposed that under the assumption of perfect markets and in the absence of taxes on corporate income, the total market value of the firm is unaffected by leverage. They showed that the leverage irrelevance proposition holds for “non-growth” firms when all investors agree in their estimates of the expected amount and the risk of each firm's future earnings. In section I, we show that this conclusion is not affected by growth trends or heterogeneous investor expectations. However, our analysis uncovers several additional assumptions which must be made explicitly for MM's Proposition I to hold. These additional assumptions pertain to the effects of leverage on the firm's future financing needs and future investment decisions. The generalized state-preference framework used for this demonstration is retained for subsequent discussion.
- Type
- Research Article
- Information
- Copyright
- Copyright © School of Business Administration, University of Washington 1966
References
1 Modigliani, F. and Miller, M. H., “The Cost of Capital, Corporation Finance and the Theory of Investment,” American Economic Review, XLVIII, No. 3 (June 1958), pp. 261–297Google Scholar, reprinted in The Management of Corporate Capital, Solomon, E. (ed.), (New York: Free Press of Glencoe, 1959).Google Scholar Page references in subsequent footnotes are to the latter volume.
2 Ibid.
3 Modigliani and Miller presented a preliminary test of their propositions. Op. cit., pp. 167–73, see also Barges, A., The Effects of Capital Structure on the Cost of Capital (Englewood Cliffs, N. J.: Prentice-Hall, Inc., 1963Google Scholar).
4 For a discussion of these assumptions, see Bodenhorn, D., “On the Problem of Capital Budgeting,” Journal of Finance, XIV, No. 4 (December 1959), pp. 473–492Google Scholar, and Durand, D., “The Cost of Capital in an Imperfect Market: A Reply to Modigliani and Miller,“ American Economic Review, XLIX, No. 4 (September 1959), pp. 646–655.Google Scholar
5 Modigliani and Miller, p. 156.
6 Ibid., pp. 153–55.
7 The dividend policy followed is regarded as irrelevant. See Miller, M. H. and Modigliani, F., “Dividend Policy, Growth and the Valuation or Shares,” Journal of Business, XXXIV, No. 4 (October 1961), pp. 411–433.CrossRefGoogle Scholar
8 That part of this paper which uses this framework directly has been drawn from a dissertation now in preparation at the Graduate School of Business, Stanford University: Stewart C. Myers, “Effects of Uncertainty on the Valuation of Corporate Securities and the Financial Decisions of the Firm.”
9 Arrow, K., “The Role of Securities in the Optimal Allocation of Risk Bearing,” Review of Economic Studies, Vol. 31 (1963–1964), pp. 91–96Google Scholar. For examples of related work, see Debreu, G., The Theory of Value (New York: John Wiley & Sons, 1959Google Scholar), esp. Ch. 7, and R. Radner, Competitive Equilibrium Under Uncertainty, Technical Report No. 26, Office of Naval Research, 1965.
10 Hirshleifer, J., “investment Decision Under Uncertainty: Choice-Theoretic Approaches,” Quarterly Journal of Economics, LXXIX, 4 (November 1965), pp. 509–536CrossRefGoogle Scholar and J. Hirschleifer, “Investment Decision Under Uncertainty: Application of the State-Preference Approach, ” Quarterly Journal of Economics, forthcoming.
11 For a more detailed formal discussion, see Myers, Ch. 2. Some observations on the practical interpretations of these concepts are presented in Robichek, A. A. and Myers, S. C., “Valuation of the Firm: Effects of Uncertainty in a Market Context,” Journal of Finance (May 1966).Google Scholar
12 This point is made by Hirshleifer in his November 1965 article cited above.
13 see their article, “Dividend Policy, Growth, and the Valuation of Shares,” already cited.
14 For example, if the firm borrows from a bank at some future time T, the effect will be a positive increment at ![]() and negative increments to
and negative increments to ![]() , in subsequent periods as the bank receives interest and principal repayments from the firm.
, in subsequent periods as the bank receives interest and principal repayments from the firm.
15 This assumption is for notational and expositional convenience. This “horizon” may conceiva.bly be very distant. In any case, any errors in valuation resulting from neglecting returns which may be received after this horizon period may be reduced to an arbitrarily small amount by extending the horizon.
16 Hirshleifer, op. cit.
17 This assumption was made by Hirshleifer (op. cit.) in his exposition of this framework. For a, formal discussion of the conditions necessary to justify it, see Myers, op. cit., Ch. 2, Roughly speaking, this assumption is justifiable if the number of available securities is at least as great as the number of state-time combinations under consideration.
18 See The Cost of Capital, Corporation Finance, and the Theory of Investment, pp. 155–56.
19 In particular see F. Modigliani and Miller, M. H., “Corporate Income Taxes end the Cost of Capital: A Correction,” American Economic Review, LIII, No. 3 (June 1963), pp. 433–442.Google Scholar
20 This sort of a world is an illuminating hypothetical case for purposes of exposition. However, see footnote 17, above, for reservations regarding its descriptive usefulness.
21 Since increases in leverage will always reduce taxable income in at least some state-time combinations, the exact size of the rebate attainable depends on all the ins and outs of tax laws.
22 Normally ![]() would be zero also, but not necessarily. For instance, a firm might promise to make a repayment of principal to creditors which is greater than its accounting income in a. particular state-time combination. This would not excuse the firm from paying a corporate income tax.
would be zero also, but not necessarily. For instance, a firm might promise to make a repayment of principal to creditors which is greater than its accounting income in a. particular state-time combination. This would not excuse the firm from paying a corporate income tax.
23 If reorganization occurs in some future contingency, the division of the remaining value of the firm between present holders of the firm's securities is also uncertain. However, present investors can hedge against this risk by holding both the bonds and stock of the corporation in their portfolios. The formal structure of our discussion implicitly assumes that investors will not be reluctant to purchase securities of leveraged corporations because of the necessity of hedging if this risk is to be avoided. They may be reluctant in practice, however, in which case this risk would be another indirect cost of bankruptcy from the viewpoint of present shareholders.
24 A recent series of articles has explored the rationality of capital rationing. For example, see Hodgman, D. R., “Credit Risk and Credit Rationing,” Quarterly Journal of Economics, LXXIV (May 1960), pp. 258–278.CrossRefGoogle Scholar
25 Note that if we solve Eq. (24) for ![]() , and if Vj and Zj are independent of leverage, the result is exactly analagous to MM's Proposition II. See “The Cost of Capital, Corporation Finance, and the Theory of Investment,” p. 158. Our variable Zj is thus directly analagous to MM's “capitalization rate” Pj
, and if Vj and Zj are independent of leverage, the result is exactly analagous to MM's Proposition II. See “The Cost of Capital, Corporation Finance, and the Theory of Investment,” p. 158. Our variable Zj is thus directly analagous to MM's “capitalization rate” Pj
26 If no investors believe the two firms to be in equivalent risk classes, then equilibrium is clearly possible if Zj ≠ Zj. However, it is not necessary for all investors to consider the risk classes equivalent to insure that Proposition I holds.
27 See The Cost of Capital, Corporation Finance, and the Theory of Investment, pp. 155–56.
28 See, for instance, Durand, op. cit.
29 To see this, note that the expected average income of the jth firm, ![]() , can be given by
, can be given by 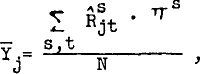 where πs is the probability of occurrence of a particular state combina.tion, and N is the number of time periods under consideration.
where πs is the probability of occurrence of a particular state combina.tion, and N is the number of time periods under consideration.
![]() ,
, 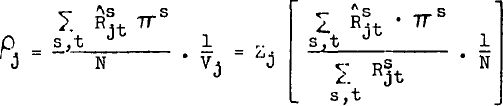
30 Actually, ![]() would be a more precise indicator of what we normally mean by “maturity structure.” But the differences between the set of promised payments and the set of actual payments will normally be rare enough that the maturity structure of the two sets will be substantially the same.
would be a more precise indicator of what we normally mean by “maturity structure.” But the differences between the set of promised payments and the set of actual payments will normally be rare enough that the maturity structure of the two sets will be substantially the same.
31 Claims to physical assets in event of default do not necessarily affect the total contingent payment to debt as a whole. One class of debt might benefit at the expense of another class, without changing the total payment to bondholders.
- 137
- Cited by


