Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T19:59:17.423Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak and strong law results for a function of the spacings

Published online by Cambridge University Press:  14 July 2016

William P. McCormick
William P. McCormick*
Affiliation:
University of Georgia
*
∗Postal address: Department of Statistics and Computer Science, Graduate Studies Building, The University of Georgia, Athens, GA 30602, USA.
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 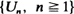be i.i.d. uniform on (0,1) random variables and define Si,n = Ui,n–1Ui–1,n–1, i = 1, · ··, n where the Ui–n–1 are the order statistics from a sample of size n – 1 and U0,n–1 =0 and Un,n–1 = 1. The Si,n are called the spacings divided by U1,· ··,Un–1. For a fixed integer l, set 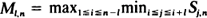. Exact and weak limit results are obtained for the Ml,n. Further we show that with probability 1 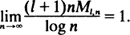This extends results of Cheng.

Keywords

ORDER STATISTICSPACINGSLIMITING DISTRIBUTION
Type
Research Papers
Information
Journal of Applied Probability , Volume 22 , Issue 3 , September 1985 , pp. 543 - 555
Copyright
Copyright © Applied Probability Trust 1985 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research supported by the National Science Foundation under Grant MCS8202259 and by AFOSR Grant No. F49620 82 C 0009.

References

[1] Balkema, A. A. and De Haan, L. (1978) Limit distributions for order statistics. I. Theory Prob. Appl. 23, 77–92.CrossRefGoogle Scholar
[2] Balkema, A. A. and De Haan, L. (1978) Limit distributions for order statistics. II. Theory Prob. Appl. 23, 341–358.Google Scholar
[3] Bender, E. A. (1974) Asymptotic methods in enumeration. SIAM Review 16, 485–515.Google Scholar
[4] Canfield, E. R. and Mccormick, W. P. (1983) Exact and limiting distributions for sustained maxima. J. Appl. Prob. 20, 803–813.CrossRefGoogle Scholar
[5] Cheng, S. (1983) On a problem concerning spacings. Center for Stochastic Processes Tech. Rept. # 27, Univ. of North Carolina, Chapel Hill, N.C. Google Scholar
[6] Chow, Y. S., Geman, S. and Wu, L. D. (1983) Consistent cross-validated density estimation. Ann. Statist. 11, 25–38.Google Scholar
[7] Leadbetter, M. R., Lindgren, G. and Rootzen, H. (1983) Extremes and Related Properties of Random Sequences and Processes. Springer-Verlag, New York.Google Scholar
[8] Marron, J. S. (1983) An asymptotic efficient solution to the bandwidth problem of kernel density estimation. Inst. Stat. Mimeo Series # 1518, Univ. of North Carolina, Chapel Hill, N.C. Google Scholar
[9] Pyke, R. (1965) Spacings. J. R. Statist. Soc. B 27, 395–436.Google Scholar