Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-28T06:08:41.887Z Has data issue: false hasContentIssue false
  • English
  • Français

Renewal theory for iterated perturbed random walks on a general branching process tree: intermediate generations

Part of: Stochastic processes Markov processes Special processes

Published online by Cambridge University Press:  18 February 2022

Vladyslav Bohun ,
Alexander Iksanov Open the ORCID record for Alexander Iksanov [Opens in a new window] ,
Alexander Marynych Open the ORCID record for Alexander Marynych [Opens in a new window]  and
Bohdan Rashytov
Vladyslav Bohun*
Affiliation:
Taras Shevchenko National University of Kyiv
Alexander Iksanov*
Affiliation:
Taras Shevchenko National University of Kyiv
Alexander Marynych*
Affiliation:
Taras Shevchenko National University of Kyiv
Bohdan Rashytov*
Affiliation:
Taras Shevchenko National University of Kyiv
*
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, 01601 Ukraine.
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, 01601 Ukraine.
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, 01601 Ukraine.
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, 01601 Ukraine.
Get access Rights & Permissions [Opens in a new window]

Abstract

An iterated perturbed random walk is a sequence of point processes defined by the birth times of individuals in subsequent generations of a general branching process provided that the birth times of the first generation individuals are given by a perturbed random walk. We prove counterparts of the classical renewal-theoretic results (the elementary renewal theorem, Blackwell’s theorem, and the key renewal theorem) for the number of jth-generation individuals with birth times 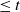$\leq t$ , when 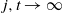$j,t\to\infty$ and 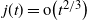$j(t)={\textrm{o}}\big(t^{2/3}\big)$ . According to our terminology, such generations form a subset of the set of intermediate generations.

Keywords

Convolutionkey renewal theoremperturbed random walkrenewal theory

MSC classification

Primary: 60K05: Renewal theory 60J80: Branching processes (Galton-Watson, birth-and-death, etc.)
Secondary: 60G05: Foundations of stochastic processes
Type
Original Article
Information
Journal of Applied Probability , Volume 59 , Issue 2 , June 2022 , pp. 421 - 446
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alsmeyer, G., Iksanov, A. and Marynych, A. (2017). Functional limit theorems for the number of occupied boxes in the Bernoulli sieve. Stoch. Proc. Appl. 127, 995–1017.10.1016/j.spa.2016.07.007CrossRefGoogle Scholar
Asmussen, S. (2003). Applied Probability and Queues, 2nd edn. Springer.Google Scholar
Biggins, J. D. (1977). Chernoff’s theorem in the branching random walk. J. Appl. Prob. 14, 630–636.10.2307/3213469CrossRefGoogle Scholar
Biggins, J. D. (1979). Growth rates in the branching random walk. Z. Wahrscheinlichkeitsth. 48, 17–34.CrossRefGoogle Scholar
Biggins, J. D. (1992). Uniform convergence of martingales in the branching random walk. Ann. Prob. 20, 137–151.10.1214/aop/1176989921CrossRefGoogle Scholar
Buraczewski, D., Dovgay, B. and Iksanov, A. (2020). On intermediate levels of nested occupancy scheme in random environment generated by stick-breaking I. Electron. J. Prob. 25, 123.CrossRefGoogle Scholar
Carlsson, H. and Nerman, O. (1986). An alternative proof of Lorden’s renewal inequality. Adv. Appl. Prob. 18, 1015–1016.CrossRefGoogle Scholar
Dong, C. and Iksanov, A. (2020). Weak convergence of random processes with immigration at random times. J. Appl. Prob. 57, 250–265.10.1017/jpr.2019.88CrossRefGoogle Scholar
Duchamps, J.-J., Pitman, J. and Tang, W. (2019). Renewal sequences and record chains related to multiple zeta sums. Trans. Amer. Math. Soc. 371, 5731–5755.CrossRefGoogle Scholar
Frenk, J. B. G. (1987). On Banach Algebras, Renewal Measures and Regenerative Processes (CW Tract 38). Centre for Mathematics and Computer Science, Amsterdam.Google Scholar
Gnedin, A., Hansen, A. and Pitman, J. (2007). Notes on the occupancy problem with infinitely many boxes: general asymptotics and power laws. Prob. Surv. 4, 146–171.10.1214/07-PS092CrossRefGoogle Scholar
Gut, A. (2009). Stopped Random Walks: Limit Theorems and Applications, 2nd edn. Springer.CrossRefGoogle Scholar
Holmgren, C. and Janson, S. (2017). Fringe trees, Crump–Mode–Jagers branching processes and m-ary search trees. Prob. Surv. 14, 53–154.Google Scholar
Iksanov, A. (2016). Renewal Theory for Perturbed Random Walks and Similar Processes. Birkhäuser.CrossRefGoogle Scholar
Iksanov, A. and Rashytov, B. (2020). A functional limit theorem for general shot noise processes. J. Appl. Prob. 57, 280–294.10.1017/jpr.2019.95CrossRefGoogle Scholar
Iksanov, A., Marynych, A. and Samoilenko, I. (2020). On intermediate levels of nested occupancy scheme in random environment generated by stick-breaking II. Available at arXiv:2011.12231.Google Scholar
Iksanov, A., Pilipenko, A. and Samoilenko, I. (2017). Functional limit theorems for the maxima of perturbed random walks and divergent perpetuities in the M1-topology. Extremes 20, 567–583.CrossRefGoogle Scholar
Iksanov, A., Rashytov, B. and Samoilenko, I. (2021). Renewal theory for iterated perturbed random walks on a general branching process tree: early levels. Available at arXiv:2105.02846.Google Scholar
Karlin, S. (1967). Central limit theorems for certain infinite urn schemes. J. Math. Mech. 17, 373–401.Google Scholar
Mitov, K. V. and Omey, E. (2014). Renewal Processes. Springer.CrossRefGoogle Scholar
Mohan, N. R. (1976). Teugels’ renewal theorem and stable laws. Ann. Prob. 4, 863–868.CrossRefGoogle Scholar
Pitman, J. and Tang, W. (2019). Regenerative random permutations of integers. Ann. Prob. 47, 1378–1416.CrossRefGoogle Scholar
Pitman, J. and Yakubovich, Yu. (2019). Gaps and interleaving of point processes in sampling from a residual allocation model. Bernoulli 25, 3623–3651.10.3150/19-BEJ1104CrossRefGoogle Scholar
Rashytov, B. (2018). Power moments of first passage times for some oscillating perturbed random walks. Theory Stoch. Proc. 23, 93–97.Google Scholar
Resnick, S. I. (2002). Adventures in Stochastic Processes, 3rd printing. Birkhäuser.Google Scholar
Rudin, W. (1962). Fourier Analysis on Groups. John Wiley.Google Scholar
Sen, P. K. (1981). Weak convergence of an iterated renewal process. J. Appl. Prob. 18, 291–296.10.2307/3213191CrossRefGoogle Scholar
Sgibnev, M. S. (1982). Renewal theorem in the case of an infinite variance. Sib. Math. J. 22, 787–796.CrossRefGoogle Scholar