Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hudson, R. L.
1973.
A quantum-mechanical central limit theorem for anti-commuting observables.
Journal of Applied Probability,
Vol. 10,
Issue. 3,
p.
502.
Hudson, R.L.
1974.
When is the wigner quasi-probability density non-negative?.
Reports on Mathematical Physics,
Vol. 6,
Issue. 2,
p.
249.
Srinivas, M. D.
and
Wolf, E.
1975.
Some nonclassical features of phase-space representations of quantum mechanics.
Physical Review D,
Vol. 11,
Issue. 6,
p.
1477.
Cockroft, A.M.
and
Hudson, R.L.
1977.
Quantum mechanical Wiener processes.
Journal of Multivariate Analysis,
Vol. 7,
Issue. 1,
p.
107.
Cockroft, A.M.
Gudder, S.P.
and
Hudson, R.L.
1977.
A quantum-mechanical functional central limit theorem.
Journal of Multivariate Analysis,
Vol. 7,
Issue. 1,
p.
125.
Gudder, S. P.
and
Hudson, R. L.
1978.
A noncommutative probability theory.
Transactions of the American Mathematical Society,
Vol. 245,
Issue. 0,
p.
1.
Giri, N.
and
Waldenfels, W.
1978.
An algebraic version of the central limit theorem.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 42,
Issue. 2,
p.
129.
Hudson, R. L.
and
Peck, S. N.
1979.
Canonical Fourier transforms.
Journal of Mathematical Physics,
Vol. 20,
Issue. 1,
p.
114.
Hudson, R.L.
1979.
The strong Markov property for canonical Wiener processes.
Journal of Functional Analysis,
Vol. 34,
Issue. 2,
p.
266.
Hudson, R.L
Wilkinson, M.D
and
Peck, S.N
1980.
Translation-invariant integrals, and Fourier analysis on Clifford and Grassmann algebras.
Journal of Functional Analysis,
Vol. 37,
Issue. 1,
p.
68.
Drisch, Thomas
1980.
Die S�tze von Bochner und L�vy f�r Gleason-Ma�e.
Archiv der Mathematik,
Vol. 34,
Issue. 1,
p.
60.
Drisch, Thomas
1980.
Zur Realisierung unabh�ngiger kanonischer Paare.
Archiv der Mathematik,
Vol. 34,
Issue. 1,
p.
357.
Farina, J. E. G.
1982.
Variance and covariance in quantum mechanics and the spreading of position probability.
International Journal of Theoretical Physics,
Vol. 21,
Issue. 2,
p.
83.
Guz, W.
1984.
Foundations of phase-space quantum mechanics.
International Journal of Theoretical Physics,
Vol. 23,
Issue. 2,
p.
157.
Accardi, L.
and
Bach, A.
1985.
Quantum central limit theorems for strongly mixing random variables.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 68,
Issue. 3,
p.
393.
Mourgues, G.
Feix, M. R.
Andrieux, J. C.
and
Bertrand, P.
1985.
Not necessary but sufficient condition for the positivity of generalized Wigner functions.
Journal of Mathematical Physics,
Vol. 26,
Issue. 10,
p.
2554.
Hudson, R.L
and
Lindsay, J.M
1985.
A non-commutative martingale representation theorem for non-Fock quantum Brownian motion.
Journal of Functional Analysis,
Vol. 61,
Issue. 2,
p.
202.
Kaaz, Mieczyslaw Albert
1987.
Concerning a quantum-like uncertainty relation for pairs of complementary fuzzy sets.
Journal of Mathematical Analysis and Applications,
Vol. 121,
Issue. 1,
p.
273.
Schürmann, Michael
and
von Waldenfels, Wilhelm
1988.
Quantum Probability and Applications III.
Vol. 1303,
Issue. ,
p.
300.
Goderis, D.
and
Vets, P.
1989.
Central limit theorem for mixing quantum systems and the CCR-algebra of fluctuations.
Communications in Mathematical Physics,
Vol. 122,
Issue. 2,
p.
249.
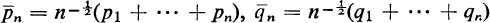 converges in distribution to a normal limit distribution.
converges in distribution to a normal limit distribution.