Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-01-14T14:33:45.962Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal stopping under g-Expectation with ${L}\exp\bigl(\mu\sqrt{2\log\!(1+\textbf{L})}\bigr)$-integrable reward process

Part of: Stochastic processes Stochastic analysis

Published online by Cambridge University Press:  14 September 2022

Mun-Chol Kim ,
Hun O  and
Ho-Jin Hwang
Mun-Chol Kim*
Affiliation:
Kim Il Sung University
Hun O*
Affiliation:
Kim Il Sung University
Ho-Jin Hwang*
Affiliation:
Kim Il Sung University
*
*Postal address: Faculty of Mathematics, Kim Il Sung University, Ryongnam-dong, Taesong District, Pyongyang, Democratic People’s Republic of Korea.
**Email address: [email protected]
*Postal address: Faculty of Mathematics, Kim Il Sung University, Ryongnam-dong, Taesong District, Pyongyang, Democratic People’s Republic of Korea.
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we study a class of optimal stopping problems under g-expectation, that is, the cost function is described by the solution of backward stochastic differential equations (BSDEs). Primarily, we assume that the reward process is 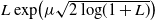$L\exp\bigl(\mu\sqrt{2\log\!(1+L)}\bigr)$ -integrable with 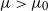$\mu>\mu_0$ for some critical value 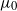$\mu_0$ . This integrability is weaker than $L^p$ -integrability for any 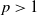$p>1$ , so it covers a comparatively wide class of optimal stopping problems. 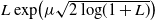To reach our goal, we introduce a class of reflected backward stochastic differential equations (RBSDEs) with $L\exp\bigl(\mu\sqrt{2\log\!(1+L)}\bigr)$ -integrable parameters. We prove the existence, uniqueness, and comparison theorem for these RBSDEs under Lipschitz-type assumptions on the coefficients. This allows us to characterize the value function of our optimal stopping problem as the unique solution of such RBSDEs.

Keywords

Optimal stoppingg-expectationL exp(µ√2 log(1 + L))-integrabilityreflected backward stochastic differential equation

MSC classification

Primary: 60H10: Stochastic ordinary differential equations 60H30: Applications of stochastic analysis (to PDE, etc.)
Secondary: 60G40: Stopping times; optimal stopping problems; gambling theory
Type
Original Article
Information
Journal of Applied Probability , Volume 60 , Issue 1 , March 2023 , pp. 241 - 252
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bayraktar, E. and Yao, S. (2011). Optimal stopping for non-linear expectations, II. Stoch. Process. Appl. 121, 212–264.CrossRefGoogle Scholar
Bayraktar, E. and Yao, S. (2012). Quadratic reflected BSDEs with unbounded obstacles. Stoch. Process. Appl. 122, 1155–1203.CrossRefGoogle Scholar
Bayraktar, E., Karatzas, I. and Yao, S. (2010). Optimal stopping for dynamic convex risk measures. Illinois J. Math. 54, 1025–1067.CrossRefGoogle Scholar
Buckdahn, R., Hu, Y. and Tang, S. (2018). Uniqueness of solution to scalar BSDEs with $L\exp\bigl(\mu\sqrt{2\log\!(1+L)}\bigr)$ -integrable terminal values. Electron. Commun. Prob. 23, 59.Google Scholar
Chen, Z. and Epstein, L. (2002). Ambiguity, risk and asset returns in continuous time. Econometrica 70, 1403–1443.CrossRefGoogle Scholar
Delbaen, F., Peng, S. and Rosazza Gianin, E. (2010). Representation of the penalty term of dynamic concave utilities. Finance Stoch. 14, 449–472.CrossRefGoogle Scholar
Duffie, D. and Epstein, L. (1992). Stochastic differential utility. Econometrica 60, 353–394.CrossRefGoogle Scholar
El Karoui, N. (1981). Les aspects probabilistes du contrôle stochastique. In Ninth Saint Flour Probability Summer School 1979 (Lecture Notes Math. 876), pp. 73–238. Springer, Berlin.Google Scholar
El Karoui, N., Kapoudjian, C., Pardoux, E., Peng, S. and Quenez, M.C. (1997). Reflected solutions of backward SDE’s and related obstacle problems for PDE’s. Ann. Prob. 25, 702–737.CrossRefGoogle Scholar
El Karoui, N., Pardoux, E. and Quenez, M. C. (1997). Reflected backward SDEs and American options. In Numerical Methods in Finance, eds L. C. G. Rogers and D. Talay, pp. 215–231. Cambridge University Press.CrossRefGoogle Scholar
Fan, S. and Hu, Y. (2019). Existence and uniqueness of solution to scalar BSDEs with $L\exp\bigl(\mu\sqrt{2\log\!(1+L)}\bigr)$ -integrable terminal values: the critical case. Electron. Commun. Prob. 24, 49.CrossRefGoogle Scholar
Hamadène, S. and Popier, A. (2012). $L^p$ -solutions for reflected backward stochastic differential equations. Stoch. Dyn. 12, 1150016.CrossRefGoogle Scholar
Hu, Y. and Tang, S. (2018). Existence of solution to scalar BSDEs with $L\exp\bigl(\sqrt{\frac{2}{\lambda}\log(1+L)}\bigr)$ -integrable terminal values. Electron. Commun. Prob. 23, 27.Google Scholar
Klenke, A. (2008). Probability Theory: A Comprehensive Course (Universitext). Springer, London.CrossRefGoogle Scholar
Klimsiak, T. (2012). Reflected BSDEs with monotone generator. Electron. J. Prob. 17, 107.CrossRefGoogle Scholar
Kobylanski, M., Lepeltier, J. P., Quenez, M. C. and Torres, S. (2002) Reflected BSDEs with superlinear quadratic coefficient. Prob. Math. Statist. 22, 51–83.Google Scholar
Lepeltier, J. P. and Xu, M. (2007). Reflected BSDE with quadratic growth and unbounded terminal value. Available at arXiv:0711.0619.Google Scholar
Lepeltier, J. P., Matoussi, A. and Xu, M. (2005). Reflected backward stochastic differential equations under monotonicity and general increasing growth conditions. Adv. Appl. Prob. 37, 134–159.CrossRefGoogle Scholar
Peng, S. (1997). Backward SDE and related g-expectation. In Backward Stochastic Differential Equations (Pitman Res. Notes Math. Ser. 364), eds N. El Karoui and L. Mazliak, pp. 141–159. Longman, Harlow.Google Scholar
Quenez, M.C. and Sulem, A. (2014). Reflected BSDEs and robust optimal stopping for dynamic risk measures with jumps. Stoch. Process. Appl. 124, 3031–3054.CrossRefGoogle Scholar
Rosazza Gianin, E. (2006). Risk measures via g-expectations. Insurance Math. Econom. 39, 19–34.CrossRefGoogle Scholar
Rozkosz, A. and Slomiński, L. (2012). $L^p$ -solutions of reflected BSDEs under monotonicity condition. Stoch. Process. Appl. 122, 3875–3900.CrossRefGoogle Scholar
Wu, H. (2013). Optimal stopping under g-expectation with constraints. Operat. Res. Lett. 41, 164–171.CrossRefGoogle Scholar