Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-18T18:20:45.933Z Has data issue: false hasContentIssue false
  • English
  • Français

Functional normalizations for the branching process with infinite mean

Published online by Cambridge University Press:  14 July 2016

Andrew D. Barbour  and
H.-J. Schuh
Andrew D. Barbour*
Affiliation:
Gonville and Caius College, Cambridge
H.-J. Schuh
Affiliation:
CSIRO Division of Mathematics and Statistics, Canberra
*
∗ Postal address: Statistical Laboratory, 16 Mill Lane, Cambridge CB2 1SB, U.K.
Get access Rights & Permissions [Opens in a new window]

Abstract

It is well known that, in a Bienaymé-Galton–Watson process (Zn) with 1 < m = EZ1 < ∞ and EZ1 log Z1 <∞, the sequence of random variables Znm –n converges a.s. to a non–degenerate limit. When m =∞, an analogous result holds: for any 0< α < 1, it is possible to find functions U such that α n U (Zn) converges a.s. to a non-degenerate limit. In this paper, some sufficient conditions, expressed in terms of the probability generating function of Z1 and of its distribution function, are given under which a particular pair (α, U) is appropriate for (Zn). The most stringent set of conditions reduces, when U (x) 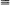x, to the requirements EZ1 = 1/α, EZ1 log Z1 <∞.

Keywords

BRANCHING PROCESSFUNCTIONAL NORMALIZATION
Type
Research Papers
Information
Journal of Applied Probability , Volume 16 , Issue 3 , September 1979 , pp. 513 - 525
Copyright
Copyright © Applied Probability Trust 1979 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

∗∗

Present address: Department of Statistics, Richard Berry Building, University of Melbourne Parkville, Victoria 3052, Australia.

References

Davies, P. L. (1978) The simple branching process: a note on convergence when the mean is infinite. J. Appl. Prob. 15, 466–480.Google Scholar
Hudson, I. L. and Seneta, E. (1977) A note on simple branching processes with infinite mean. J. Appl. Prob. 14, 836–842.Google Scholar
Kesten, H. and Stigum, B. P. (1966) A limit theorem for multi-dimensional Galton–Watson processes. Ann. Math. Statist. 37, 1211–1223.Google Scholar
Schuh, H-J. and Barbour, A. D. (1977) On the asymptotic behaviour of branching processes with infinite mean. Adv. Appl. Prob. 9, 681–723.Google Scholar
Seneta, E. (1976) Regularly Varying Functions. Springer-Verlag, Berlin.Google Scholar