Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pakes, A. G.
1971.
Some limit theorems for the total progeny of a branching process.
Advances in Applied Probability,
Vol. 3,
Issue. 1,
p.
176.
Pakes, A. G.
1971.
A branching process with a state dependent immigration component.
Advances in Applied Probability,
Vol. 3,
Issue. 2,
p.
301.
Pakes, A. G.
1972.
Further results on the critical Galton-Watson process with immigration.
Journal of the Australian Mathematical Society,
Vol. 13,
Issue. 3,
p.
277.
Heyde, C. C.
and
Seneta, E.
1972.
Estimation theory for growth and immigration rates in a multiplicative process.
Journal of Applied Probability,
Vol. 9,
Issue. 2,
p.
235.
Hering, H.
1973.
Asymptotic behaviour of immigration-branching processes with general set of types. I: Critical branching part.
Advances in Applied Probability,
Vol. 5,
Issue. 3,
p.
391.
Iosifescu, M.
and
Tăutu, P.
1973.
Stochastic processes and applications in biology and medicine I.
Vol. 3,
Issue. ,
p.
11.
Hering, H.
1973.
Asymptotic behaviour of immigration-branching processes with general set of types. I: Critical branching part.
Advances in Applied Probability,
Vol. 5,
Issue. 3,
p.
391.
Pakes, A. G.
1973.
On dams with Markovian inputs.
Journal of Applied Probability,
Vol. 10,
Issue. 2,
p.
317.
Pakes, A. G.
1974.
On supercritical Galton-Watson processes allowing immigration.
Journal of Applied Probability,
Vol. 11,
Issue. 04,
p.
814.
Pakes, A. G.
1974.
On supercritical Galton-Watson processes allowing immigration.
Journal of Applied Probability,
Vol. 11,
Issue. 4,
p.
814.
Kaplan, Norman
1974.
Limit theorems for the integral of a population process with immigration.
Stochastic Processes and their Applications,
Vol. 2,
Issue. 3,
p.
281.
Pakes, A.G.
1975.
Limit theorems for the integrals of some branching processes.
Stochastic Processes and their Applications,
Vol. 3,
Issue. 1,
p.
89.
Pakes, A.G.
1975.
Some results for non-supercritical Galton-Watson processes with immigration.
Mathematical Biosciences,
Vol. 24,
Issue. 1-2,
p.
71.
Pakes, A.G.
1975.
Non-parametric estimation in the Galton-Watson process.
Mathematical Biosciences,
Vol. 26,
Issue. 1-2,
p.
1.
Pakes, A.G.
and
Parthasarathy, P.R.
1975.
Some convergence rate results for the Bellman-Harris process with immigration.
Mathematical Biosciences,
Vol. 26,
Issue. 3-4,
p.
207.
Stewart, David B.
1976.
A subcritical branching process with state dependent immigration.
Mathematical Biosciences,
Vol. 31,
Issue. 1-2,
p.
175.
Stewart, David B.
1976.
A supercritical branching process with state dependent immigration.
Mathematical Biosciences,
Vol. 32,
Issue. 3-4,
p.
187.
Pakes, Anthony G.
1979.
Limit theorems for the simple branching process allowing immigration, I. The case of finite offspring mean.
Advances in Applied Probability,
Vol. 11,
Issue. 1,
p.
31.
Pakes, Anthony G.
1979.
Limit theorems for the simple branching process allowing immigration, I. The case of finite offspring mean.
Advances in Applied Probability,
Vol. 11,
Issue. 1,
p.
31.
Bhat, B. R.
and
Adke, S. R.
1981.
Maximum likelihood estimation for branching processes with immigration.
Advances in Applied Probability,
Vol. 13,
Issue. 3,
p.
498.
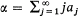 , satisfies α < 1 or α > 1, respectively. Unless stated specifically to the contrary, we assume that the following condition holds.
, satisfies α < 1 or α > 1, respectively. Unless stated specifically to the contrary, we assume that the following condition holds.

