Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-04T18:55:53.828Z Has data issue: false hasContentIssue false
  • English
  • Français

Average cost under the PMλ, τ policy in a finite dam with compound Poisson inputs

Part of: Operations research and management science Special processes

Published online by Cambridge University Press:  14 July 2016

Jongho Bae ,
Sunggon Kim  and
Eui Yong Lee
Jongho Bae*
Affiliation:
Jeonju University
Sunggon Kim*
Affiliation:
KAIST
Eui Yong Lee*
Affiliation:
Sookmyung Women's University
*
∗ Postal address: Department of Mathematics, Jeonju University, Jeonju, 560-759, Republic of Korea. Email address: [email protected]
∗∗ Postal address: Department of Electrical Engineering and Computer Science, KAIST, Daejon, 305-701, Republic of Korea.
∗∗∗ Postal address: Department of Statistics, Sookmyung Women's University, Seoul, 140-742, Republic of Korea.
Get access Rights & Permissions [Opens in a new window]

Abstract

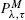We consider the policy in a finite dam in which the input of water is formed by a compound Poisson process and the rate of water release is changed instantaneously from a to M and from M to a (M > a) at the moments when the level of water exceeds λ and downcrosses τ (λ > τ) respectively. After assigning costs to the changes of release rate, a reward to each unit of output, and a cost related to the level of water in the reservoir, we determine the long-run average cost per unit time.

Keywords

PMλ, τ policyfinite damcompound Poisson inputlong-run average cost

MSC classification

Primary: 60K25: Queueing theory
Secondary: 90B05: Inventory, storage, reservoirs
Type
Short Communications
Information
Journal of Applied Probability , Volume 40 , Issue 2 , June 2003 , pp. 519 - 526
Copyright
Copyright © Applied Probability Trust 2003 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdel-Hameed, M. S. (2000). Optimal control of a dam using PM λ,τ policies and penalty cost when the input process is a compound Poisson process with positive drift. J. Appl. Prob. 37, 408–416.Google Scholar
Asmussen, S. (1987). Applied Probability and Queues. John Wiley, Chichester.Google Scholar
Bae, J., Kim, S., and Lee, E. Y. (2001). The virtual waiting time of the M/G/1 queue with impatient customers. Queueing Systems 38, 485–494.CrossRefGoogle Scholar
Bae, J., Kim, S., and Lee, E. Y. (2002). A PM λ-policy for an M/G/1 queueing system. Appl. Math. Modelling 26, 929–939.CrossRefGoogle Scholar
Faddy, M. J. (1974). Optimal control of finite dams: discrete (2-stage) output procedure. J. Appl. Prob. 11, 111–121.CrossRefGoogle Scholar
Lee, E. Y., and Ahn, S. K. (1998). Pλ M-policy for a dam with input formed by a compound Poisson process. J. Appl. Prob. 35, 482–488.Google Scholar
Ross, S. M. (1983). Stochastic Processes. John Wiley, New York.Google Scholar
Yeh, L. (1985). Optimal control of a finite dam: average-cost case. J. Appl. Prob. 22, 480–484.CrossRefGoogle Scholar