Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
A-Hameed, M.S.
and
Proschan, F.
1973.
Nonstationary shock models.
Stochastic Processes and their Applications,
Vol. 1,
Issue. 4,
p.
383.
Abdel-Hameed, Mohamed
1975.
A Gamma Wear Process.
IEEE Transactions on Reliability,
Vol. R-24,
Issue. 2,
p.
152.
Kirstein, B.M.
1976.
Monotonicity and comparability of time-homogeneous markov processes with discrete state space.
Mathematische Operationsforschung und Statistik,
Vol. 7,
Issue. 1,
p.
151.
Barlow, Richard E.
and
Proschan, Frank
1976.
Some Current Academic Research in System Reliability Theory.
IEEE Transactions on Reliability,
Vol. R-25,
Issue. 3,
p.
198.
Cambanis, Stamatis
Simons, Gordon
and
Stout, William
1976.
Inequalities for E k(X, Y) when the marginals are fixed.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 36,
Issue. 4,
p.
285.
Franken, Peter
and
Kirstein, Bernd Michael
1977.
Zur Vergleichbarkeit zufälliger Prozesse.
Mathematische Nachrichten,
Vol. 78,
Issue. 1,
p.
197.
Kirstein, B. M.
Franken, P.
and
Stoyan, D.
1977.
Comparability and Monotonicity of Markov Processes.
Theory of Probability & Its Applications,
Vol. 22,
Issue. 1,
p.
40.
Nevius, S.E.
Proschan, F.
and
Sethuraman, J.
1977.
Statistical Decision Theory and Related Topics.
p.
281.
1979.
Inequalities - Theory of Majorization and Its Applications.
Vol. 143,
Issue. ,
p.
531.
1980.
Probability Inequalities in Multivariate Distributions.
p.
208.
Kim, Jee Soo
Proschan, Frank
and
Sethuraman, Jayaram
1988.
Stochastic comparisons of order statistics,with applications in relibility.
Communications in Statistics - Theory and Methods,
Vol. 17,
Issue. 7,
p.
2151.
Ebrahimi, Nader
1990.
Ordering of repairable systems.
Journal of Applied Probability,
Vol. 27,
Issue. 01,
p.
193.
Boland, Philip J.
Proschan, Frank
and
Tong, Y. L.
1992.
A stochastic ordering of partial sums of independent random variables and of some random processes.
Journal of Applied Probability,
Vol. 29,
Issue. 03,
p.
645.
Rangan, A.
and
Sarada, G.
1993.
Some results on the life distribution properties of systems subject to shocks and general repair.
Microelectronics Reliability,
Vol. 33,
Issue. 1,
p.
1.
Cheng, Cheng
and
Tong, Y.L.
1999.
Concentration order on a metric space, with some statistical applications.
Statistics & Probability Letters,
Vol. 44,
Issue. 4,
p.
327.
Lehmann, E. L.
and
Rojo, J.
2012.
Selected Works of E. L. Lehmann.
p.
793.
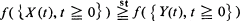 for increasing functionals f.
for increasing functionals f.