Article contents
Stationary M/G/1 excursions in the presence of heavy tails
Published online by Cambridge University Press: 14 July 2016
Abstract
It is shown that the stationary excursions above level x for the stationary M/G/1 queue with the service time distribution belonging to a certain class 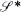 of subexponential distributions are asymptotically of two types as x →∞: either the excursion starts with a jump from a level which is O(1) and the initial excess over x converges to ∞, or it starts from a level of the form x – O(1) and the excess has a proper limit distribution. The two types occur with probabilities ρ, resp. 1 – ρ.
of subexponential distributions are asymptotically of two types as x →∞: either the excursion starts with a jump from a level which is O(1) and the initial excess over x converges to ∞, or it starts from a level of the form x – O(1) and the excess has a proper limit distribution. The two types occur with probabilities ρ, resp. 1 – ρ.
Keywords
MSC classification
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1997
References
- 5
- Cited by


