No CrossRef data available.
Article contents
The number of K-tons in the coupon collector problem
Published online by Cambridge University Press: 07 February 2023
Abstract
Consider the coupon collector problem where each box of a brand of cereal contains a coupon and there are n different types of coupons. Suppose that the probability of a box containing a coupon of a specific type is 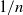 $1/n$, and that we keep buying boxes until we collect at least m coupons of each type. For
$1/n$, and that we keep buying boxes until we collect at least m coupons of each type. For 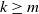 $k\geq m$ call a certain coupon a k-ton if we see it k times by the time we have seen m copies of all of the coupons. Here we determine the asymptotic distribution of the number of k-tons after we have collected m copies of each coupon for any k in a restricted range, given any fixed m. We also determine the asymptotic joint probability distribution over such values of k, and the total number of coupons collected.
$k\geq m$ call a certain coupon a k-ton if we see it k times by the time we have seen m copies of all of the coupons. Here we determine the asymptotic distribution of the number of k-tons after we have collected m copies of each coupon for any k in a restricted range, given any fixed m. We also determine the asymptotic joint probability distribution over such values of k, and the total number of coupons collected.
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of Applied Probability Trust



