Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T02:14:33.996Z Has data issue: false hasContentIssue false
  • English
  • Français

A Markov construction for a multidimensional point process

Published online by Cambridge University Press:  14 July 2016

Valerie Isham
Valerie Isham*
Affiliation:
Imperial College, London
Get access Rights & Permissions [Opens in a new window]

Abstract

A sequence of finite point processes {Pn} is constructed in 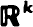using a Markov sequence of points. Essentially, in the process Pn consisting of n events, the coordinates of these events are simply the first n points of a Markov sequence suitably scaled so that the average density of the process is independent of n. The second-order properties of Pn are discussed and sufficient conditions are found for Pn to converge in distribution to a Poisson process as n →∞. A simple example involving the cardioid distribution is described.

Keywords

MULTIDIMENSIONAL POINT PROCESSFINITE POINT PROCESSMARKOV SEQUENCECARDIOID DISTRIBUTION
Type
Research Papers
Information
Journal of Applied Probability , Volume 14 , Issue 3 , September 1977 , pp. 507 - 515
Copyright
Copyright © Applied Probability Trust 1977 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Daley, D. J. and Vere-Jones, D. (1972) A summary of the theory of point processes. In Stochastic Point Processes , ed. Lewis, P.A.W., Wiley, New York, 299–393.Google Scholar
Doob, J. L. (1953) Stochastic Processes. Wiley, New York.Google Scholar
Fisher, L. (1972) A survey of the mathematical theory of multidimensional point processes. In Stochastic Point Processes , ed. Lewis, P.A.W., Wiley, New York, 468–513.Google Scholar
Isham, V. (1974) Some Multidimensional Point Processes. Unpublished Ph.D. thesis, University of London.Google Scholar
Isham, V. (1975) On a point process with independent locations. J. Appl. Prob. 12, 435–446.Google Scholar
Isham, V. (1976) Constructions for planar point processes using concentric circles. Stoch. Proc. Appl. To appear.Google Scholar