Article contents
Critical behavior of percolation and Markov fields on branching planes
Published online by Cambridge University Press: 14 July 2016
Abstract
For an independent percolation model on 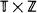 , where
, where 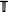 is a homogeneous tree and
is a homogeneous tree and 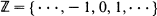 is a one-dimensional lattice, it is shown, by verifying that the triangle condition is satisfied, that the percolation probability θ (p) is a continuous function of p at the critical point pc, and the critical exponents
is a one-dimensional lattice, it is shown, by verifying that the triangle condition is satisfied, that the percolation probability θ (p) is a continuous function of p at the critical point pc, and the critical exponents 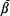 , γ, δ, and Δ exist and take their mean-field values. Some analogous results for Markov fields on
, γ, δ, and Δ exist and take their mean-field values. Some analogous results for Markov fields on 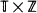 are also obtained.
are also obtained.
MSC classification
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1993
Footnotes
Research supported in part by NSF Grants DMS-8902156 and DMS-9196086.
References
- 9
- Cited by


