Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-12T16:30:06.275Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Existence of an Universal Spin-down Equation for Pulsars

Published online by Cambridge University Press:  12 April 2016

César Alvarez  and
Alberto Carramiñana
César Alvarez
Affiliation:
Instituto National de Astrofísica Optica y Electrónica (INAOE), Luis Enrique Erro No. 1 Tonantzintla Puebla, México. C.P. 72840
Alberto Carramiñana
Affiliation:
Instituto National de Astrofísica Optica y Electrónica (INAOE), Luis Enrique Erro No. 1 Tonantzintla Puebla, México. C.P. 72840

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Taylor expansion of the generalized spin-down equation 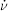= − f(v), derives into the multipolar equation 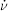= −gv5 – rv3 − sv), which is used here to study pulsar evolution. The coefficients g, r and s can be physically associated to gravitational radiation, magnetic dipolar radiation and particle aceleration. The multipolar expansion with constant coefficients cannot describe the P Ṗ diagram in a consistent manner and therefore it is unlikely a generalized equation of the form 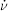= −f(v;t) can be used to describe pulsar evolution. A more general form, 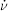= − f(v; t) can be investigated giving to the coefficients g, r and s time dependence. We consider some explicit functional forms for g(t), r(t) and s(t) which are consistent with observational and theoretical constraints.

Type
Part 9. Population and Neutron Star Properties
Information
International Astronomical Union Colloquium , Volume 177: Pulsar Astronomy - 2000 and Beyond , 2000 , pp. 601 - 602
Copyright
Copyright © Astronomical Society of the Pacific 2000

References

Alvarez, C., Carramiñana, A. 1998, RevMexAASC, 7, 207 Google Scholar
Bhattacharya, D., Wijers, R.A.M.J., Hartman, J.W., Verbunt, F. 1992, A&A 254, 198 Google Scholar
Boyd, P.T. et al. 1995, ApJ, 448, 365 CrossRefGoogle Scholar
Kaspi, V.M., et al. 1994, ApJ 422, L83 Google Scholar
Lyne, A.G., Pritchard, R.S., & Smith, F.G. 1993, MNRAS, 265, 1003 Google Scholar
Lyne, A.G., Pritchard, R.S., Smith, F.G., & Camilo, F. 1996 Nature, 381, 497 Google Scholar
New, K.C.B., Chanmugam, G., Johnson, W.W., Tohline, J.E. 1995, ApJ 450, 757 Google Scholar
Thompson, D.J., et. al. 1994, ApJ, 436, 229 Google Scholar