Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-12T10:38:38.440Z Has data issue: false hasContentIssue false
  • English
  • Français

Determination of Resonance terms using Optical Observations of Two Meteosat Satellites

Published online by Cambridge University Press:  12 April 2016

U. Hugentobler ,
T. Schildknecht  and
G. Beutler
U. Hugentobler
Affiliation:
Astronomical Institute, University of Berne Berne, Switzerland
T. Schildknecht
Affiliation:
Astronomical Institute, University of Berne Berne, Switzerland
G. Beutler
Affiliation:
Astronomical Institute, University of Berne Berne, Switzerland

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

During an observation campaign in winter 94/95 astrometric positions from Meteosat 4 and 5 were acquired at the Zimmerwald observatory using a CCD camera mounted in the prime focus of the 0.5 m Satellite Laser Ranging telescope. The measurements cover a time interval of four months, their precision is of the order of .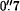

The modeling of radiation pressure for the small, cylindrically shaped satellites is relatively easy and they are therefore excellent objects to probe the geopotential. The orbital parameters and the radiation pressure coefficients for the two satellites as well as the resonant coefficients C22, S22 of the geopotential were determined by a single least square adjustment procedure including all the Zimmerwald observations. The relative errors estimated for the terms C22 and S22 are of the order of 1 ÷ 3 · 10−4.

Type
Dynamics of Artificial Satellites and Space Debris
Information
International Astronomical Union Colloquium , Volume 165: Dynamics and Astrometry of Natural and Artificial Celestial Bodies , 1997 , pp. 355 - 360
Copyright
Copyright © Kluwer 1997

References

Beutler, G., Brockmann, E., Hugentobler, U., Mervart, M., Rothacher, M., and Weber, R.: 1996, “Combining consecutive short arcs into long arcs for precise and efficient GPS orbit determination”, J. Geodyn. 70, 287–299.Google Scholar
Blitzer, L., Boughton, E.M., Kang, G., and Page, R.M.: 1962, “Effect of ellipticity of the equator on 24-hour nearly circular satellite orbits”, J. Geophys. Res. 67, 329–335.Google Scholar
Catalano, S., McCrosky, R., Milani, A., and Nobili, A.M.: 1983, “Optical tracking of synchronous Earth’s satellites for geophysical purposes”, J. Geophys. Res. 88, 669–676.Google Scholar
Kovalevsky, J.: 1961, First Int. Symposium on Analytical Astrodynamics, UCLA.Google Scholar
Schildknecht, T., Hugentobler, U., and Verdun, A.: 1993, “CCD astrometry of artificial satellites”, in: Dynamics and Astrometry of Natural and Artificial Celestial Bodies (Kurzyńska, K., Barlier, F., Seidelmann, P.K., Wytrzyszczak, I., eds), Astronomical Observatory, Poznan, 103–108.Google Scholar
Schildknecht, T.: 1994, “Optical astrometry of fast moving objects using CCD detectors”, Ph.D. thesis, Geodätisch-geophysikalische Arbeiten in der Schweiz, Vol. 49.Google Scholar
Sehnal, L.: 1960, “The perturbations of the orbit of the stationary satellite of the Earth”, Bull. Astron. Inst. Czech. 11, 132–135.Google Scholar
Veillet, Ch., Metris, G., and Boudon, Y.: 1990, “Status report of Cerga/OCA”, Third COGEOS Workshop, Brussels, June 11-12, 1990.Google Scholar
Wagner, C.A.: 1965, “A determination of Earth equatorial ellipticity from seven months of Syncom 2 longitude drift”, J. Geophys. Res. 70, 1566–1568.Google Scholar