Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-03T11:31:32.331Z Has data issue: false hasContentIssue false
  • English
  • Français

Star-complexes, and the dependence problems for hyperbolic complexes

Published online by Cambridge University Press:  18 May 2009

Stephen J. Pride
Stephen J. Pride
Affiliation:
Department of Mathematics, University of Glasgow, University Gardens, Glasgow G12 8QW
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

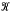Given a group presentation (or more generally† a 2-complex) one can associate with it an object which has variously been called the co-initial graph, star-graph, star-complex, and which has proved useful in several contexts [2], [6], [7], [8], [9], [10], [12]. For certain mappings of 2-complexes φ: ⃗ℒ (”strong mappings”) one gets an induced mapping φst: 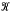st⃗ℒst of the associated star-complexes. Then st is a covariant functor from the category of 2-complexes (where the morphisms are strong mappings) to the category of 1-complexes, and this functor behaves very nicely with respect to coverings (Theorem 1).

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 30 , Issue 2 , May 1988 , pp. 155 - 170
Copyright
Copyright © Glasgow Mathematical Journal Trust 1988

References

1.Comerford, L. P. Jr, Subgroups of small cancellation groups, J. London Math. Soc. (2) 17 (1978), 422–424.CrossRefGoogle Scholar
2.El-Mosalamy, M., Free subgroups of small cancellation groups, Israel J. Math., 56 (1986), 345–348.CrossRefGoogle Scholar
3.El-Mosalamy, M. and Pride, S. J., On T(6)-groups, Math. Proc. Cambridge Philos. Soc., 102 (1987), 443–451.CrossRefGoogle Scholar
4.Gersten, S. M., Reducible diagrams and equations over groups in Essays in group theory, edited by Gersten, S. M. (Springer-Verlag, 1987).Google Scholar
5.Gersten, S. M., Branched coverings of 2-complexes and diagrammatic asphericity, preprint.Google Scholar
6.Hill, P., Pride, S. J. and Vella, A. D., On the T(q)-conditions of small cancellation theory, Israeli. Math. 52 (1985), 293–304.CrossRefGoogle Scholar
7.Hoare, A. H. M., Coinitial graphs and Whitehead automorphisms. Canad. J. Math. 31 (1979), 112–123.CrossRefGoogle Scholar
8.Hoare, A. H. M., Karrass, A. and Solitar, D., Subgroups of finite index in Fuchsian groups, Math. Z. 120 (1971), 289–298.Google Scholar
9.Hoare, A. H. M., Karrass, A. and Solitar, D., Subgroups of infinite index in Fuchsian groups, Math. Z. 125 (1972), 59–69.CrossRefGoogle Scholar
10.Hoare, A. H. M., Karrass, A. and Solitar, D., Subgroups of NEC groups, Comm. Pure Appl. Math. 26 (1973), 731–744.CrossRefGoogle Scholar
11.Lyndon, R. C. and Schupp, P. E., Combinatorial group theory (Springer-Verlag, 1977).Google Scholar
12.Pride, S. J., On Tits' conjecture and other questions concerning Artin and generalized Artin groups, Invent. Math., 86 (1986), 347–356.Google Scholar
13.Pride, S. J., Involutary presentations, with applications to Coxeter groups, NEC-groups and groups of Kanevskiῐ, J. Algebra, to appear.Google Scholar
14.Gromov, M., Hyperbolic groups in Essays in group theory, edited by Gersten, S. M. (Springer-Verlag, 1987).Google Scholar