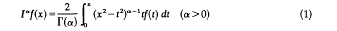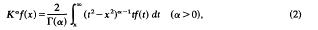Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lowndes, J. S.
1983.
Cauchy problems for second order hyperbolic differential equations with constant coefficients.
Proceedings of the Edinburgh Mathematical Society,
Vol. 26,
Issue. 3,
p.
307.
Debnath, Lokenath
2004.
A brief historical introduction to fractional calculus.
International Journal of Mathematical Education in Science and Technology,
Vol. 35,
Issue. 4,
p.
487.
Rubin, Boris
2008.
Inversion formulae for the spherical mean in odd dimensions and the Euler–Poisson–Darboux equation.
Inverse Problems,
Vol. 24,
Issue. 2,
p.
025021.
Antipov, Yuri A.
Estrada, Ricardo
and
Rubin, Boris
2012.
Method of analytic continuation for the inverse spherical mean transform in constant curvature spaces.
Journal d'Analyse Mathématique,
Vol. 118,
Issue. 2,
p.
623.
Kiryakova, Virginia
2014.
From the hyper-Bessel operators of Dimovski to the generalized fractional calculus.
Fractional Calculus and Applied Analysis,
Vol. 17,
Issue. 4,
p.
977.
2020.
Transmutations, Singular and Fractional Differential Equations with Applications to Mathematical Physics.
p.
527.
Shishkina, Elina
and
Sitnik, Sergei
2020.
Transmutations, Singular and Fractional Differential Equations with Applications to Mathematical Physics.
p.
85.
2020.
Transmutations, Singular and Fractional Differential Equations with Applications to Mathematical Physics.
p.
xv.