Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T01:29:33.690Z Has data issue: false hasContentIssue false
  • English
  • Français

On a Ramanujan's identity

Published online by Cambridge University Press:  18 May 2009

Manvendra Tamba
Manvendra Tamba
Affiliation:
Ramanujan Institute for Advanced Study in Mathematics, University of Madras, Madras-600 005, India
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In [4], Ramanujan stated the following beautiful identity which was later proved by Bailey [2] and [3];

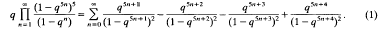
We denote by S(n) the coefficient of qn on the right hand side of (1). The object of this paper is to prove the following two theorems.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 34 , Issue 1 , January 1992 , pp. 17 - 19
Copyright
Copyright © Glasgow Mathematical Journal Trust 1992

References

REFERENCES

1.Andrews, G. E., The theory of partitions, Encyclopedia of Math. Appl., Vol. 2 (Addison-Wesley, 1976).Google Scholar
2.Bailey, W. N., A note on two of Ramanujan's formulae, Quart. J. Math. 3 (1952), 29–31.CrossRefGoogle Scholar
3.Bailey, W. N., A further note on two of Ramanujan's formulae, Quart. J. Math. 3 (1952), 158–160.CrossRefGoogle Scholar
4.Ramanujan, S., Congruence properties of p(n), (unpublished manuscript), Trinity College Library (1920).Google Scholar