Hostname: page-component-745bb68f8f-f46jp Total loading time: 0 Render date: 2025-01-26T07:04:35.733Z Has data issue: false hasContentIssue false
  • English
  • Français

$L^p$ harmonic 1-forms on hypersurfaces with finite index

Part of: Global differential geometry

Published online by Cambridge University Press:  02 November 2022

Xiaoli Chao ,
Bin Shen  and
Miaomiao Zhang
Xiaoli Chao*
Affiliation:
School of Mathematics, Southeast University, Nanjing 211189, P. R. China
Bin Shen
Affiliation:
School of Mathematics, Southeast University, Nanjing 211189, P. R. China
Miaomiao Zhang
Affiliation:
School of Mathematics, Southeast University, Nanjing 211189, P. R. China Current Address: School of Mathematical Sciences, Laboratory of Mathematics and Complex Systems, Beijing Normal University, Beijing 100875, P.R. China
*
*Corresponding author. E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the present note, we establish a finiteness theorem for 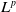$L^p$ harmonic 1-forms on hypersurfaces with finite index, which is an extension of the result of Choi and Seo (J. Geom. Phys. 129 (2018), 125–132).

Keywords

Lp harmonic 1-formindexend

MSC classification

Primary: 53C40: Global submanifolds 53C42: Immersions (minimal, prescribed curvature, tight, etc.)
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 65 , Issue 2 , May 2023 , pp. 310 - 323
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Glasgow Mathematical Journal Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Calderbank, D. M., Gauduchon, P. and Herzlich, M., Refined Kato inequalities and conformal weights in Riemannian geometry, J. Funct. Anal. 173 (2000), 214–255.CrossRefGoogle Scholar
Carron, G., $L^2$ -Cohomologie et inégalités de Sobolev, Math. Ann. 314 (1999), 613–639.CrossRefGoogle Scholar
Cavalcante, M. P., Mirandola, H. and Vitório, F., $L^2$ harmonic 1-form on submanifolds with finite total curvature, J. Geom. Anal. 24 (2014), 205–222.Google Scholar
Chao, X. L., Hui, A. Y. and Bai, M. M., Vanishing theorems for p-harmonic $\ell$ -forms on Riemannian manifolds with a weighted Poincare inequality, Diff. Geom. Appl. 76 (2021), 101741.CrossRefGoogle Scholar
Chao, X. L. and Lv, Y. S., $L^2$ harmonic 1-form on submanifold with weighted Póincare inequality, J. Korean Math. Soc. 53 (2016), 583–595.CrossRefGoogle Scholar
Chao, X. L. and Lv, Y. S., $L^p$ harmonic 1-form on submanifold with weighted Póincare inequality, Czech. Math. J. 68 (2018), 195–217.Google Scholar
Choi, H. and Seo, K., $L^p$ harmonic 1-form on minimal hypersurfaces with finite index, J. Geom. Phys. 129 (2018), 125–132.Google Scholar
Dung, N. T. and Seo, K., Stable minimal hypersurfaces in a Riemannian manifold with pinched negative sectional curvature, Ann. Global Anal. Geom. 41 (2012), 447–460.CrossRefGoogle Scholar
Dung, N. T. and Seo, K., Vanishing theorems for $L^2$ harmonic 1-forms on complete submanifolds in a Riemannian manifold, J. Math. Anal. Appl. 423 (2015), 1594–1609.CrossRefGoogle Scholar
Fischer-Colbrie, D., On complete minimal surfaces with finite Morse index in three-manifolds, Invent. Math. 82 (1985), 121–132.CrossRefGoogle Scholar
Fu, H. P. and Li, Z. Q., $L^2$ harmonic 1-forms on complete submanifolds in Euclidean space, Kodai Math. J. 32 (2009), 432–441.CrossRefGoogle Scholar
Greene, R. E. and Wu, H., Integrals of subharmonic functions on manifolds of nonnegative curvature, Invent. Math. 27 (1974), 265–298.10.1007/BF01425500CrossRefGoogle Scholar
Greene, R. E. and Wu, H., Harmonic forms on noncompact Riemannian and Kähler manifolds, Michigan Math. J. 28 (1981), 63–81.CrossRefGoogle Scholar
Hoffman, D. and Spruck, J., Sobolev and isoperimetric inequalities for Riemannian submanifolds, Commun. Pure Appl. Math. 27 (1974), 715–727.10.1002/cpa.3160270601CrossRefGoogle Scholar
Kawai, S., Operator $\triangle-aK$ on surfaces, Hokkaido Math. J. 17 (1988), 147–150.Google Scholar
Lam, K. H., Results on a weighted Poincaré inequality of complete manifolds, Trans. Am. Math. Soc. 362 (2010), 5043–5062.CrossRefGoogle Scholar
Li, P., On the Sobolev constant and the p-spectrum of a compact Riemannian manifold, Ann. Sci. école Norm. Sup. 13 (1980), 451–468.10.24033/asens.1392CrossRefGoogle Scholar
Li, P., Geometric Analysis, Cambridge Studies in Advanced Mathematics, vol. 134 (Cambridge University Press, Cambridge, 2012), x+406 pp.Google Scholar
Li, P. and Schoen, R., $L^p$ and mean value properties of subharmonic functions on Riemannian manifolds, Acta Math. 153 (1984), 279–301.CrossRefGoogle Scholar
Li, P. and Wang, J., Complete manifolds with positive spectrum, J. Differential. Geom. 58 (2001), 501–534.CrossRefGoogle Scholar
Matheus, V., Vanishing theorems for $L^2$ harmonic forms on complete Riemannian manifolds, arXiv:1407.0236v1.Google Scholar
Miyaoka, R., $L^2$ harmonic 1-forms on a complete stable minimal hypersurface, in Geometry Global Analysis. (Tohoku University, Sendai, 1993), 289–293.Google Scholar
Palmer, B., Stability of minimal hypersurfaces, Comment. Math. Helv. 66 (1991), 185–188.CrossRefGoogle Scholar
Pigola, S., Rigoli, M. and Setti, A. G., Vanishing and finiteness results in geometric analysis. A generalization of the Bochner technique, in Progress in Mathematics, vol. 266 (Birkh $\ddot{a}$ user Verlag, Basel, 2008).Google Scholar
Sang, N. N. and Thanh, N. T., Stability minimal hypersurfaces with weighted Poincaré inequality in a Riemannian manifold, Commum. Korean. Math. Soc. 29 (2014), 123–130.Google Scholar
Seo, K., $L^p$ harmonic 1-forms and first eigenvalue of a stable minimal hypersurface, Paci. J. Math. 268 (2014), 205–229.Google Scholar
Seo, K., Rigidity of minimal submanifolds in hyperbolic space, Arch. Math. 94 (2010), 173–181.CrossRefGoogle Scholar
Seo, K., $L^2$ harmonic 1-forms on minimal submanifolds in hyperbolic space, J. Math. Anal. Appl. 371 (2010), 546–551.CrossRefGoogle Scholar
Shiohama, K. and Xu, H., The topological sphere theorem for complete submanifolds, Compos. Math. 107 (1997), 221–232.CrossRefGoogle Scholar
Tam, L. F. and Zhou, D., Stability properties for the higher dimensional catenoid in $\mathbb{R}^{n+1}$ , Proc. Am. Math. Soc. 137 (2009), 3451–3461.CrossRefGoogle Scholar
Tysk, J., Finiteness of index and total scalar curvature for minimal hypersurfaces, Proc. Am. Math. Soc. 105(2) (1989), 429–435.CrossRefGoogle Scholar
Wang, P., Chao, X., Wu, Y. and Lv, Y., Harmonic p-forms on Hadamard manifolds with finite total curvature, Ann. Glob. Anal. Geom. 54 (2018), 473–487.10.1007/s10455-018-9609-1CrossRefGoogle Scholar
Yau, S. T., Some function-theoretic properties of complete Riemannian manifold and their applications to geometry, Indiana Univ. Math. J. 25 (1976), 659–670.CrossRefGoogle Scholar
Yun, G., Total scalar curvature and $L^2$ harmonic 1-forms on a minimal hypersurface in Euclidean space, Geom. Dedicata. 89 (2002), 135–141.CrossRefGoogle Scholar