Lattice subgroups of free congruence groups

Extract

Let Г(1) denote the homogeneous modular group of 2 × 2 matrices with integral entries and determinant 1. Let 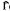(1) be the inhomogeneous modular group of 2 × 2 integral matrices of determinant 1 in which a matrix is identified with its negative. 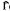(N), the principal congruence subgroup of level N, is the subgroup of 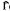(1) consisting of all T ∈ 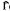(1) for which T ≡ ± I (mod N), where N is a positive integer and I is the identity matrix. A subgroup 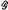of 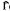(1) is said to be a congruence group of level N if 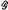contains 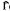(N) and N is the least such integer. Similarly, we denote by Г(N) the principal congruence subgroup of level N of Г(1), consisting of those T∈(1) for which T ≡ I (mod N), and we say that a sub group 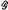of Г(1) is a congruence group of level N if 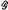contains Г (N) and N is minimal with respect to this property. In a recent paper [9] Rankin considered lattice subgroups of a free congruence subgroup 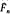of rank n of 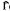(1). By a lattice subgroup of 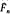we mean a subgroup of 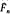which contains the commutator group . In particular, he showed that, if 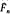is a congruence group of level N and if 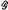is a lattice congruence subgroup of 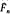of level qr, where r is the largest divisor of qr prime to N, then N divides q and r divides 12. He then posed the problem of finding an upper bound for the factor q. It is the purpose of this paper to find such an upper bound for q. We also consider bounds for the factor r.