Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-12-02T21:13:00.472Z Has data issue: false hasContentIssue false
  • English
  • Français

Group rings with finite central endomorphism dimension

Published online by Cambridge University Press:  18 May 2009

B. A. F. Wehrfritz
B. A. F. Wehrfritz
Affiliation:
Queen Mary College, London, England. E1 4NS
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

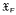Let F be any field. Denote by the class of all groups G such that every irreducible FG-module has finite dimension over F and by the class of all groups G such that every irreducible FG-module has finite dimension over its endomorphism ring. Clearly

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 24 , Issue 2 , July 1983 , pp. 169 - 176
Copyright
Copyright © Glasgow Mathematical Journal Trust 1983

References

REFERENCES

1.Hartley, B., preprint.Google Scholar
2.Musson, I. M., Representation of infinite soluble groups, preprint.Google Scholar
3.Passman, D. S., The Algebraic Structure of Group Rings (John Wiley & Sons, 1977).Google Scholar
4.Robinson, D. J. S., Finiteness Conditions and Generalized Soluble Groups (2 vols) (Springer-Verlag, 1972).Google Scholar
5.Snider, R. L., Solvable groups whose irreducible modules are finite dimensional, Comm. Algebra 10 (1982), 1477–1485.CrossRefGoogle Scholar
6.Wehrfritz, B. A. F., Infinite Linear Groups (Springer-Verlag, 1973).CrossRefGoogle Scholar
7.Wehrfritz, B. A. F., Groups whose irreducible representations have finite degree, Math. Proc. Cambridge Philos. Soc. 90 (1981), 411–421.CrossRefGoogle Scholar
8.Wehrfritz, B. A. F., Groups whose irreducible representations have finite degree II, Proc. Edinburgh Math. Soc, 25 (1982), 237–243.CrossRefGoogle Scholar
9.Wehrfritz, B. A. F., Groups whose irreducible representations have finite degree III, Math. Proc. Cambridge Philos. Soc. 91 (1982), 397–406.CrossRefGoogle Scholar